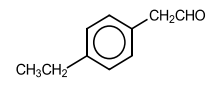
You asked to keep it simple, which is why I’m skipping explaining some details. Check the bottom of the answer to see the parts with superscript numbers explained.
The signal at $7\,\mathrm{ppm}$ is not a quartet. It would only be a (typical; there are exceptions, however not in typical organic chemistry) quartet if both of the following two conditions are met:
The signal ratio is $1\,:\,3\,:\,3\,:\,1$ and
The distance between all four signals is equal (they have the same coupling constant[1])
Both reasons stem from the way quartets are created due to coupling.
In your case, the signal is approximately $3.5\,:\,6.5\,:\,6.5\,:\,3.5$ (violating the first condition) and the distances are (enlarging the image on my screen and measuring with a ruler, so only a very rough approximation) $\mathrm{7\,mm, 12\,mm, 7\,mm}$ (violating the second condition).
What you are in fact observing is a pair of doublets. (It could be a doublet of doublets[2] as suggested by bon, but it is not.) You can think of it as two signals, each being a doublet, that are very close together. And that fits perfectly with the theory: Each signal would have an integral of $2$ and would correspond to one set of aromatic protons:
They are doublets because each one couples with one hydrogen of the other group. All that is left to explain is why one of the two signals of the doublet is higher than the other, creating a quartet-like effect. This is due to an effect called roofing or tenting. The bottom line about roofing is that it occurs if two coupling protons have close chemical shifts, and that once you have identified its existence, you can basically ignore it (it doesn’t give you any information that you couldn’t have derived otherwise).[3] You can observe the same effect in the triplet at $\mathrm{1\,ppm}$ and the quartet at $\mathrm{2.5\,ppm}$: The right-hand side signals of the quartet are slightly stronger than those on the left-hand side and vice-versa for the triplet.
[1] As you know, coupling stems from two protons being close, and connected via a chain of bonds — usually three for hydrogens. The reason that nuclei couple is because they have a spin. For hydrogen (and carbon), the spin can be ‘up’ or ‘down’. Consider two protons next to each other: Their spins can point in the same or in the opposite direction. Both cases have a slight difference in energy which gives rise to slightly different chemical shifts — the coupling you observe.
For reasons that I won’t go into detail into here, this difference does not depend on the strength of your NMR magnet (while the difference between the signals of different protons does). The ppm-scale has been chosen to remove the differences between different proton’s signals (so $2\,\mathrm{ppm}$ will always be $2\,\mathrm{ppm}$) and therefore the coupling splits will vary with the strength of the NMR magnet, which is why they aren’t given in ppm, but rather in Hz.
If you have multiple protons of the same type, the coupling value (one says: coupling constant) will always be the same, so $7\,\mathrm{Hz}$ three times in the event of a true quartet. The reason why the signals then need to be $1\,:\,3\,:\,3\,:\,1$ lies within Pascal’s triangle: Starting from a central signal split it into two halfes with a certain distance from the centre: You will get two $1\,:\,1$ signals. Do the same thing with each signal and the same distance: You will get a $1\,:\,2\,:\,1$ signal, because the two central ones will overlap. Do the same again: Voilà, $1\,:\,3\,:\,3\,:\,1$. So all true quartets that stem from this will obey the $1\,:\,3\,:\,3\,:\,1$ rule.
[2] If you have two different protons on a neighbouring atom instead of two identical ones, you will receive a doublet of doublets instead of a triplet. Think of it this way: One proton has a coupling constant of (say) $\mathrm{5\,Hz}$ with the proton of interest, and would give a doublet. The second one has a coupling constant of (say) $\mathrm{7\,Hz}$ with the same proton, and by itself would give a doublet again. Together, these two doublets would not meet in the middle as in the above $1\,:\,2\,:\,1$ case, because they have different distances. Instead, you will see a $1\,:\,1\,:\,1\,:\,1$ set of four signals, where the distance between the first and the second corresponds to $\mathrm{5\,Hz}$ while the distance between the first and the third corresponds to $\mathrm{7\,Hz}$.
But this doesn’t work here, because we are only coupling to one proton (and I would estimate a coupling constant of $\mathrm{19\,Hz}$ for the second coupling which is impossible in the structure given, and isn’t seen anywhere else in the spectra. Estimate was based on the aromatic coupling being $\mathrm{7\,Hz}$.)
[3] Two spins pointing in the same direction are different in energy from two pointing in different directions I just pointed out. However, if the chemical shifts of both protons that couple are similar, the difference is also noticeable without an external magnet, so that even without the sample being in the NMR magnet the two energy levels would differ — hence the protons are slightly more aligned per se than even distribution would suggest. This in turn means that one of the sides of the signal (corresponding to an energy difference) shows stronger absorption than the other, and because it is always the inner one, one can assume to see a roof — hence roofing.
The only information that roofing can give you — that two protons are close by in the molecule and their shifts are similar — can be derived from other information in the spectrum as well (information that corresponds to numbers! Real, mathematically physical hard numbers! Hence preferred by scientists): The proximity by the coupling constants and 2D-COSY and the similarity of the shifts … well, science doesn’t care too much.