Explain on the basis of valence bond theory that tetracyanonickelate(II) ion with square planar structure is diamagnetic

Question

Answers on the internet suggest that tetracyanonickelate ($\ce{([Ni(CN)4]^{2-}}$) with square planar structure is diamagnetic because $\ce{CN-}$ is a strong field ligand and causes pairing, but my book says that VBT does not distinguish between strong and weak field ligands. So can anyone explain the logic using VBT?

Answer 1

@M.L has already provided the solution as per VBT, but that answer seems to have some flaws when it comes to CFT (looks like he has corrected the mistakes now). So, I would like to take some time to answer this question using CFT.

---

According to crystal field theory, the degeneracy of the orbitals of a same orbital is lost in presence of a ligand and the pattern in which they are distorted depends upon the nature of the ligand. The spectrochemical series helps us understand the nature of a ligand.

In general there are two types of ligands, strong field and weak field. Strong field ligands have a greater power to distort the degeneracy of the sub-orbitals whereas weak field have a weaker power of distortion.

As per the spectrochemical series, $\ce{CN-}$ is a strong field ligand and thus creates a greater distortion in the degeneracy of $\mathrm d$ orbitals.

The central metal ion here is the $\ce{Ni^2+}$ with its $\mathrm d^8$ electronic configuration.

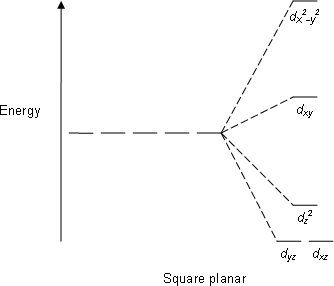
A $\mathrm d^8$ system prefers a square planar approach of ligands if the ligands are strong field. In such a condition the the degeneracy is disrupted and the energy diagram of the $\mathrm d$ orbitals is as below:

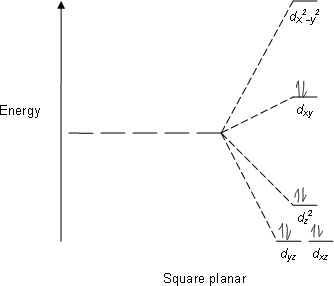
Now when trying to fill this diagram with electrons we have to take into consideration that an electron in the $\mathrm d_{x^2-y^2}$ orbital can pair up with the electron in $\mathrm d_{xy}$ in case of a strong field ligand. This is because the extent of splitting done by a strong field ligand is so high that it can overcome the energy gained due to pairing of electrons.

Thus the energy diagram filled with electrons looks as following:

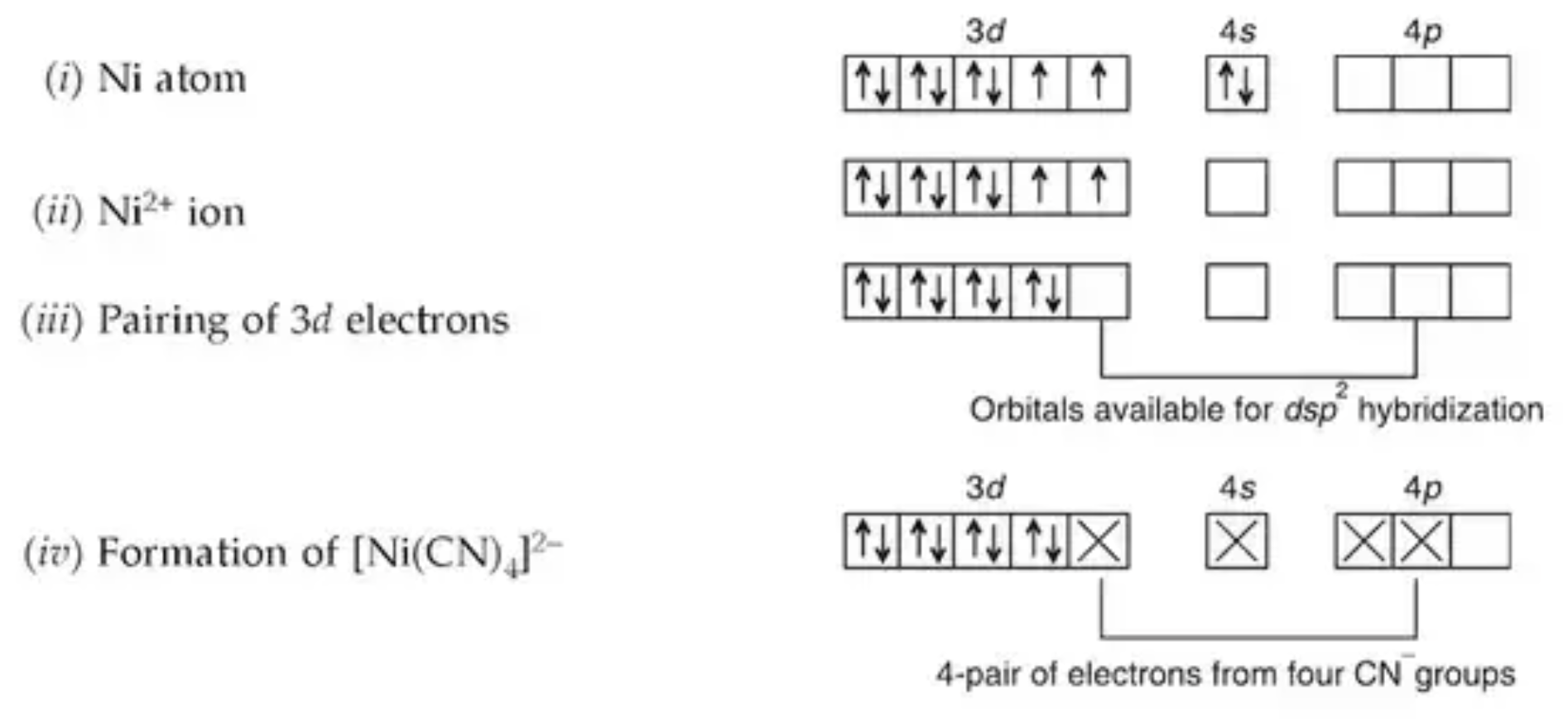
Now the ligands donate their lone pairs in the $3\mathrm d_{x^2-y^2}$, $4\mathrm s$, $4\mathrm p_x$ and $4\mathrm p_y$ sub-orbitals.

In reality, no hybridization takes place but if forced to say the hybridization of this complex, we can say $\mathrm{dsp}^2$ because of the orbitals involved in the $\sigma$ bonds between the metal and ligands.

As you can see the energy diagram does not contain any unpaired electron and thus the complex is diamagnetic.

Answer 2

According to VBT, for $\ce{[Ni(CN)_4]^{2-}}$, because it is a square planar geometry, it will experience $\ce{dsp^2}$ hybridization. The following process and result will be the following:

The diagram clearly shows that using VBT, we get that $\ce{[Ni(CN)_4]^{2-}}$ is diamagnetic. However, in general, VBT is not used often to describe these bonds, and more and more complex theories of bonding have been developed, although all involve approximations/assumptions. One of them is crystal field theory which is what the internet answers were suggesting.

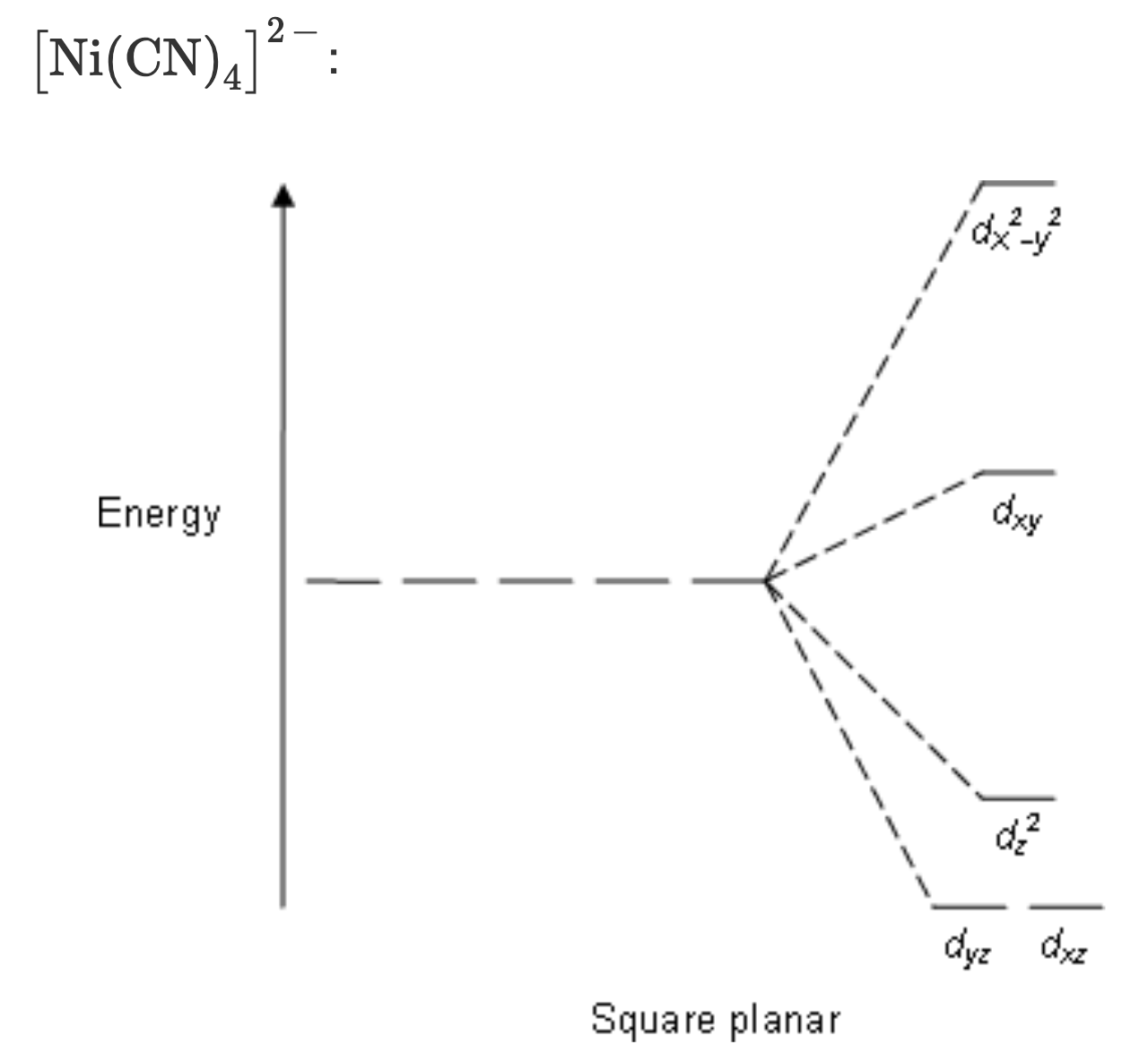
In terms of crystal field theory. $\ce{Ni^{2+}}$ has a $\ce{d^8}$ configuration, and the crystal field splitting diagram is square planar. Since $\ce{CN-}$ creates large splitting, only four orbitals will be filled in the following diagram:

We will find that only $\ce{d_{yz}}$, $\ce{d_{xz}}$, $\ce{d_{z^2}}$, and $\ce{d_{xy}}$ will be filled as $\ce{d_{x^2-y^2}}$ is too high in energy. Since there are 8 electrons, all of the 4 orbitals will be fully filled and this makes it diagmagnetic according to crystal field theory.