In density functional theory (DFT) there is classic Coulomb energy and non-classic correlation energy. But what is difference between them? In books these energies are explained very similarly. Both contributions are explained by the fact that electrons are negatively charged particles.
-
$\begingroup$ I would say its not the correlation energy which is nonclassical, since it is just a consequence of the motion of the electrons being correlated. What is nonclassical is the exchange energy (which is often lumped together with the correlation in DFT). The exchange energy is nonclassical because it is Coulomb like, but only arises due to the fact that electrons are fermions (i.e. that their wavefunction is antisymmetric with respect to exchange of particle labels). @Stanislav $\endgroup$– Tyberius ♦Commented Jan 16, 2019 at 16:21
-
$\begingroup$ But hence why we cannot consider that correlated motion via only Coulomb interaction? Coulomb repulsion doesn't really include that correlated motion? $\endgroup$– StanislavCommented Jan 16, 2019 at 16:30
-
$\begingroup$ The correlation is due to e-e repulsion. However the Coulomb energy is generally used to refer to the energy based on the average effect of the electrons repelling each other. $\endgroup$– Tyberius ♦Commented Jan 16, 2019 at 16:42
1 Answer
All mentioned terms -- classical Coulomb, exchange and correlation energy -- have the very same physical origin: the electron-electron Coulomb repulsion.
The split into these contributions is only an artifact arising from Hartree-Fock theory. Here the electronic wave function is approximated by a Slater determinant (the anti-symmetrized product of orbitals). This results in a mean-field description of the electron-electron interaction, and the error with respect to the exact solution is the correlation energy.
Plugging the Slater determinant into the Schrödinger equation eventually leads to an expression for the Hartree-Fock energy:
\begin{equation} E_\mathrm{HF} = \sum_i^{N_\mathrm{elec}} \langle i|\hat h|i\rangle + \sum_{i>j}^{N_\mathrm{elec}} [ii|jj] - [ij|ji] \end{equation}
The three terms in that equation use a short hand notation. Explicitly written those are the one-electron integrals \begin{equation} \langle i|\hat h|i\rangle = \int\chi_i^*(\boldsymbol x_1)\hat h\chi_i(\boldsymbol x_1) \mathrm{d}\boldsymbol x_1 \end{equation} which cover the kinetic energy and electron-nuclear Coulomb interaction.
The other two terms originate from the electron-electron interaction. The first one is called Coulomb integral, and gives the contribution of the classical Coulomb energy \begin{equation} [ii|jj] = \int\chi_i^*(\boldsymbol x_1)\chi_i(\boldsymbol x_1) \frac{1}{r_\mathrm{12}}\chi_j^*(\boldsymbol x_2)\chi_j(\boldsymbol x_2) \mathrm{d}\boldsymbol{x_1}\mathrm{d}\boldsymbol{x_2} \end{equation} The only reason for its name is, because with $\chi_i^*(\boldsymbol x_1)\chi_i(\boldsymbol x_1) = \rho_i(\boldsymbol x_1)$ and $\chi_j^*(\boldsymbol x_2)\chi_j(\boldsymbol x_2) = \rho_j(\boldsymbol x_2)$ one obtains the formula for the coulomb interaction of two classical charge distributions $\rho_i(\boldsymbol x_1)$ and $\rho_j(\boldsymbol x_2)$. However, we are doing quantum mechanics here, so do not over interpret that analogy.
The last term is the exchange integrals \begin{equation} [ij|ji] = \int\chi_i^*(\boldsymbol x_1)\chi_j(\boldsymbol x_1) \frac{1}{r_\mathrm{12}}\chi_j^*(\boldsymbol x_2)\chi_i(\boldsymbol x_2) \mathrm{d}\boldsymbol{x_1}\mathrm{d}\boldsymbol{x_2} \end{equation} and simply named like that because it is essentially the Coulomb term but two indices have been exchanged.
In DFT these three terms show up, because it borrows some concepts from HF and but tries to add the missing electron correlation.
Interpretation of Electron Correlation
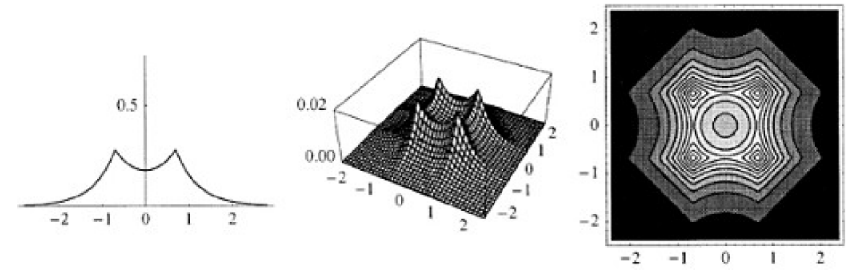
I always struggle with the "correlated motion" thing to explain what electron correlation is. I don't think it helps anyone to get an intuitive understand what this means. I do like however the following pictures from the book by Helgaker, Jorgensen and Olsen.
They consider the electron density in $\ce{H2}$ in HF (mean-field) approximation in the top plots and the exact solution below. To the left we have the 1-electron density which is obtained by integration (=averaging) of the 2-electron density the in middle and right plots. The positions of the two Hydrogen atoms are obvious by the peaks in the 1-electron density.
The 2-electron density of the HF solution has 4 peaks: The 2 at ($r_1\approx 1$, $r_2\approx -1$) and ($r_1\approx 1$, $r_2\approx -1$) are the probability of finding both electron at different atoms. The other 2 at ($r_1\approx 1$, $r_2\approx 1$) and ($r_1\approx -1$, $r_2\approx -1$) are the probability of finding both electrons at the same atom. The latter 2 peaks are missing in the exact solution, because the electrons try to avoid such situations. HF however is unable to consider this correlation. Note that this effect is not visible in the 1-electron densities. HF only works based the 1-electron wave functions (orbitals) and constructs the 2-electron wave function by simple multiplication the 1-electron wave functions.