Determining R-S configuration with lowest priority groups in plane of paper

Question

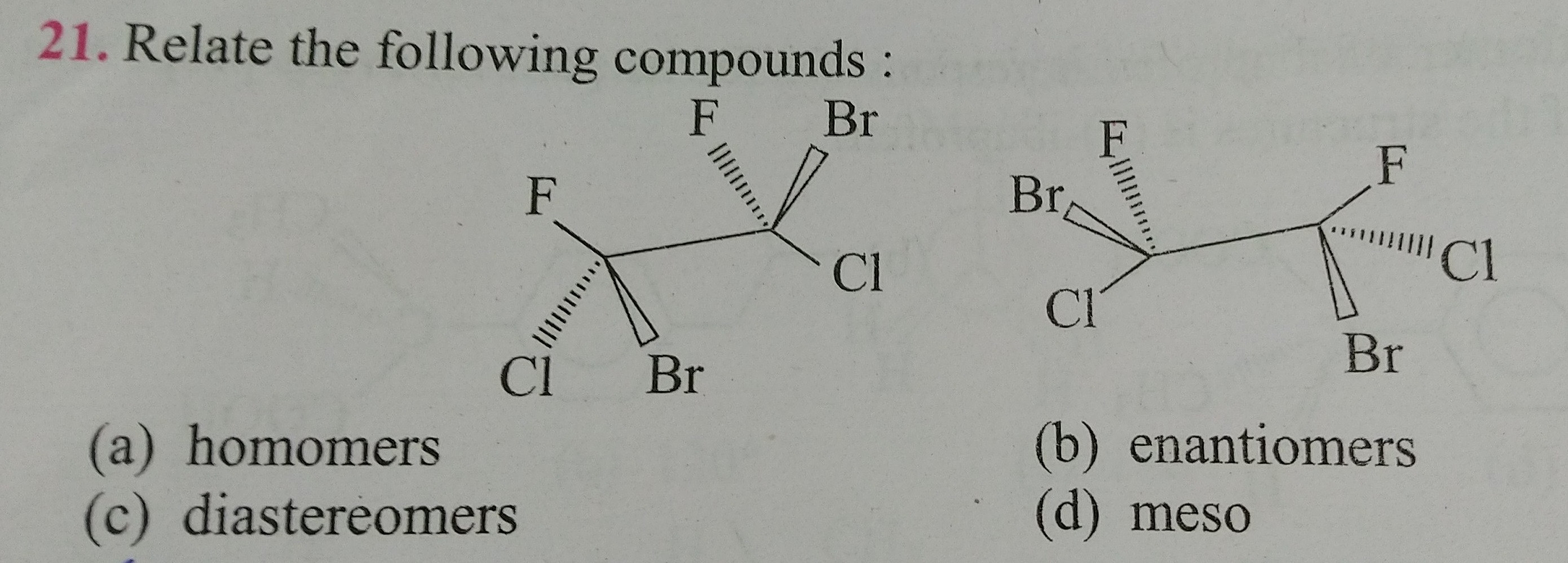
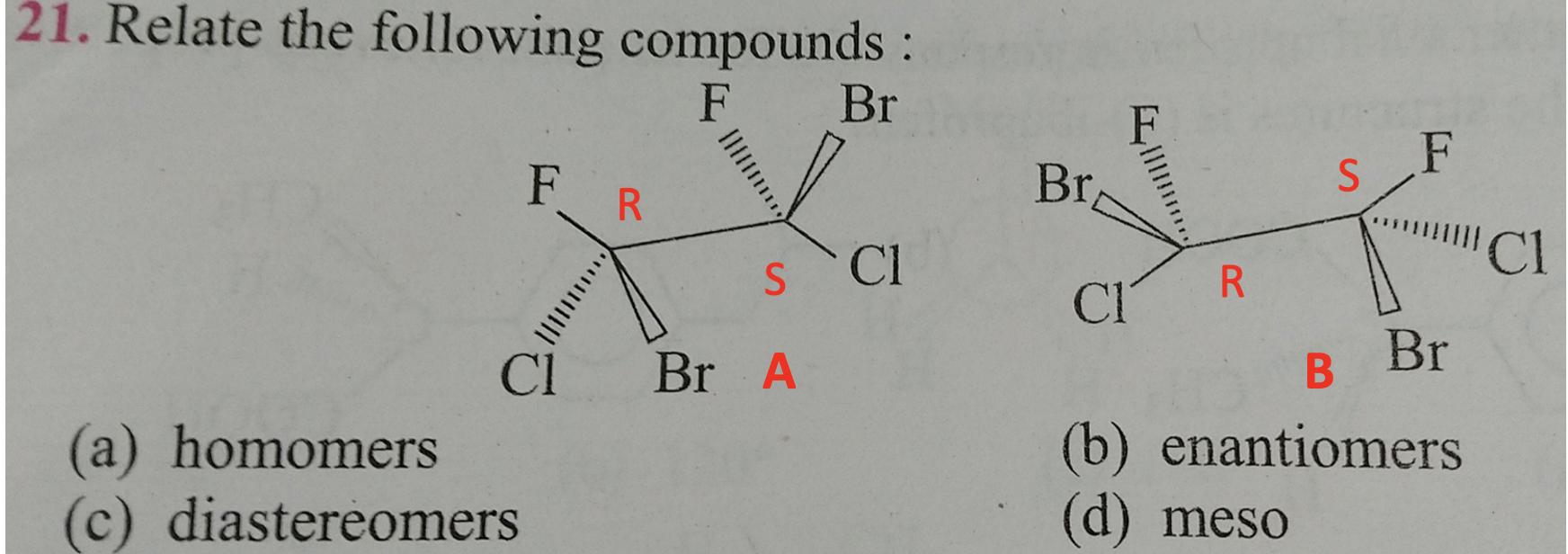
I was studying R-S nomenclatures and determining configurations of molecules when I came across a certain problem, which had the 4th priority group on the plan of paper (not on a dash or wedge)

According to this: https://www.masterorganicchemistry.com/2016/10/20/introduction-to-assigning-r-and-s-the-cahn-ingold-prelog-rules/#five

All I need to do to determine the R-S config in these cases is to switch the group in the 4th place with the one in the back, thus "flipping its configuration", then determine the R-S for that molecule and the actual result is the opposite. I know why this works and it has worked for me in the previous problems that I have attempted. However, in this question:

For the left compound (looking at the carbon on the bottom), clearly, $\ce{Br}$ has priority $1$, $\ce{Cl}$ is $2$, $\ce{F}$ is $3$ and the other carbon attached to it is clearly $4$ in priority which is in the plane of paper. Swapping the groups in priority $4$ with that in the back i.e., $\ce{Cl}$, and going from $1,2,3$, I get S as the absolute configuration of the flipped molecule, so the actual configuration should be R. But the answer does say that S is indeed the configuration of both the carbons in the 1st compound and R is the configuration for both carbons on the right molecule.

Why does the swapping of the groups in back and lowest priority not work here?

Answer 1

Techie5879: If you have followed my suggestion in the Comment above, you probably have answered this question yourself. Now that you no longer "flip" structures, you should have the R/S assignments for A and B (vide infra). Because each carbon in A and B bears F, Cl and Br and both A and B have R and S configurations, they are identical meso compounds. I was not familiar with the term "homomer", so I looked it up.

Homomers : Homomers are the identical representations of the same compound. It means that when the same compound is represented in different ways, then all the representations are known as homomers of each other. These representations are superimposable on each other. (Italics added.)

If you pickup A (mentally) and superimpose the S-carbons onto B, pairing halogens (F with F, etc.), then the R-carbons will superimpose halogens but they will not match halogen for halogen. In this context they are not homomers. That is to say, they are different conformations of the same compound. Because A and B are identical compounds, they cannot be enantiomers or diastereomers.

I would appreciate any constructive feedback as to whether or not my interpretation of "homomer" is correct or whether or not the term serves a meaningful purpose. Exclude any applications to polymers or biochemistry.

ADDENDUM: Per the OP's request, the Fischer projections of A, aka B, are provided here.

Answer 2

One way to assess the configuration without having yo swap or ritate functional groups us to use the concept of even and odd permutations.

Say you have ranked the substituents on a stereocenter and a group other than Group 4 is oriented to the bottom. Proceed as follows:

1. List the rankings of the three groups directed to you in clockwise order.

2. Attach the bottom group at the end of the list.

3. Count the pairwise interchages that you would need to get your permutation to the "standard" 1234. An even count, called an even permutation, corresponds to R and an odd permutarion corresponds to S.

Let us look at the left carbon in molecule A. Bromine ranks 1, chlorine 2, fluorine 3 and the other half of the molecule, attached through carbon, ranks 4. So 2 is directed to the bottom and the other groups in clockwise order are 134. Thus you render your permutation as 1342.

To get from 1342 to 1234 you then need to interchage the 2 with the 4 and then with the 3; if you try to interchage 2 directly with 3 first you have 3 and 4 in the wrong order. Thus you need two interchanges, an even number, and so the left carbon is configured R.

Can you work out the other centers with this method?