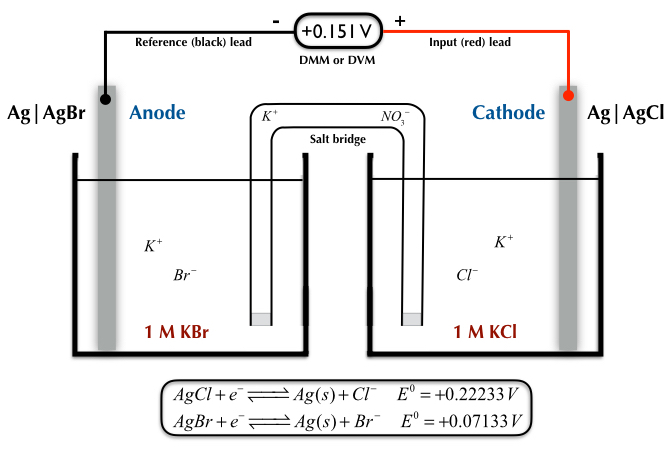
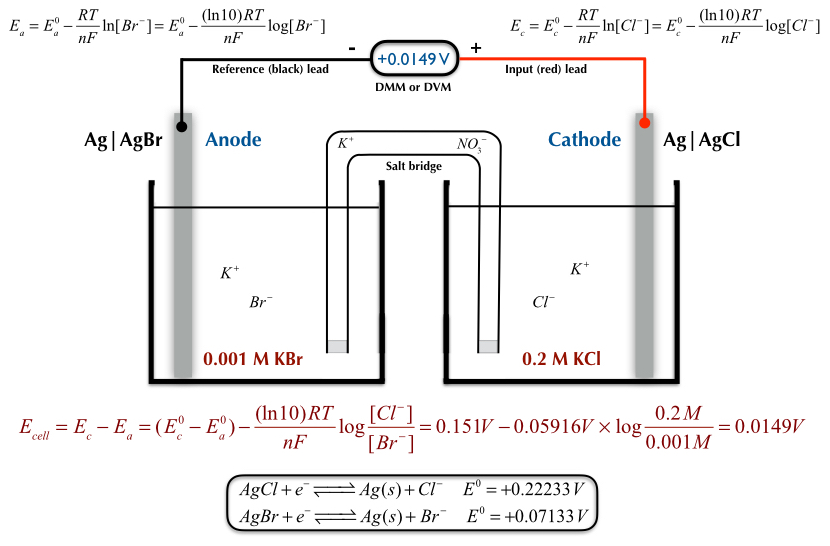
How to start solving the problem since the $E^\circ$ of individual half reactions is not given?
This is a concentration cell, i.e. the half reactions at the anode and at the cathode are the same (except for the direction).
AgCl(s) electrode
$$\ce{AgCl(s) <=> Ag+(aq) + Cl-(aq)}$$
$$\ce{Ag+(aq) + e- -> Ag(s)} $$
AgBr(s) electrode
$$\ce{Ag(s) -> Ag+(aq) + e-}$$
$$\ce{Ag+(aq) + Br-(aq) <=> AgBr(s)}$$
The standard reduction potentials will cancel out, i.e. $E^\circ (\mathrm{cell}) = 0$.
Further thoughts
[Comment by EdV] The Ag|AgCl electrodes I have are commercial, but making them in the lab is just a matter of oxidizing Ag wire in a chloride solution, so the electrode is Ag wire with an adherent coating of AgCl... I have never seen one of these made by just sticking an Ag wire in the Ag halide, but I guess it would work.
I added more to the answer prompted by that thoughtful comment.
[...my own comment] I pictured the silver electrode submerged in the solution, with the solid halide on the bottom. I am puzzled now too. Does it make a difference if the electrode touches the solid, the liquid, or both?
What are the actual reduction potentials?
$$\ce{AgCl(s) + e− <=> Ag(s) + Cl−}\ \ \ \ E^\circ_\mathrm{red} = \pu{+0.22233 V}\tag{1}$$
$$\ce{AgBr(s) + e− <=> Ag(s) + Br−}\ \ \ \ E^\circ_\mathrm{red} = \pu{ +0.07133 V}\tag{2}$$
$$\ce{Ag+ + e− <=> Ag(s)}\ \ \ \ E^\circ_\mathrm{red} = \pu{ +0.7996 V}\tag{3}$$
Are they related?
If you subtract (3) from (1), you get the dissolution reaction of AgCl, if you subtract (3) from (2), you get the dissolution reaction of AgBr. Thus, standard reduction potentials for (1) and (3) should be different by
$$ -\frac{RT}{zF} \ln K_\mathrm{sp}(\ce{AgCl})$$
and standard reduction potentials of (2) and (3) should be different by
$$ -\frac{RT}{zF} \ln K_\mathrm{sp}(\ce{AgBr})$$
Finally, standard reduction potentials (1) and (2) should be different by
$$ -\frac{RT}{zF} \ln \frac{K_\mathrm{sp}(\ce{AgBr})}{K_\mathrm{sp}(\ce{AgCl})}$$
Numerical answer using half reaction (1) and (2)
$$\ce{AgCl(s) + Br-(aq) <=> AgBr(s) + Cl-(aq)}$$
$$Q = \frac{[\ce{Cl-}]}{[\ce{Br-}]} = 200$$
$$E_\mathrm{cell} = E^\circ_\mathrm{cell} - \frac{R T}{z F} \ln(Q)$$
$$= \pu{(0.22233 V− 0.07133 V) - 0.13612 V = 0.0149 V}$$
Numerical answer using half reaction (3) twice
$$\ce{Ag+(c) + Ag(b) <=> Ag(c) + Ag+(b)}$$
"c" stands for chloride side, and "b" stands for bromide side. For consistency, I am using the following values for the solubility products (derived from difference of standard reduction potentials of half reactions (1), (2) and (3)).
$$K_\mathrm{sp}(\ce{AgCl}) = \pu{1.74e−10}$$
$$K_\mathrm{sp}(\ce{AgBr}) = \pu{4.89e−13}$$
$$[\ce{Ag+}]_c = K_\mathrm{sp}(\ce{AgCl}) / [\ce{Cl-}] = \pu{8,27e−10} $$
$$[\ce{Ag+}]_b = K_\mathrm{sp}(\ce{AgBr}) / [\ce{Br-}] = \pu{4.89e−10} $$
$$ Q = \frac{[\ce{Ag+}]_c}{[\ce{Ag+}]_b} = 0.560 $$
$$E_\mathrm{cell} = E^\circ_\mathrm{cell} - \frac{R T}{z F} \ln(Q)$$
$$\pu{= 0 - (-0.0149 V) = 0.0149 V}$$