8
$\begingroup$

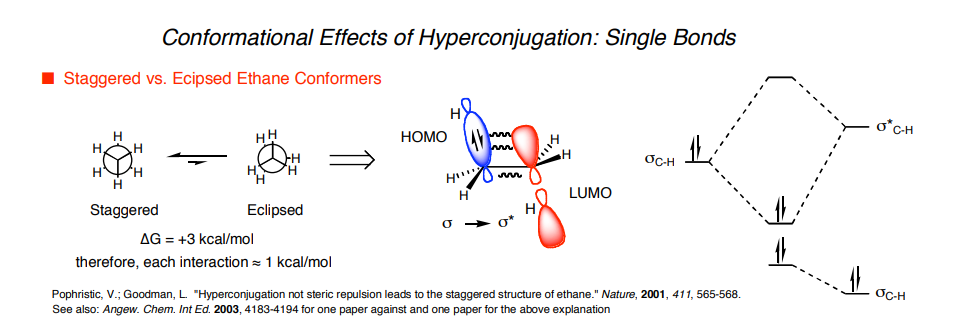
I refer you to this set of presentation slides. On slide 8, the author explains the preference for the staggered conformation of ethane by saying that there is a favourable hyperconjugative interaction between $\sigma^{}_{\ce{C-H}}$ of the $\ce{C-H}$ bond in one methyl group and $\sigma^{*}_{\ce{C-H}}$ of another $\ce{C-H}$ bond in the other methyl group.

However, I am puzzled by the diagram. Since both $\ce{C-H}$ bonds are similar in terms of bonding, their bonding and antibonding $\sigma$ molecular orbitals should have the same energies. Instead, the diagram shows that one of the $\sigma_{\ce{C-H}}$ bonds is lower than the other in energy. How can this be right? I have shown a screenshot of the diagram below.

enter image description here

Also, how can a $\sigma_{\ce{C-H}}$ donate electron density to a $\sigma^*_{\ce{C-H}}$ effectively? After all, the energy difference between them is rather significant since the orbital interaction between the atomic orbitals of $\ce{C}$ and $\ce{H}$ is large.

Improve this question
$\endgroup$
0

2 Answers 2

Reset to default
5
$\begingroup$

I am not entirely sure what purpose the lower sigma bonding orbital in the MO-diagram serves, because an ethane molecular orbital would, as you have surmised correctly, have six degenerate $\sigma_{\ce{C-H}}$ MOs and a $\sigma_{\ce{C-C}}$ MO, lower in energy.

My best guess would be the position of the $\sigma_{\ce{C-H}}$ on the right hand is quite arbitrary and the basic purpose of the diagram is to show the possible overlap between the $\sigma_{\ce{C-H}}$ of one $\ce{C-H}$ bond and the $\sigma^{*}_{\ce{C-H}}$ of $\ce{C-H}$ bond anti to it - you don't really need to be worried about the $\sigma_{\ce{C-H}}$ of that anti bond because it cannot overlap with the other $\sigma^{}_{\ce{C-H}}$.

That brings us to the second part of your query. A $\sigma_{\ce{C-H}}$ can donate electron density to an empty $\sigma^{*}_{\ce{C-H}}$ anti to it, because the latter is oriented correctly (anti) to accept the electron density from it. It's a question of orbital symmetry and not energy difference.

Improve this answer
$\endgroup$
4
  • $\begingroup$ You are right. But if the energy difference is large, interaction between the orbitals of differing energies would be very small. In this case, this should be the case. $\endgroup$
    – Tan Yong Boon
    Commented Mar 18, 2018 at 8:09
  • 2
    $\begingroup$ You're talking about energies of the order of ~1 kcal/mol. You think that's large as compared to the bond enthalpies of the various C-C or C-H bonds? $\endgroup$
    – Sagnik
    Commented Mar 18, 2018 at 15:54
  • 2
    $\begingroup$ I agree that I don't know what the third MO in the diagram is meant to be; the cited Nature paper doesn't include it either and it could just have been something that was elaborated on during the presentation itself. [I didn't check the Angew paper.] I also agree that the hyperconjugative stabilisation is tiny compared to some other similar things we see (e.g. anomeric effect, (Z)- vs (E)-ester conformation preference). I don't agree, though, that the σ(CH) cannot overlap with another σ(CH). The overlap seems to me to be very poor, but perfectly symmetry allowed. $\endgroup$
    – orthocresol
    Commented Mar 21, 2018 at 2:08
  • 2
    $\begingroup$ One should be very careful assigning energies to hybrid orbitals, as these are not the eigenfunctions of the Hamiltonian. (Hybrid orbitals do at the very least break wave function symmetry.) As such the image is just a very tempting illustration of that concept. $\endgroup$
    – Martin - マーチン ♦
    Commented Mar 21, 2018 at 3:59
-1
$\begingroup$

Just to build on the very good answer from Sagnik, the purpose of the σC-H bond on the right side of the diagram is to show that the C-H bond is destabilized by electron density in its antibonding orbital. But the energy of the overall system is reduced.

The red orbital structure isn't in the Angew. paper either.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.