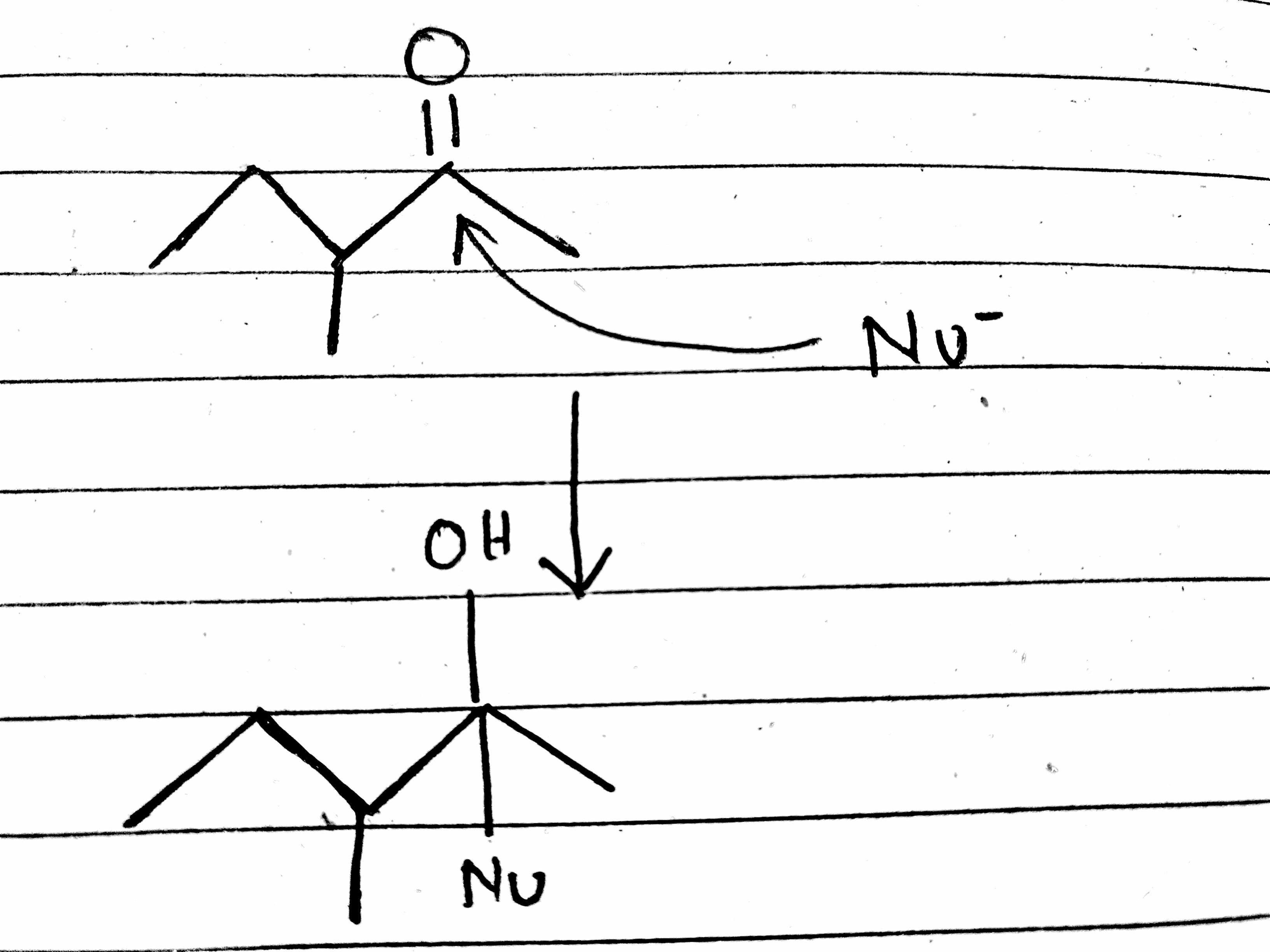
When you add to the planar carbonyl group, attack from both sides of the plane is equally probable. If there are no other carbons bearing four different groups in the molecule (chiral centres), a pair of enantiomers will be formed in equal amounts (racemate). Keep in mind that diastereomers and enantiomers apply to relationships between pairs of molecules. If you create two pairs of enantiomers, then the molecules in each pair will be diastereomers of the other pair. So you also create two pairs of diastereomers.
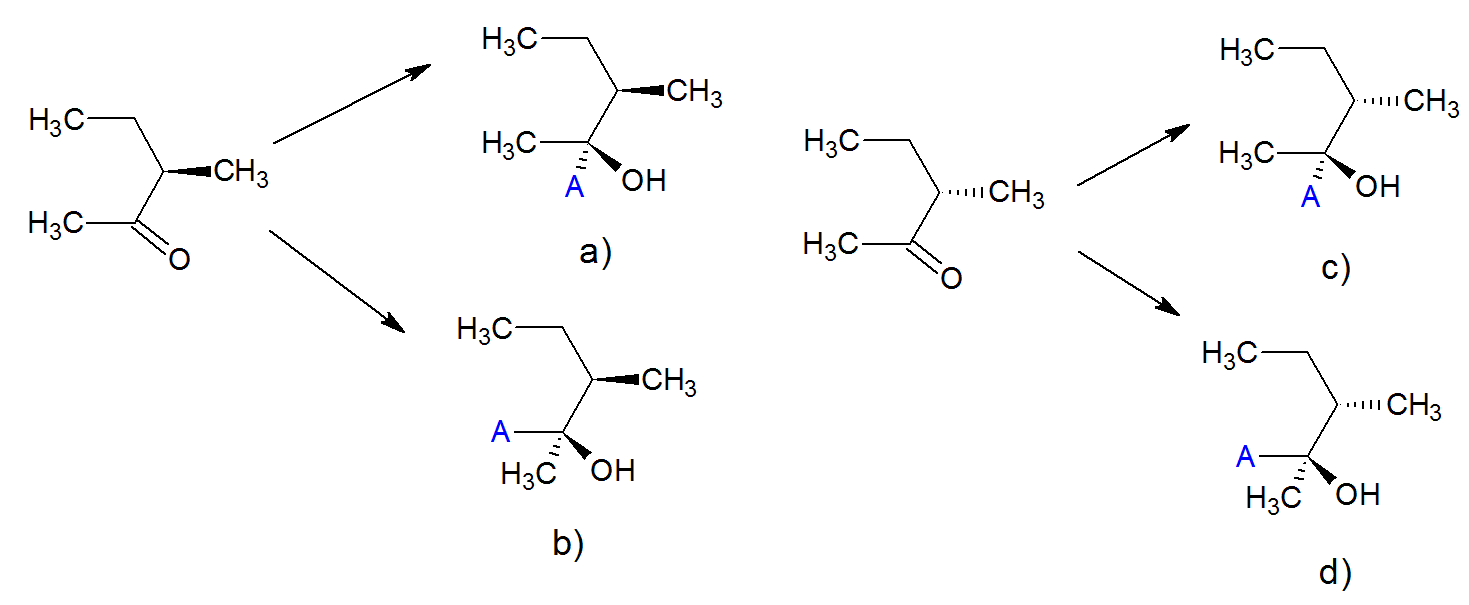
Now, there is already a chiral centre in your molecule - but its configuration is not given. Without knowing that, the question cannot be answered properly. The molecules could be either all R or all S; assuming it is R addition of the nucleophile will give (R,S) and (R,R) in equal amounts. These are diastereomers because the mirror image of (R,S) is (S,R) and the mirror image of (R,R) is (S,S). The same applies if all your molecules would have an S chiral centre before addition. The molecules that are formed are not mirror images.
If your molecule would be present as 50% R and 50% S beforehand (racemate), addition will create equal amounts of (R,R), (R,S), (S,R) and (S,S). Now, you have two pairs of enantiomers: (R,R)/(S,S) and (R,S)/(S,R). Every stereoisomer in the mixture has one mirror image (together they are enantiomers) and 2 others that are diastereomers. I'd also like to add that enantiomers are definitely not diastereomers. In fact, that's how IUPAC defines diastereomers: they are stereoisomers that are not enantiomers. This mixture is not a racemate because there are four stereoisomers. According to IUPAC, a racemate is a pair of enantiomers present in equal amounts.
If the nucleophile is hydroxide, then addition will not create an additional chiral centre. So then it would depend on whether you had a single stereoisomer to start with or a mixture, whatever stereochemistry you started with will be retained since no bonds to the chiral centre are formed or broken.