When you have charged particles in a liquid acted upon by a electric field, the experience a force, and said force accelerates them to a terminal, drift speed.
Note: The physical treatment of the problem, and the notion of terminal speed is quite similar to say a ball falling from the top of a sky scraper through air (a fluid medium)
Now, let's go back to our charged particles in fluids. Let's say we have applied a voltage $\Delta\phi$ between two large, planar electrodes, immersed in the liquid and separated by a distance $l$
The magnitude of the electric field, and the electric force experienced by an ion of charge $ze$ are given by the following relations:
$$ E = \frac{\Delta\phi}{l}$$
$$F = zeE = (ze).\frac{\Delta\phi}{l}$$
A cation responds by accelerating towards the negative electrode, and an anion responds by accelerating towards the positive electrode. This acceleration, however, is short lived because the fluid medium offers a retarding force $F_{\text{friction}}$.
$$F_{\text{friction}}\propto s\text{, where s := speed} $$
Assuming we have spherical particles of radius $a$, let's say our retarding force is given by Stokes' Law
$$F_{\text{friction}} = (6\pi\eta a)s$$ $\eta$) is the viscosity of the medium.
When the electric force is exactly balaced by the viscous drag, the acceleration drops to zero and this final speed of the particle is called the drift speed ($s_d$).
$$F_{\text{friction}} = F_{\text{electric}}$$ and solving for the drift speed we get,
$$s_d = \frac{zeE}{6\pi\eta a}$$
At this point we note that the drift speed is proportional to the applied electric field and introduce the following definition:
$$s_d = \mu E$$ where, $$\mu = \frac{ze}{6\pi\eta a} $$
and we call $\mu$ the mobility.
From the aforementioned equations/definitions one can deduce that $\mu \propto \frac{1}{a}$. Thus, bigger/bulky ions ought to have lower mobilities, and lower drift speeds in solution.
However, $a$ is not the ionic radius, but the Stokes radius (or the hydrodyanmic radius), i.e the "effective radius" of an ion in solution taking into account its solvation shell (i.e the solvent molecules surrounding the ion). I believe the following illustration is instructive, 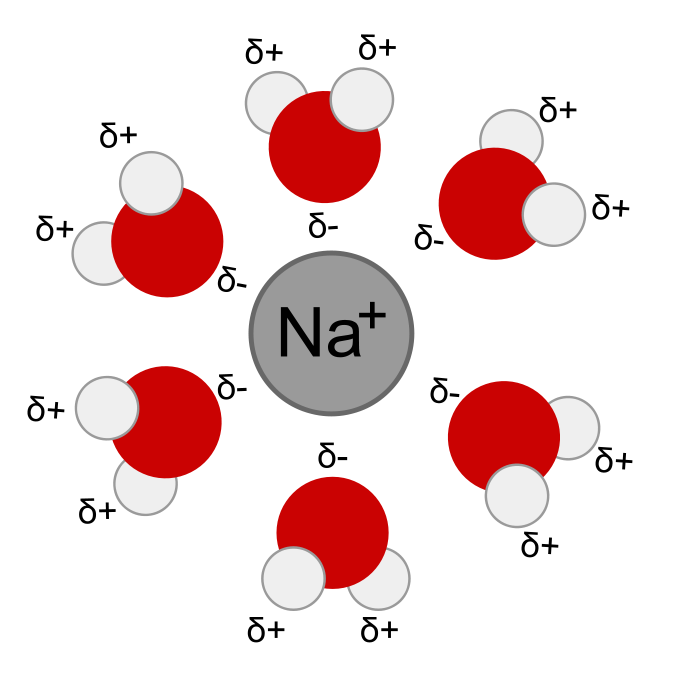
The size of this solvation shell depends on how "attractive" an ion appears to a solvent molecule, and that in turn depends on the electric field produced by the ions.
For spherical ions, we consider the electric field on the surface of a charged sphere: $$ E _\text{surface of a charged sphere} \propto \frac{ze}{r^2} $$
here $r$ is the ionic radius. So smaller ions have a larger solvation shell than larger ions (assuming they carry the same charge).
This is the reason why mobilities of alkali metal ions increase from $\ce{Li^+}$ to $\ce{Cs^+}$ (and not the other way around); the lithium ions are effectively dragging a large amount of solvent molecules with them and thus, move slower.

