The oxidation state of platinum in $\ce{[PtCl2(NH3)2]}$ complex is $+2,$ so its configuration will be $\ce{5d^8 6s^0 6p^0}$ and the shape will be square planar.
But this complex has two strong field ligands $(\ce{NH3})$ and two weak field ligands $(\ce{Cl-}).$ So, will the pairing energy be higher, or the CFSE will be higher because CFSE depends on the ligands capacity?
In this particular case, while CFT is surely useful to do a preliminary analysis, I think that one should resort to Ligand Field Theory and use the proper combinations of molecular orbitals to investigate the properties of this complex. Consider that for a $C_{2v}$ point group (such as $\ce{cis-PtCl2(NH3)2}$) you should resort to group theory in order to build a reliable energy level diagram.
If you already know the symmetry labels for the metal, assuming a square planar structure, you get a diagram of general form:
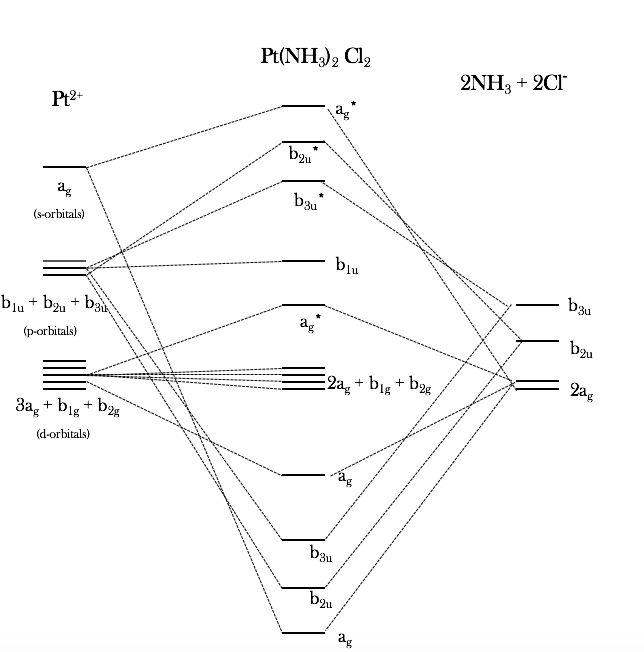
On the left, the original metal orbitals are labeled with their irreducible representations, while on the right, the SALCs (Symmetry Adapted Linear Combinations) of the molecular orbitals for each ligand are displayed. Constructing the diagram at the center of this image, though neither simple nor quick to do manually, is feasible with access to character tables for any point group.
The correct ordering of energy levels in the complex results directly from evaluating overlap, Coulomb, and exchange integrals (using the Hartree-Fock approximation, that is not perfect but gives a reasonable estimation). Even without explicit energy values, we can predict that some energy levels of the metallic ion will not combine with the SALCs. This occurs because certain metallic atomic orbitals do not pair with any possible SALCs, remaining as non-bonding molecular orbitals with energy eigenvalues essentially unchanged from the original atomic orbitals.
To evaluate the ligand field stabilization energy, fill the molecular orbitals according to the Aufbau principle and then calculate the stabilization energy explicitly.
Reference for the image: https://www.chegg.com/homework-help/questions-and-answers/molecular-orbital-diagram-pt-nh3-2cl2-cisplatin-q26485164
