Why is disilyne $\ce{Si2H2}$ bent when the steric number of silicon is 2?
$\begingroup$
$\endgroup$
9
-
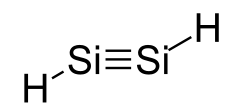
5$\begingroup$ Short answer is: because VSEPR theory is oversimplified model of reality. $\endgroup$– WildcatCommented Sep 21, 2014 at 10:25
-
1$\begingroup$ "The equilibrium geometry of disilyne is not linear, but is twisted. The potential surfaces of acetylene and disilyne have a critical internuclear distance between the central atoms, where the stable geometry changes from linear to twisted forms. The R-dependence of the valence-shell electron energy causes the difference in the structure of the molecules." dx.doi.org/10.1016/0009-2614(82)87044-9 $\endgroup$– WildcatCommented Sep 21, 2014 at 10:30
-
$\begingroup$ Could you elaborate that in an answer? Why is that particular form more abundant than linear? $\endgroup$– EJCCommented Sep 21, 2014 at 10:32
-
$\begingroup$ So electronic structure calculations cited above predict twisted equilibrium geometry for $\ce{H2Si2}$ with the only issue that the calculations are rather crude (HF/STO-3G). :D $\endgroup$– WildcatCommented Sep 21, 2014 at 10:33
-
1$\begingroup$ The twisted form of $\ce{H2Si2}$ is more energetically stable, and thus, it is more likely to find $\ce{H2Si2}$ in this form. The question why the twisted form is more stable does not have an answer you are looking for, except for that this is just the way nature works. :D It can be explained in terms of electronic structure theory as in the paper linked in one of my previous comments. $\endgroup$– WildcatCommented Sep 21, 2014 at 10:38
|
Show 4 more comments
1 Answer
$\begingroup$
$\endgroup$
2
The following disilyne has been prepared and found to be stable to ~100 C. An X-ray crystal structure found that the two silicon atoms in the triple bond, Si(1) and Si(1'), along with the two attached silicon atoms, Si(2) and Si(2') are coplanar and the Si(1)-Si(1')-Si(2') angle is 137.44 degrees ("trans-bent").
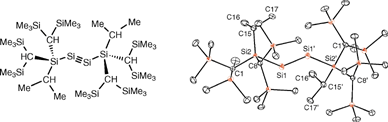
The full text of the article describing the preparation, characterization and reactivity of the compound can be found here. See figure 4 in the paper for a MO explanation as to why the trans-bent geometry occurs. Basically, the authors suggest that, "the bending is thought to be the result of the mixing of an in-plane $\ce{\pi}$-orbital with a $\ce{\sigma^{\ast}}$ orbital whose energies are close enough to cause the interaction."
-
1$\begingroup$ Very interesting, but it's hard to imagine the overlap. $\endgroup$– EJCCommented Sep 21, 2014 at 14:38
-
3$\begingroup$ I was impressed by the fact (above link, Section 4, paragraph 2) that the bond length contraction going from $\ce{Si=Si}$ to $\ce{Si#Si}$ is half that for $\ce{C=C}$ to $\ce{C#C}$. That seems significant to me. $\endgroup$– ronCommented Sep 21, 2014 at 15:10