2
$\begingroup$

This is a simple question but I can't seem to find a place where they use the same nomenclature... I was wondering whether these are all the same thing: $B_\mathrm{induced}$, $B_\mathrm{local}$, $B_\mathrm{effective}$? I know $B_\mathrm{effective} = B_0 - B_\mathrm{shielding}$. But do all of them mean the same thing (as in the magnetic field felt by the nucleus in a particular environment)? Thank you!

Edit (including context)

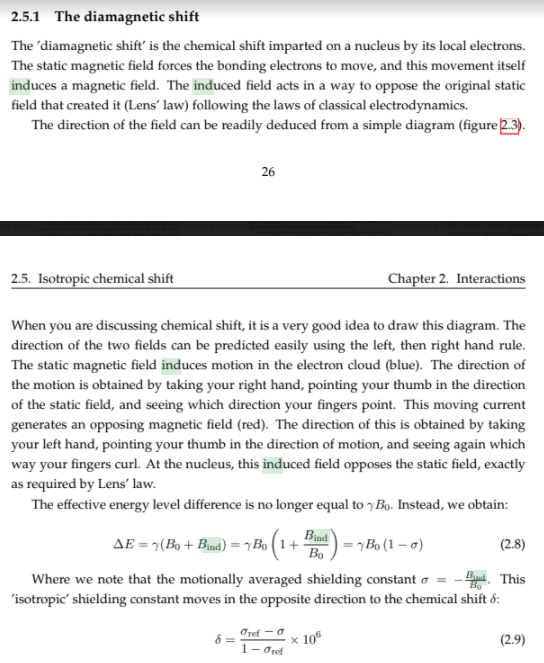
Context for $B_\mathrm{effective}$:

$\mathrm{\omega}$ = $\mathrm{\gamma}$$B_\mathrm{eff}$ = $\mathrm{\gamma}$$(B_\mathrm{0}-B_\mathrm{shielding})$

I can understand this, but I wanted to know if this $B_\mathrm{eff}$ is the same as induced, and local.

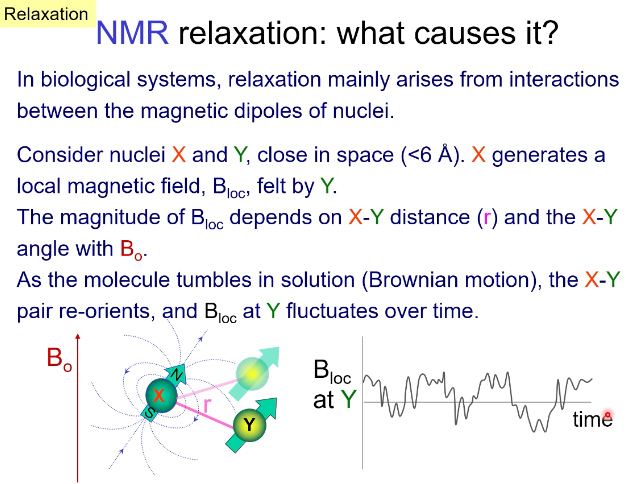
Context for $B_\mathrm{local}$:

B_local

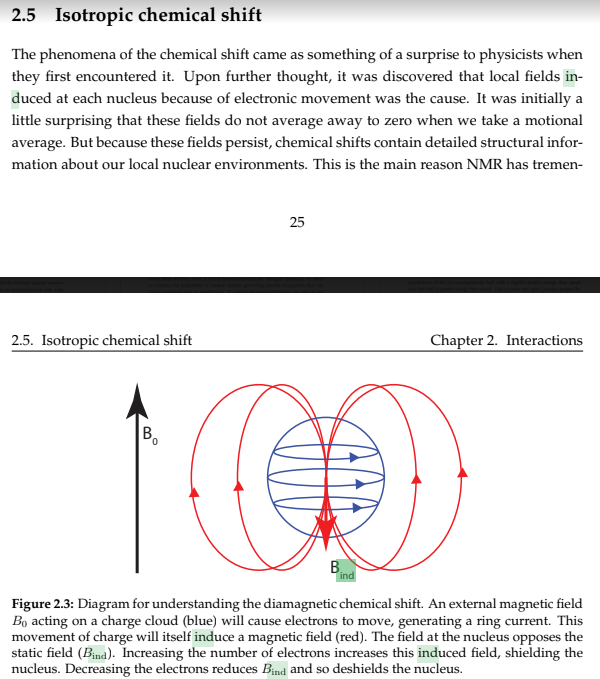
Context for $B_\mathrm{induced}$

B_ind 1

B_ind 2

Thank you!

Improve this question
$\endgroup$
3
  • 4
    $\begingroup$ +1 Welcome to Chemistry.SE! Please note the edits, we use MathJax to format equations and symbols: chemistry.meta.stackexchange.com/questions/86/… . I think it might be useful if you could also refer to the context in which $B_\mathrm{induced}$ and $B_\mathrm{local}$ are used. $\endgroup$
    – S R Maiti
    Commented Apr 17, 2022 at 9:35
  • 3
    $\begingroup$ The meaning of these words depends upon context. It may help if you could say what you are reading and quote passages where the words are used. $\endgroup$
    – 10ppb
    Commented Apr 17, 2022 at 15:18
  • 1
    $\begingroup$ Agree with above posters - context is required. $\endgroup$
    – orthocresol
    Commented Apr 17, 2022 at 19:20

1 Answer 1

Reset to default
5
$\begingroup$

In this context, i.e. the discussion of how the chemical shift arises, I'd assume that the induced magnetic field is the "internal" magnetic field, which is generated by electrons surrounding the nucleus, in response to the external magnetic field ($B_0$). This induced magnetic field generally serves to oppose the external magnetic field, meaning that the effective magnetic field is less than $B_0$. The equation you gave,

$$\omega = \gamma B_\mathrm{eff} = \gamma(B_0 - B_\mathrm{shielding})$$

suggests that $B_\mathrm{shielding}$ is to be identified with the induced magnetic field and that $B_\mathrm{eff}$ is what's left after removing that.

Note, however, that $B_\mathrm{eff}$ may mean something else in a different context (e.g. when discussing the vector model of NMR).

On the other hand, $B_\mathrm{local}$ is being used in a completely different scenario, namely that of nuclear spin relaxation. It refers to a magnetic field generated at the location of one nucleus due to other nuclei. (Think of every nucleus, or every NMR-active nucleus at least, being a bar magnet — $B_\mathrm{local}$ is the magnetic field generated by these bar magnets.)

This is in contrast to $B_\mathrm{shielding}$ above, which is generated by electrons around the nucleus of interest.

So none of them are the same.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.