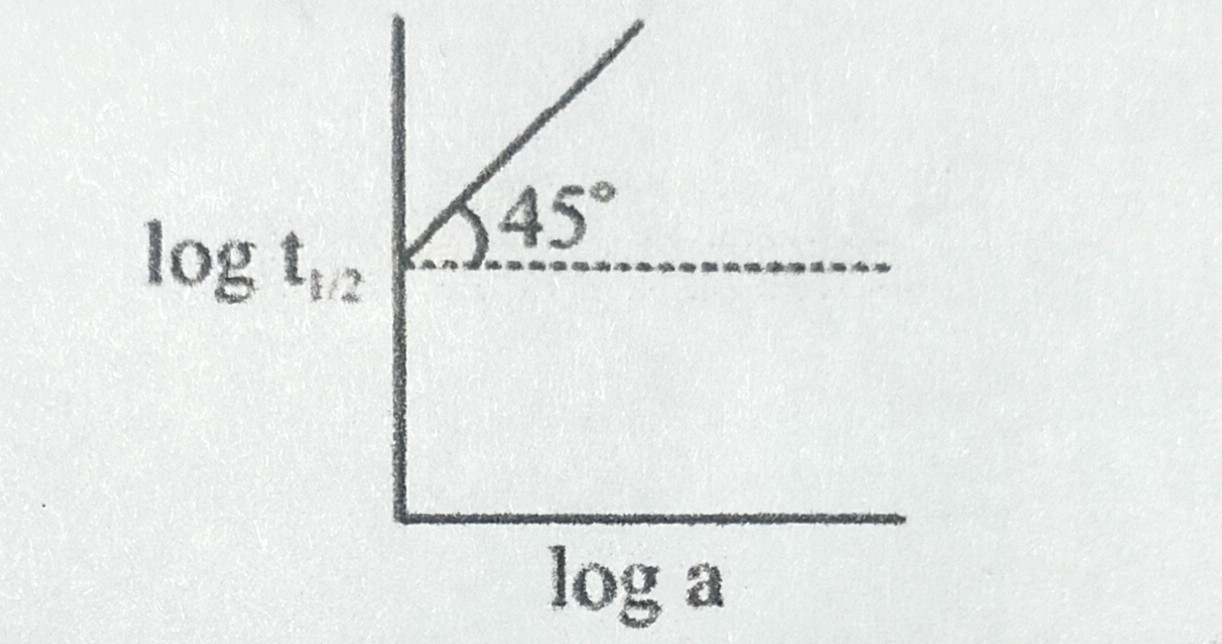Finding the reaction order from a given plot of chemical kinetics

Question

What will be the order of the reaction for a chemical change having $\log t_{1/2}$ VS $\log a$ Where $a=$ Initial concentration of reactant and $t_{1/2} =$ Half Life?

1. 0 order
2. 1st order
3. 2nd order
4. None of These

Actually the answer I found by searching in internet is option 1 but following my calculations, I am getting the answer as option 4.

My calculations are as follows:

For 0 order reaction: $$t_{1/2} = \frac{a}{2K}$$

Taking $\log$ on both sides:

$$\log t_{1/2} = \log{\frac{a}{2K}}$$

$$\log t_{1/2} = \log a - \log 2K \tag1$$

This is a Straight line equation of type $y= mx - C $.

MY DOUBT IS If you notice in graph you will get to know that the intercept is positive while in the equation $(1)$, intercept is negative so how is it possible?

My Background- actually I didn't have kept Mathematics as my major subject in senior secondary high school so I have little knowledge with respect to graph so please forgive me if I am wrong at formulating (1).

Answer 1

The equation that you have derived is correct. For a zeroth-order reaction, $$\log t_{1/2}=\log a-\log2K$$ By superficially observing, this seems to be giving a straight line with the equation $y=mx-c$ but actually there are several(not all) reactions in which the $K$ value is very small. In case of any reaction having $K\lt0.5$, $2K$ becomes less than $1$ and hence $\log2K$ becomes negative. Then you would obtain an equation of the form $y=mx+c$ whose graph will have a positive ordinate and it will appear similar to the graph in the question. So that graph in your question is correct for a zero-order reaction having a small rate constant (less than $0.5$).

Answer 2

The generic slope-intercept equation for a line is:

$$y=mx+c$$

When given angle $\theta$ for a straight line, the slope $m$ is calculated by:

$$m=tan\;\theta$$

In this case:

$$m=tan\;(45°)=1$$

Substituting $m$ into the generic slope-intercept equation for a line, and comparing it with your derived equation (1):

$$y=x+c$$

$$logt_{1/2}=log\;a-log\;(2K)$$

We can see that:

$$y=log\;t_{1/2}$$

$$m=1$$

$$x=log\;a$$

$$c=-log\;(2K)$$

The slope $m$ is not negative, since it's equal to 1.

As far as the intercept $c$ is concerned, as explained by other answers, the value of $K$ for this particular reaction rate is such that taking the base 10 logarithm of $2K$ value will itself result in a negative number, which will then become a positive number once mutiplied by the $-$ sign next to the logarithm function.

In other words, $log(2K)$ is negative and $-log(2K)$ is positive.

In conclusion, both $m$ and $c$ are positive in this problem.

Answer 3

For an $n$-th order reaction, $$ -\frac{\text{d}a}{\text{d}t}=ka^n $$ Integrating between the limit $0$ and $t_{1/2}$

$$\begin{align} k\int_0^{t_{1/2}}{\text{d}t} &= -\int_a^{a/2}{\frac{1}{a^n}\ \text{d}a} \end{align}$$

$$\begin{equation}\begin{aligned} kt_{1/2} &=-\frac{1}{1-n}\left(a^{1-n}\right)_a^{a/2} \\ &= \frac{1}{n-1}\left[\left(\frac{a}{2}\right)^{1-n}-a^{1-n}\right] \\ &= \frac{2^{n-1}-1}{n-1}a^{1-n} \\ t_{1/2} &= \frac{1}{k}\frac{2^{n-1}-1}{n-1}a^{1-n} \end{aligned}\end{equation}$$

$$ \ln(t_{1/2})=\ln\left(\frac{1}{k}\frac{2^{n-1}-1}{n-1}\right)+(1-n)\ln(a) $$ The slope of the straight line equation is $m=1-n$. Comapring with the graph, where $m=1$, we can see that $n=0$. The intercept, for $n=0$, is $-\ln(2k)$, and since in the graph slope is positive, what we can conclude is that $\bbox[yellow] {k<\pu{0.5 mol \ell^{-1} s^{-1}}} $