How does changing the concentrations of intermediate products/reactants change the rate law?

Question

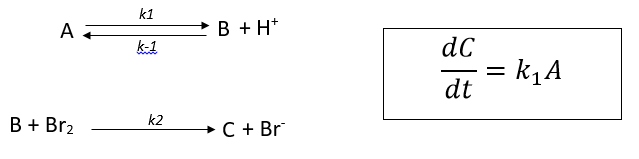
A = CH₂(CN)₂
B = CH(CN)₂^-
C = BrCH(CN)₂
The rate law above is derived from applying the steady state approximation.

I am trying to work out how to modify the rate law to accomodate the following conditions:

1) [Br₂] is low relative to [H⁺]

2) [Br₂] is high relative to [H⁺]

And in the 2nd case how this affects the RDS and order of reaction.

It seems to me like these conditions would just affect the rate coefficient, not actually change the rate law.

EDIT:

$$ \frac{d [CH(CN)_{2}^{-}]}{dt} = k_{1}[CH_{2}(CN)_{2}] - k2[CH(CN)_{2}^{-}][Br_{2}] - K_{-1}[CH(CN)_{2}^{-}][H^{+}] $$

$$ Applying\ Steady\ State\ Approximation: $$ $$ k_{1}[CH_{2}(CN)_{2}] - k2[CH(CN)_{2}^{-}][Br_{2}] - K_{-1}[CH(CN)_{2}^{-}][H^{+}] = 0 $$

$$ k2[CH(CN)_{2}^{-}][Br_{2}] + K_{-1}[CH(CN)_{2}^{-}][H^{+}] = k_{1}[CH_{2}(CN)_{2}] $$ $$ Dividing\ by\ [CH(CN)_{2}^{-}]: $$

$$ k2[Br_{2}] + K_{-1}[H^{+}] = \frac{k_{1}[CH_{2}(CN)_{2}]}{[CH(CN)_{2}^{-}]} $$

$$ [CH(CN)_{2}^{-}](k2[Br_{2}] + K_{-1}[H^{+}]) = k_{1}[CH_{2}(CN)_{2}] $$

$$ [CH(CN)_{2}^{-}] = \frac{k_{1}[CH_{2}(CN)_{2}]}{(k2[Br_{2}] + K_{-1}[H^{+}])} $$

$$ \frac{d [BrCH(CN)_{2}]}{dt} = {k_{2}[Br_{2}][CH(CN)_{2}^{-}]}{} $$

$$Rate\ Equation:$$

$$ \frac{d [BrCH(CN)_{2}]}{dt} = \frac{k_{2}[Br_{2}]k_{1}[CH_{2}(CN)_{2}]}{{k_{2}[Br_{2}]}+k_{-1}[H^{+}]} $$

So if $ [Br_{2}]$ is low compared to $ [H^{+}]$ then I would remove $ [Br_{2}]$ from the rate equation giving:

$$ \frac{d [BrCH(CN)_{2}]}{dt} = \frac{k_{1}[CH_{2}(CN)_{2}]}{k_{-1}[H^{+}]} $$

If $ [H^{+}]$ is low compared to $ [Br_{2}]$ then I would remove $ [H^{+}]$ from the rate equation giving:

$$ \frac{d [BrCH(CN)_{2}]}{dt} = k_{1}[CH_{2}(CN)_{2}] $$

So in this case the reaction is 1st order and the RDS no longer proceeds backwards with rate coefficient $k_{-1}$.

Is this right or have I made a mistake somewhere?

Answer 1

The steady state approximation allows us to assume that the concentration of $\ce{B}$ does not change.

$$\dfrac{d[\ce{B}]}{dt}=0$$

Thus the rates of production and consumption of $\ce{CH(CN)2-}$ must be equal.

$\ce{CH(CN)2-}$ can be produced by one pathway:

$$\ce{CH2(CN)2 ->[k_1] CH(CN)2- + H+} \\ \mathrm{rate}=k_1[\ce{A}]$$

$\ce{CH(CN)2-}$ can be consumed by two pathways:

$$\ce{CH(CN)2- + Br2 ->[k_2] BrCH(CN)2 + Br-} \\ \ce{CH(CN)2- + H+ ->[k_{-1}] CH2(CN)2} \\ \mathrm{rate}=k_2 [\ce{CH(CN)2-}][\ce{Br2}]+K_{-1}[\ce{CH(CN)2-}][\ce{H+}]$$

Thus:

$$\dfrac{d[\ce{CH(CN)2-}]}{dt}=k_1[\ce{CH2(CN)2}] -\left( k_2 [\ce{CH(CN)2-}][\ce{Br2}]+K_{-1}[\ce{CH(CN)2-}][\ce{H+}] \right)= 0$$

Now you can can solve for $[\ce{CH(CN)2-}]$ and substitute into the rate law equation for the second step:

$$\dfrac{d[\ce{BrCH(CN)2}]}{dt}=k_2[\ce{CH(CN)2-}][\ce{Br2}]$$

You should get a final rate law that contains $[\ce{CH2(CN)2}],\ [\ce{Br2}],\ \&\ [\ce{H+}]$, and then you can answer your questions.

Update based on revised question.

Your new rate late is correct:

$$\dfrac{d[\ce{BrCH(CN)2}]}{dt}=\dfrac{k_2[\ce{Br2}]k_1[\ce{CH2(CN)2}]}{k_2[\ce{Br2}]+k_{-1}[\ce{H+}]}$$

Your interpretation of the simplification you can do is correct for one case and almost correct for the other.

$[\ce{H+}] \ll [\ce{Br}]$

In this case, we assume that $k_{-1}[\ce{H+}]\ll k_2[\ce{Br}]$ so

$$k_2[\ce{Br2}]+k_{-1}[\ce{H+}] \approx k_2[\ce{Br2}] \\ \therefore \ \dfrac{d[\ce{BrCH(CN)2}]}{dt}\approx \dfrac{k_2[\ce{Br2}]k_1[\ce{CH2(CN)2}]}{k_2[\ce{Br2}]}\approx k_1[\ce{CH2(CN)2}$$

which is as you figured.

$[\ce{H+}] \gg [\ce{Br}]$

In this case, we assume that $k_{-1}[\ce{H+}]\gg k_2[\ce{Br}]$ so

$$k_2[\ce{Br2}]+k_{-1}[\ce{H+}] \approx k_{-1}[\ce{H+}] \\ \therefore \ \dfrac{d[\ce{BrCH(CN)2}]}{dt}\approx \dfrac{k_2[\ce{Br2}]k_1[\ce{CH2(CN)2}]}{k_{-1}[\ce{H+}]}$$

Note that this approximation does not remove the $k_2[\ce{Br2}]$ term in the numerator.