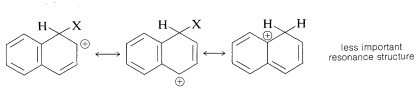
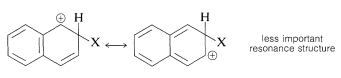
I read that Friedel Craft's alkylation of naphthalene by alkyl halides having more than 2 carbons occurs at 2-position. On the other hand, with smaller halides, it occurs at the 1-position.
Does it have something to do with steric factors or is there some other reason?
The 1-isomer is the generally favoured product as it is formed via a less energetic intermediate, whereas the 2-isomer is formed via a more energetic one.
The stabilization of the 1-isomer intermediate is due to the higher extent of resonance. Substitution Reactions of Polynuclear Aromatic Hydrocarbons[1] nicely sums up the point.
Substitution usually occurs more readily at the 1 position than at the 2 position because the intermediate for 1-substitution is more stable than that for 2-substitution. The reason is that the most favorable resonance structures for either intermediate are those that have one fully aromatic ring. We can see that 1-substitution is more favorable because the positive charge can be distributed over two positions, leaving one aromatic ring unchanged. Only one resonance structure is possible for the 2-substitution intermediate that retains a benzenoid-bond arrangement for one of the rings.
1-substitution
2-substitution
But as the size of the substituent increases there is one more factor to consider. As the substituent becomes bulkier, it's steric hindrance with the hydrogens of the neighbouring carbons increases.
This is an issue for the 1-isomer as it's steric interaction with the neighbouring carbons' hydrogen is way more than it's 2-isomer counterpart. This causes the 2-isomer to be the preferred product when the substituent is bulkier.
Following is an excerpt from intermediateorgchemistry's article[2] about naphthalene:
There is one other factor that will also effect the position of the substitution; that is the size of the group being added. In the case of large groups the ß position is favoured. This is a case of steric hindrance with the hydrogen's on neighbouring carbon atoms.
Reference:
(1) Roberts, J. D.; Caserio, M. C. Substitution Reactions of Polynuclear Aromatic Hydrocarbons https://chem.libretexts.org/@go/page/22330 (accessed Jun 12, 2021).
(2) naphthalene http://www.intermediateorgchemistry.co.uk/aromatic1.htm (accessed Jun 12, 2021).
I read that Friedel Craft's alkylation of naphthalene by alkyl halides having more than 2 carbons occurs at 2-position. On the other hand, with smaller halides, it occurs at the 1-position.
The above statement from OP clearly indicate that the substitution selection is due to steric reasons. I'd say the above belief is derived by the most of literature evidence, yet you can find a plathora of references contradicting the idea as well. The $\alpha$- and $\beta$-acetylation or alkylation is mainly depend on the conditions, catalysts, solvents, etc. For instance, acetylation of 2-methoxynaphthalene in carbon disulphide, chloroform, or nitrobenzene, has given various yields of 1-acetyl-2-methoxynaphthalene, 2-acetyl-6-methoxynaphthalene, 1-acetyl-7-methoxy-naphthalene, and small amounts of the corresponding phenols (Ref.1). It was reported that in carbon disulphide, 1-acetyl-2-methoxynaphthalene has been obtained in 44% yeild while it was 43% in nitrobenzene.
On the other hand, Zeolite catalyzed Friedel-Crafts acetylation of 2-methoxynaphthalene by acetic anhydride in acetic acid has given 2-acetyl-6-methoxynaphthalene with high selectivity, which is a key intermediate for an anti-inflammatory agent, Naproxen (Ref.2). The reaction has converted 82% of 2-methoxynaphthalene to 2-acetyl-6-methoxynaphthalene (major, 86%) and 1-acetyl-2-methoxynaphthalene (minor, 14%).
Nonetheless, the Friedel-Crafts acetylation of unsubstituted naphthalene is of particular interest, since it was shown that the $\alpha/\beta$-isomer ratio was concentration-dependent. The results obtained for 1,2-dichloroethane solution pointed to a duality of mechanism, in that $\beta$-acetylation is first-order in acylating reagent $\ce{(AcCl.AlCl3)}$, whereas $\alpha$-acetylation is second-order in the acylating reagent. The paper (Ref.3) describeing the kinetic studies of the Friedel-Crafts acetylation of naphthalene in 1,2-dichloroethane states that:
The Friedel-Crafts acetylation of naphthalene, using acetyl chloride and aluminum chloride in 1,2- dichloroethane solution, has been studied kinetically. The $\alpha/\beta$ isomer ratio changes as a function of concentration of reactants, and time, e.g., from an initial 4-5 to a final 0.7. The results point to a different dependence for the two positions on the concentration of acylating reagent, $\ce{(AcCl.AlCl3)}$, being second-order in this reagent for the $\alpha$-reaction and first-order for the $\beta$-reaction. The rate of the $\alpha$-reaction is impeded by the presence of free acetyl chloride, whilst the $\beta$-reaction is unaffected. The latter reaction exhibits activation parameters in the expected range, $\Delta H^\ddagger = ca. \pu{48 kJ mol-1}$ and $\Delta S^\ddagger = ca. \pu{-99 J K-1 mol-1}$, whereas for the a-reaction $\Delta H^\ddagger = ca. \pu{21 kJ mol-1}$ and $\Delta S^\ddagger = ca. \pu{-160 J K-1 mol-1}$ are both very low. Competitive and non-competitive kinetic hydrogen isotope experiments were carried out using $\ce{[^2H_8]}$ naphthalene. The mechanism for $\beta$-naphthyl acetylation is believed to involve a two-stage process, the second (loss of proton) being rate-limiting. The $\alpha$-acetylation is believed to proceed through a $\sigma$-complex, from which elimination of $\ce{HCl}$ to give products is prevented for steric reasons; the reaction instead proceeds through a second $\sigma$-complex, decomposition of which is usually at least partly rate-limiting.
The initial formation of the $\alpha$-isomer is rapid but its curve soon flattens out, whilst that of the $\beta$-isomer climbs more steadily and overtakes the $\alpha$-isomer after $ca. \pu{4 min}$. Formation of the $\alpha$-isomer is virtually complete after $\pu{10 min}$, but the $\beta$-isomer continues to increase for $\pu{1 h}$. The total yield of acetyl isomers was usually 90-95% (for all experiments).
Computating their data on different computer programs, the authors have concluded that the only set of equations which gave satisfactory results, i.e., internal consistency and replicability, involved the equations $(1)$ and $(2)$: $$\text{Rate}_\alpha = k_\alpha \ce{[naphthalene][AcCl.AlCl3]^2} \tag1$$ $$\text{Rate}_\beta = k_\beta \ce{[naphthalene][AcCl.AlCl3]} \tag2$$
Following shows the isotope effect $(\frac{k_\ce{H}}{k_\ce{D}})$ of acylation of two positions:
$$ \begin{array}{c|ccc} \hline \text{Product} & \ce{[AcCl.AlCl3]}, \ \pu{mol L-1} &\ce{[AcCl]_{free}}, \ \pu{mol L-1} & \frac{k_\ce{H}}{k_\ce{D}} \text{ uncorr.} & \frac{k_\ce{H}}{k_\ce{D}} \text{ corr.} \\ \hline \text{1-acetylnaphthalene} & 0.0041 & 0.0942 & 2.17 & 2.22 \\ & 0.0337 & \text{trace} & 1.09 & 1.09 \\ & 0.0168 & 0.0725 & 1.236 & 1.243 \\ & 0.0174 & 0.0751 & 1.70 & 1.61 \\ \hline \text{2-acetylnaphthalene} & 0.0041 & 0.0942 & 4.11 & 4.35 \\ & 0.0337 & \text{trace} & 5.07 & 5.39 \\ & 0.0168 & 0.0725 & 5.04 & 5.40 \\ & 0.0174 & 0.0751 & 5.01 & 5.35 \\ \hline \end{array} $$
The $\frac{k_\ce{H}}{k_\ce{D}}$ clearly shows the two mechanism for each substitution at $\alpha$- and $\beta$-possitions.
References:
