How are there two C3 rotation axes in ammonia?

Question

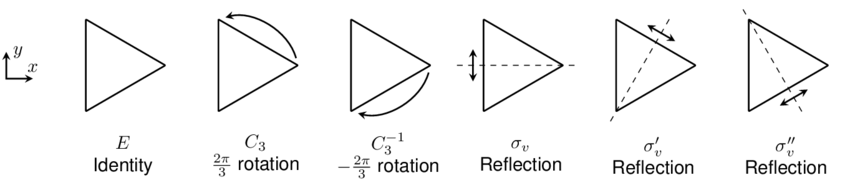
If we look at the character table for the $C_\mathrm{3v}$ point group (which $\ce{NH3}$ is an example of), we see that these are the symmetry elements ${E, 2 C_3, 3 \sigma_v}$ present in the molecule. My question is — why are there $2 C_3$ rotation axes? Shouldn't there be only one, which is perpendicular to the plane containing the three hydrogens?

The $C_\mathrm{3v}$ character table:

$$\begin{array}{c|ccc|cc} \hline C_\mathrm{3v} & E & 2C_3 & 3\sigma_\mathrm{v} & & \\ \hline \mathrm{A_1} & 1 & 1 & 1 & z & x^2+y^2, z^2 \\ \mathrm{A_2} & 1 & 1 & -1 & R_z & \\ \mathrm{E} & 2 & -1 & 0 & (x,y), (R_x,R_y) & (x^2-y^2,xy), (xz,yz) \\ \hline \end{array}$$

Answer 1

In a character table for $C_{3v}$, $ 2C_3$, means that the element is actually 2 $C_3$ elements in the same conjugacy class. That means the two $C_3$ operations are related in some way.

In fact, it means they share the same single axis. One $C_3$ operation is a $120^\circ$ turn around the axis, and the other $C_3$ operation is a $240^\circ$ turn around the same axis in the same direction - or equivalently a $120^\circ$ turn around the same axis in the opposite direction.

In the image below the two elements $C_3$ and $C_3^{-1}$ are the two symmetry elements of the $2C_3$ class, which are rotations about the same axis out of the plain of the image.

Symmetry elements of the $C_{3v}$ point group Image taken from Fig S5 in supplementary information of T. Karin, et al, Phys. Rev. B 94, 041201

Answer 2

The top row of the character table does not refer to symmetry elements, but rather symmetry operations. (For the difference between the two, see Wikipedia:Molecular symmetry.)

In this particular case, the difference is that there is only one $C_3$ symmetry element in ammonia (i.e. the rotation axis), but there are two operations: one corresponds to the clockwise rotation, and the other to the anticlockwise rotation (which is equivalent to two clockwise rotations, should you prefer that).

It is the symmetry operations, not the elements, which form the so-called "group" in group theory (see Wikipedia:Group (mathematics)).

In mathematics, a group is a set equipped with a binary operation that combines any two elements to form a third element in such a way that three conditions called group axioms are satisfied, namely associativity, identity and invertibility.

(Emphasis mine.) The "binary operation" for symmetry operations is to simply perform one operation after another, which is somewhat akin to composition. Note that the word "element" in the quoted paragraph refers to an abstract member of the group: it is mildly unfortunate that the elements are symmetry operations, not symmetry elements.