2
$\begingroup$

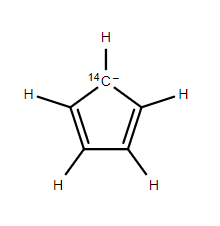
enter image description here

Is there any difference between electronegativities of isotopes of the same element. For example consider the above compound. Will all the resonance structures be equivalent?

Also if I place the above compound in water (which will act as a Bronsted acid), which carbon will get the H atom?

Improve this question
$\endgroup$
4
  • $\begingroup$ An equally intriguing question could be how the resonance hybrid might be represented in this case. $\endgroup$
    – William R. Ebenezer
    Commented May 13, 2020 at 11:23
  • $\begingroup$ @orthocresol Can you please explain why a negative charge on a $\ce{_{14} C}$ would be different then a negative charge on a $\ce{_{12} C}$. Is there a difference of electronegativities (hence the question)? $\endgroup$
    – gauri agrawal
    Commented May 13, 2020 at 13:38
  • 4
    $\begingroup$ It's really just a wording nitpick. 14C isn't the same thing as 12C; thus a negative charge on 14C isn't the same thing as a negative charge on 12C. Asking whether the resonance structure are "equivalent" has the answer "no", because they aren't the same thing. Chemically speaking, it may of course be that the difference is so small so as to be completely negligible. I am guessing that is the point of your question. But we don't describe that as "equivalence". We'd describe it by saying that they contribute (almost) equally. $\endgroup$
    – orthocresol
    Commented May 13, 2020 at 14:11
  • 1
    $\begingroup$ @orthocresol , the background of this question was what happens when we place this compound in water. Where would the hydrogen get placed in the acid-base reaction? This would be based on which resonating structure would be more stable, in turn giving different carbons a different negative charge. My guess was that maybe it was due to electronegativities, but I may be wrong. $\endgroup$
    – gauri agrawal
    Commented May 13, 2020 at 14:41

3 Answers 3

Reset to default
2
$\begingroup$

enter image description here (Star represents $\ce{^14C}$ atom)

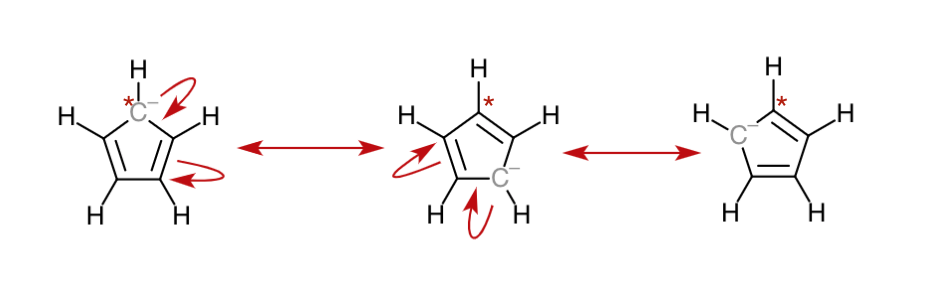
This figure represents three out of the five possible resonance structures. Of these five structures, four have a negative charge on a $\ce{^12C}$ atom whereas one has negative charge on $\ce{^14C}$ atom.

As the first premise, let's consider stability of the two kinds of resonating structures. The more stable the resonating compound, the more likely it is to be protonated.

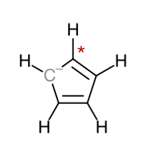
enter image description here enter image description here

We can compare stability using the premise of electronegativity (or rather charge density). According to this property, the more heavy an atom, the larger is its volume. If volume increases, the charge density decreases making it less likely to be able to stabilize the negative charge (becomes less electronegative). Due to this we can say that the resonance structure with $\ce{^14C}$ having the negative charge is less stable and so is less likely to be protonated.

Now, moving onto the second premise, the number of resonating structures containing the negative charge on $\ce{^14C}$ and $\ce{^12C}$ respectively.

If you notice only one resonating structure has a $\ce{^14C}$ with the negative charge, whereas there are $4$ resonating structures containing $\ce{^12C}$ atom with the negative charge. This would mean that probabilistically (considering stability to be equal) The resonance structure having a $\ce{^12C}$ with the negative charge would be more likely to be attacked.

Now, if we combine the two premises, we see that both stability and frequency of the resonance structure containing $\ce{^12C}$ with negative charge is greater its $\ce{^14C}$ counterpart. Concluding, we can say that the resonating structure with $\ce{^12C}$ stabilizing the negative charge would be more likely to be protonated.

Further Reading on how stability of isotopic compounds can be determined : Inductive effect of hydrogen isotopes

Improve this answer
$\endgroup$
0
$\begingroup$

A tentative reasoning could be made on the basis of charge density (on nucleus).

More number of neutrons means larger size of nucleus, but with the same number of protons. Hence, positive charge density (on nucleus) will get reduced, and hence the electronegativity.

But these comparisons can be made on numbers only, as discussed in the comments.

EDIT: (after I read the marked answer carefully, I found that I'd not answered the second part of question. So, here it goes.)

As the $\ce{^{14}C}$ isotope is less EN, therefore it is relatively less stable while carrying a negative charge on it. Hence, protonation would happen on a stable intermediate, which is $\ce{^{12}C}$.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Intuitively it's not clear how extra neutral will impact electronegativity. Will it make protons more sparse in the nucleus? Or vice versa? I assume denser protons would decrease electronegativity while sparser protons would increase it? $\endgroup$
    – Stanislav Bashkyrtsev
    Commented Jun 14, 2020 at 10:09
  • $\begingroup$ @StanislavBashkyrtsev, I guess I thought it the other way. But, here's what I think: more number of neutrons will decrease charge density and hence the $\ce{Z_{eff}}$, which in turn makes it easy for the atom to lose electrons, and therefore make it, relative to its isotope, a good e-donor. Hence, reduces its electronegativity. Am I missing something? $\endgroup$
    – Rahul Verma
    Commented Jun 14, 2020 at 15:07
  • $\begingroup$ @StanislavBashkyrtsev This may help $\endgroup$
    – Safdar Faisal
    Commented Jul 13, 2020 at 9:43
-1
$\begingroup$

Yes, it is there due to the increased size of nucleus. But, the difference in the electronegativity of the two isotopes would be so small that you can easily neglect it.

The resonating structure will be different in $\ce{^14C}$ and $\ce{^12C}$. The reason is $\ce{^14C}$ is radioactive (a very small effect) so it may cause a difference between the chemical properties of the two resonating structures.

Improve this answer
$\endgroup$
2
  • $\begingroup$ so where will hydrogen be added if we react this with water $\endgroup$
    – gauri agrawal
    Commented May 14, 2020 at 4:08
  • 1
    $\begingroup$ An isotope is defined as an element that has similar chemical properties but varied physical properties due to a different number of neutrons in the nucleus. So your premise feels flawed. $\endgroup$
    – Safdar Faisal
    Commented Jul 13, 2020 at 9:40

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.