Wavelength-dependence of refractive index in internal reflection spectroscopy?

Question

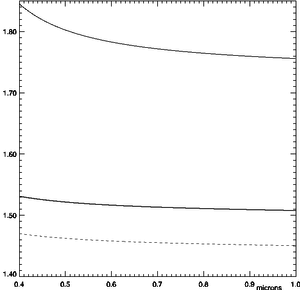
In many literature sources (web example), only a single value for the refractive index is assumed for the infrared element, and another is typically assumed for the sample substance. However, should not the refractive index be wavelength-dependent? So the wavelength-dependence of the penetration depth,

\begin{equation} d_p = \frac{\lambda}{2\pi n_1 \sqrt{\sin^2\theta - (n_2/n_1)^2}} \end{equation}

would not only be in the numerator, but also in the denominator: $n_1 = n_1(\lambda)$ and $n_2 = n_2(\lambda)$? Here $d_p$ is the penetration depth, $\lambda$ is wavelength, $\theta$ is the incident angle, and $n_1$ and $n_2$ are the refractive indices of the infrared element and sample, respectively.

Answer 1

Yes it should, but it doesn't matter much.

In physics, roughly speaking, everything depends on everything. We wouldn't be able to do a thing if we couldn't tell what is important and what isn't. Now let's see how much the refractive index actually changes as $\lambda$ goes, say, from 400 to 800nm (thus covering the entire visible range).

(source)

Looks like $n$ of a heavy flint glass goes from 1.85 to 1.75 (that's a change of 5%, in most other materials even less), while $\lambda$ changes by... how many percent?