When a problem requires calculations using values, always write the values with the correct units and carry the units through the calculation. Do not omit the units while performing intermediate steps and do not just reintroduce units at the end of the calculation.
Nevertheless, your approach is correct. You calculated the volume of the solution as
$$\begin{align}
V_\text{solution}&=\frac{23}{30\ \mathrm{ml^{-1}}}\tag{1}\\[6pt]
&=0.77\ \mathrm{ml}\tag{2}
\end{align}$$
the concentration as
$$\begin{align}
c&=\frac n{V_\text{solution}}=\frac m{M\cdot V_\text{solution}}\tag{3}\\[6pt]
&=\frac \gamma M\tag{4}\\[6pt]
&=\frac{1.2\times10^{-4}\ \mathrm{g\ ml^{-1}}}{284.48\ \mathrm{g\ mol^{-1}}}\tag{5}\\[6pt]
&=4.2\times10^{-7}\ \mathrm{mol\ ml^{-1}}\tag{6}
\end{align}$$
and the amount of stearic acid as
$$\begin{align}
n&=c\cdot V_\text{solution}\tag{7}\\[6pt]
&=\frac{1.2\times10^{-4}\ \mathrm{g\ ml^{-1}}}{284.48\ \mathrm{g\ mol^{-1}}}\cdot\frac{23}{30\ \mathrm{ml^{-1}}}\tag{8}\\[6pt]
&=3.2\times10^{-7}\ \mathrm{mol}\tag{9}
\end{align}$$
Furthermore, you can calculate the area of the surface as
$$\begin{align}
A&=\pi r^2\tag{10}\\[6pt]
&=\frac{\pi d^2}4\tag{11}\\[6pt]
&=\frac{\pi \left(15.5\ \mathrm{cm}\right)^2}4\tag{12}\\[6pt]
&=189\ \mathrm{cm^2}\tag{13}
\end{align}$$
For simplicity’s sake, you may assume that a single stearic acid molecule is a small block with a length $l$ and a width $w$.
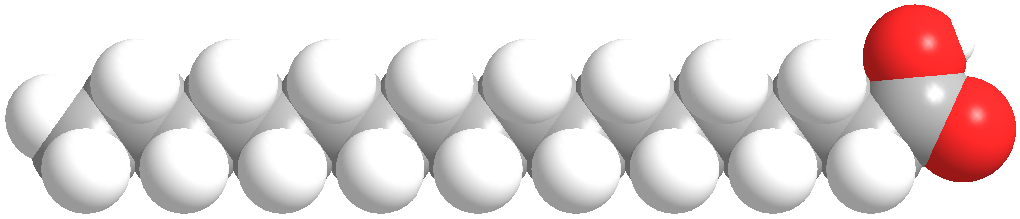
Depending on the used model, you may find that the ratio is roughly
$$l=6w\tag{14}$$
Assuming that the surface is densely packed with stearic acid molecules, the number $N$ of molecules can be estimated as
$$N=\frac A{w^2}\tag{15}$$
The corresponding volume of stearic acid is
$$\begin{align}
V&=A\cdot l\tag{16}\\[6pt]
&=A\cdot 6w\tag{17}
\end{align}$$
You also know that
$$\begin{align}
V&=\frac m\rho\tag{18}\\[6pt]
&=\frac {n\cdot M}\rho\tag{19}
\end{align}$$
where $\rho$ is the density of stearic acid. Instead of using the density of solid stearic acid at room temperature, you may want to assume the density of liquid stearic acid close to its melting point, which is
$$\rho=0.847\ \mathrm{g\ cm^{-3}}\tag{20}$$
Solving $\text{(17)}$ for $w$ and inserting the result into $\text{(15)}$ yields
$$N=A\cdot\left(\frac{6A}{V}\right)^2\tag{21}$$
And inserting $\text{(19)}$ for $V$ yields
$$N=A\cdot\left(\frac{6A\cdot\rho}{n\cdot M}\right)^2\tag{22}$$
The Avogadro constant is defined as
$$N_\mathrm A=\frac Nn\tag{23}$$
Inserting $\text(22)$ for $N$ yields
$$\begin{align}
N_\mathrm A&=\frac{A\cdot\left(\frac{6A\cdot\rho}{n\cdot M}\right)^2}{n}\tag{24}\\[6pt]
&=\left(\frac{6\rho}{M}\right)^2\left(\frac An\right)^3\tag{25}\\[6pt]
&=\left(\frac{6\times0.847\ \mathrm{g\ cm^{-3}}}{284.48\ \mathrm{g\ mol^{-1}}}\right)^2\left(\frac{189\ \mathrm{cm^2}}{3.2\times10^{-7}\ \mathrm{mol}}\right)^3\tag{26}\\[6pt]
&=6.6\times10^{22}\ \mathrm{mol^{-1}}\tag{27}
\end{align}$$
Even when taking the uncertainty of the used values into account, this result is significantly smaller than the literature value of the Avogadro constant. Therefore, you may conclude that a large part of the difference may be attributed to experimental error. I guess, you did not use the floating device method to push the stearic acid molecules together, which makes sure that the molecules are densely packed and lined up on the surface of the water with polar heads on the surface and nonpolar tails sticking up away from the surface.

