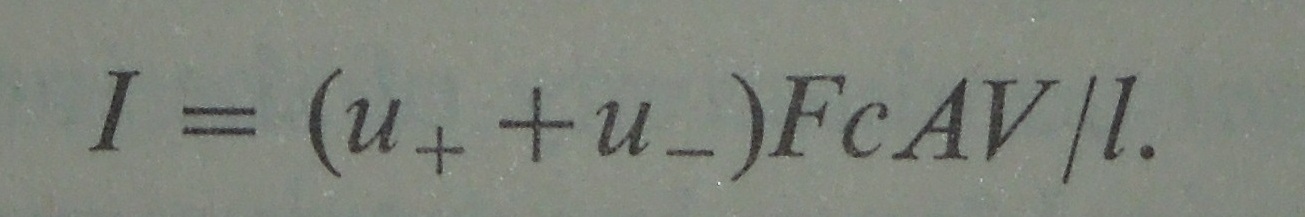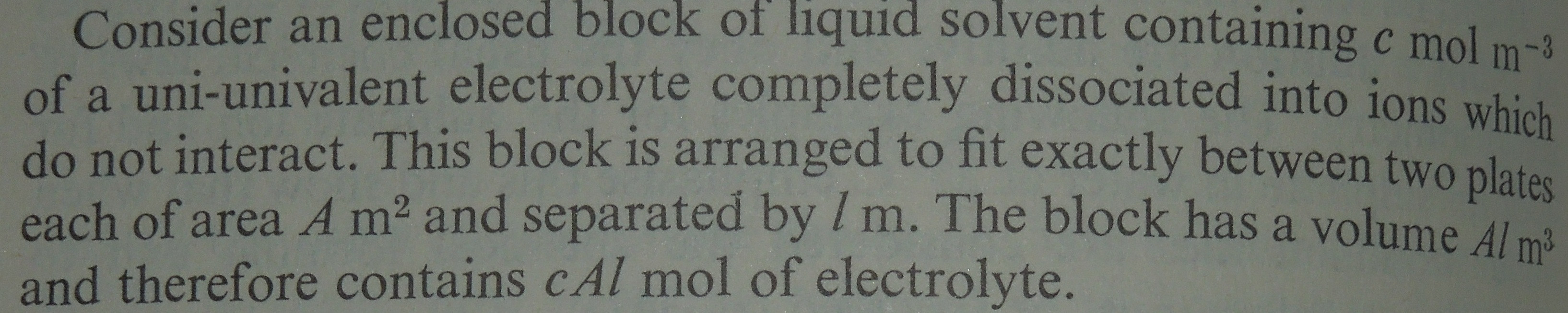Deriving the equation for ionic current

Question

High-schooler here reading up on electrochemistry.....

I came across this equation (and its derivation) in the book Physical Chemistry, by Wallwork and Grant:

Now the thing is, the equation is valid for 'uni-univalent' electrolytes (like KCl). But I want a more....'versatile' formula.

So I attempted to modify that equation to suit electrolytes of the type AB (i.e- magnitude of charge on a formula unit cation is equal to that of the charge on a formula unit anion).

I wrote down the whole thing (my formatting skills are terrible, so to type it out would be a HUGE pain in the neck), pardon the handwriting, I hope it's legible enough....

As for that last question.....I was wondering if the equation could be made even more 'versatile' 3:)

( @getafix don't think of me as clingy but...I'm counting on you for this one too! )

Answer 1

I will be using the following equation from my previous answer

$$I = (z \mu \nu c F E)A$$

You are interested in an electrolyte of the type $\ce{A^{2+}}$ $\ce{B^{2-}}$.

So, the "cation" current is

$$I_+ = (z_+ \mu_+ \nu_+ c F E)A$$

and similarly the "anion" current is

$$I_- = (z_- \mu_- \nu_- c F E)A$$

Here, $z_+ = z_- = z$ and $\nu_+ = \nu_- = \nu$. The mobilities, however, are different, so $\mu_+ \neq \mu_-$

So combining the contribution from the cations and anions in the solution, we get a total current

$$\mathrm{I}_{\text{tot}} = (z \nu c F EA)(\mu_+ + \mu_-)$$

After substituting $\ E = \frac{V}{L}$, one gets the desired result

$$\mathrm{I}_{\text{tot}} = \left (z \nu c FA \frac{V}{L}\right ) (\mu_+ + \mu_-)$$

In general, if $z_+ \neq z_- $ and $\nu_+ \neq \nu_-$ then simply write down the individual expressions for cationic and anionic contributions to current and add them.