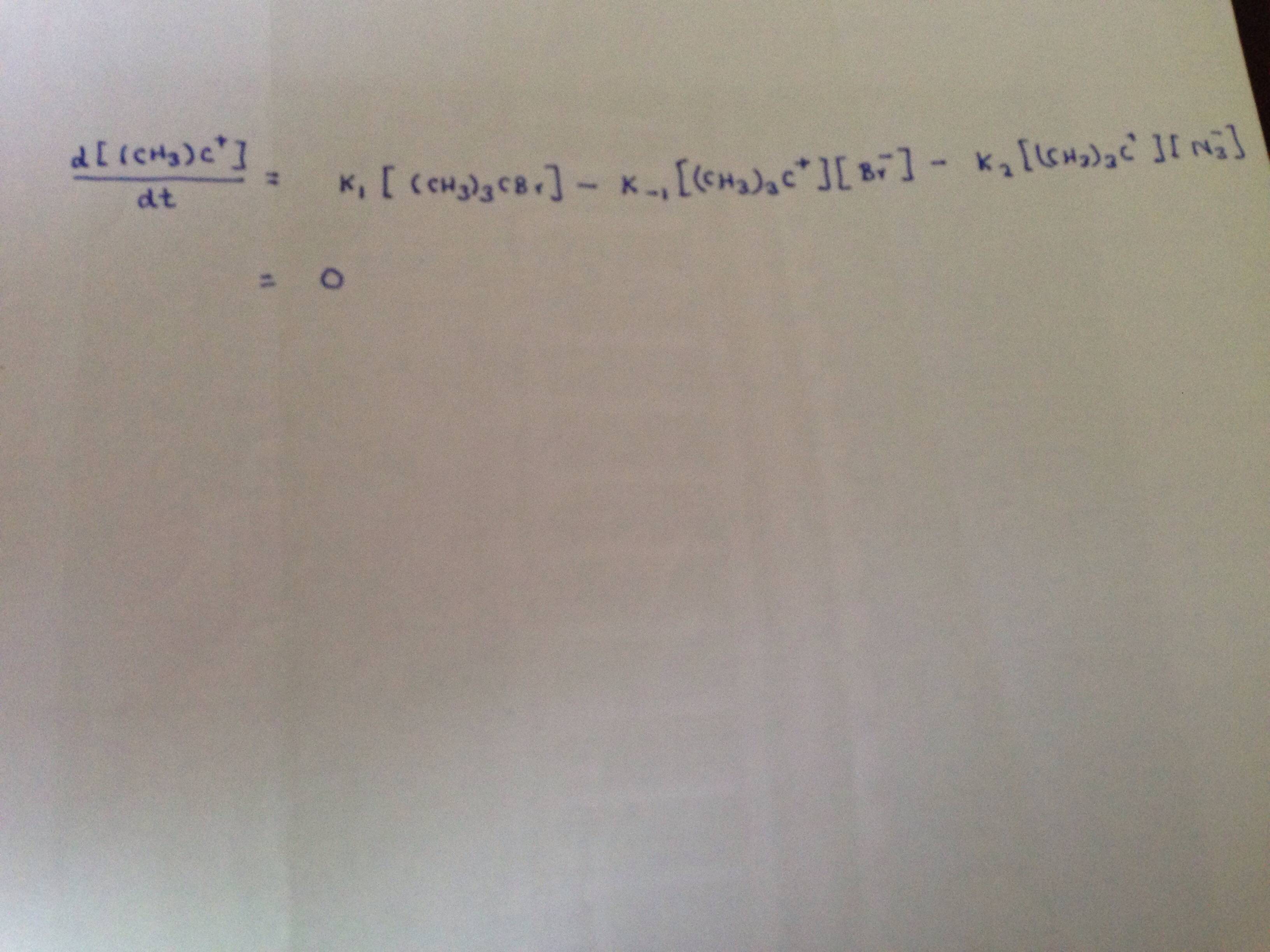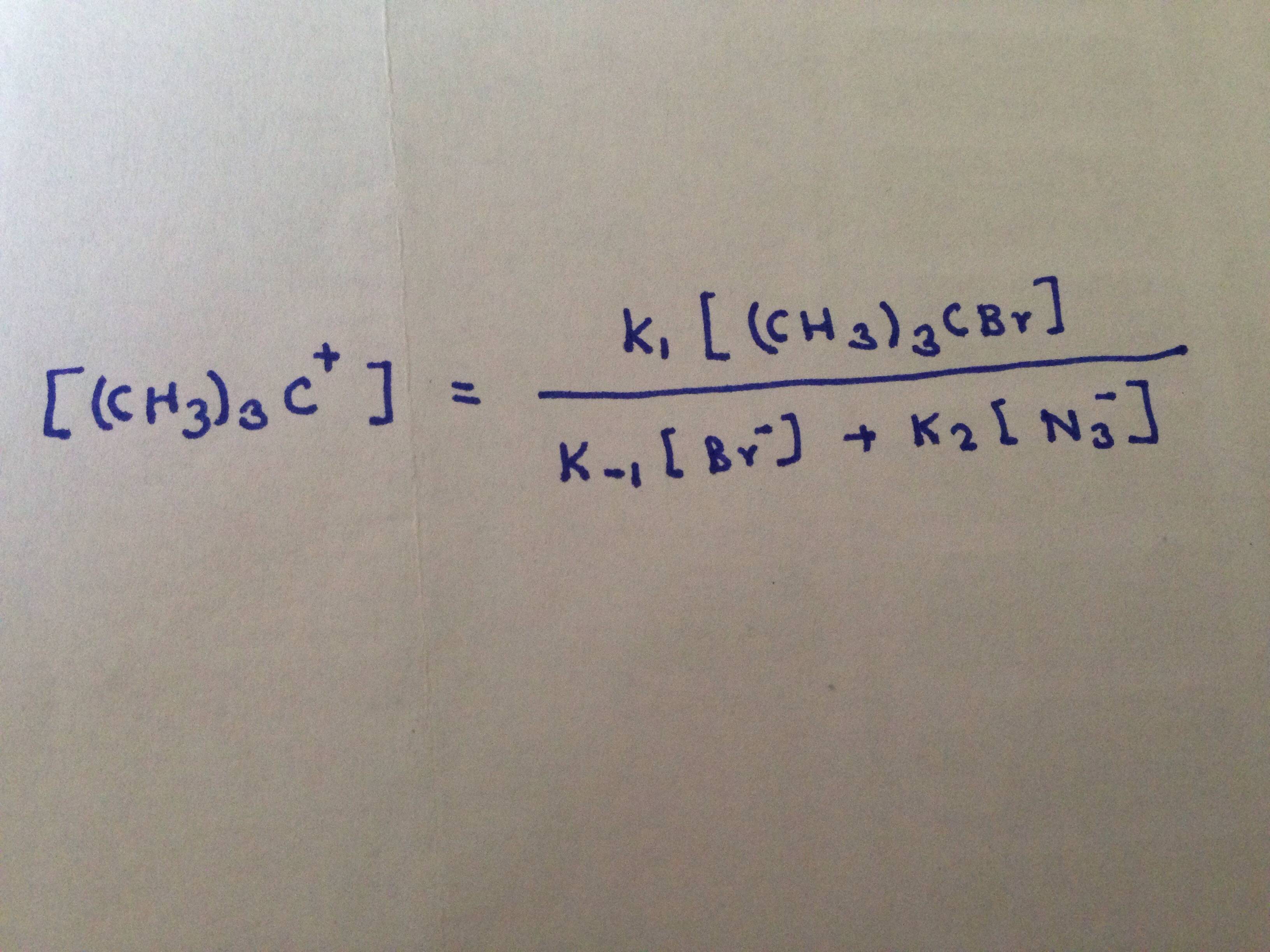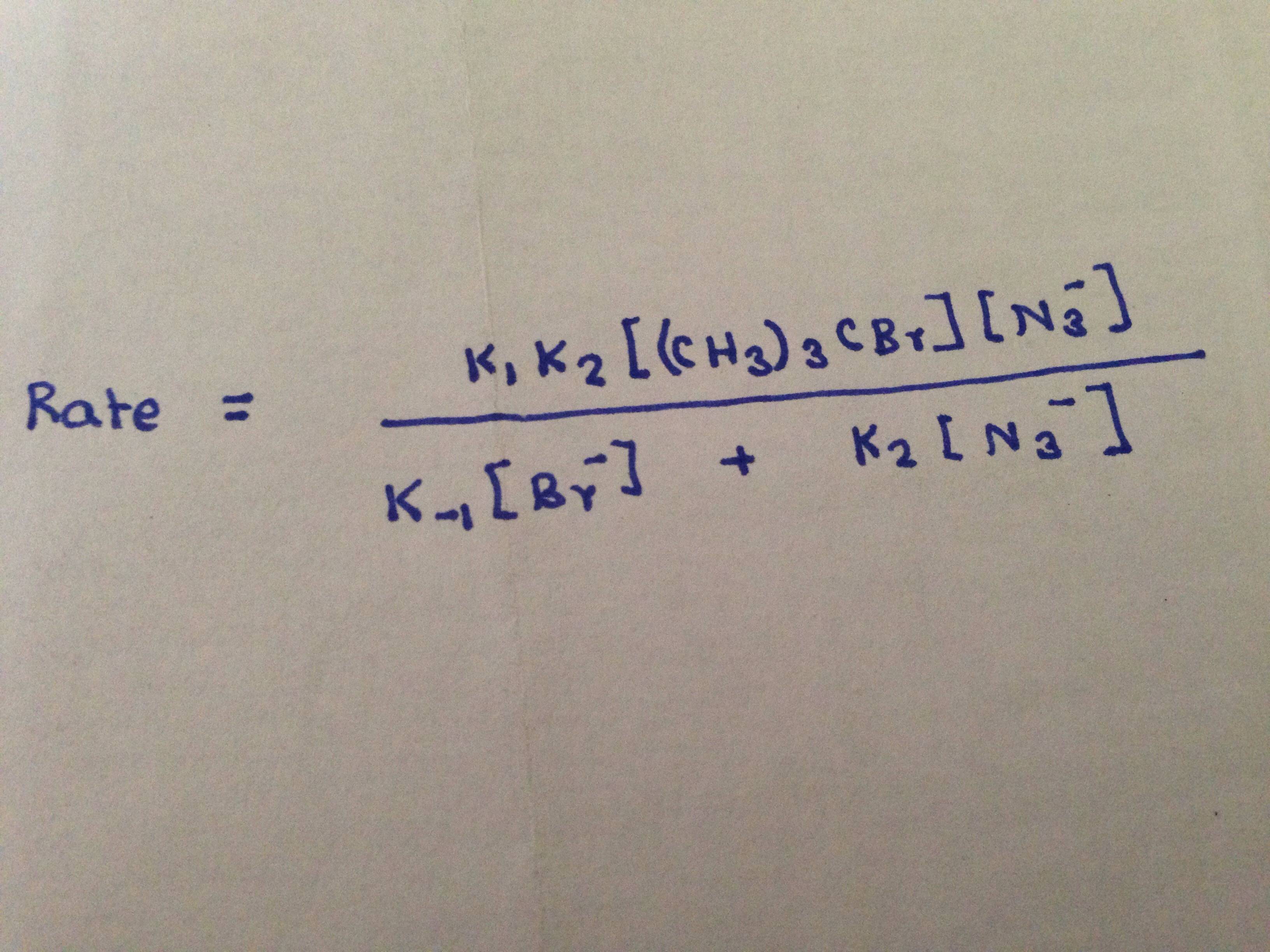3
$\begingroup$

The reaction of tert-butyl bromide with azide ion in aqueous solution is proposed to proceed by the following mechanism:

$\ce{(CH3)3CBr(aq) <=>(CH3)3C+(aq) + Br–(aq)}$

$\ce{(CH3)3C+(aq) + N3–(aq) \to (CH3)3CN3(aq)}$

Assuming that $\ce{(CH3)3C+ (aq)}$ achieves a steady-state concentration, but making no further assumptions about the relative magnitudes of the three rate constants, what is the rate law for this reaction, where $k_1$ is the forward reaction rate of the first equation, $k_{-1}$ is the reverse reaction rate of the first equation, and $k_2$ is the forward reaction rate of the second equation?

(A) $\ce{k_1[(CH3)3CBr]}$

(B) $\ce{k_2[(CH3)3CBr][N3-]}$

(C) $\ce{\displaystyle\frac{k_1k_2[(CH3)3CBr][N3-]}{k_{-1}[Br-]}}$

(D) $\ce{\displaystyle\frac{k_1k_2[(CH3)3CBr][N3-]}{k_{-1}[Br-] + k2[N3-]}}$

My thoughts: First of all, I read that $\ce{(CH3)3C+ (aq)}$ is a steady state concentration, which meant that $\frac{d\ce{[(CH3)3C+ (aq)}]}{dt} = 0,$ which prompted me to write out differential equations for each reactant. Thus, I got a system of differential equations: $$\frac{d\ce{[(CH3)3CBr (aq)}]}{dt} =-\frac{k_1}{k_{-1}}\ce{[(CH3)3CBr (aq)}]$$ $$0=\frac{d\ce{[(CH3)3C+ (aq)}]}{dt} = -\frac{d\ce{[(CH3)3CBr (aq)}]}{dt} - \frac{d\ce{[(CH3)3CN3 (aq)}]}{dt} = \frac{k_1}{k_{-1}}\ce{[(CH3)3CBr (aq)}] -k_2 \frac{d\ce{[(CH3)3CN3 (aq)]}}{dt}$$ $$\frac{d\ce{[Br- (aq)}]}{dt} = -\frac{d\ce{[(CH3)3CBr (aq)}]}{dt} = \frac{k_1}{k_{-1}}\ce{[(CH3)3CBr (aq)}]$$ $$\frac{d\ce{[N3- (aq)}]}{dt} = -k_2\ce{[N3-(aq)]}$$

But I was unsure of how to use these differential equations to actually find the rate law. Could anyone provide any suggestions?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

You really don't have to write all that differential equations.

Note: I'll write all my steps on a paper, since MathJax formatting is a little time-taking at the moment.

Consider the rate laws for the two reactions: enter image description here

Since the $\ce{[(CH3)3C+]}$ isn't changing with time, we get enter image description here

From which, you get

enter image description here

Plugging these in the Rate2 which I mentioned, you will get, enter image description here

Which is the same as what's given in option (D).

I hope I have satisfied your queries.

Improve this answer
$\endgroup$
8
  • $\begingroup$ Sorry, but how did you get your first rate law? $\endgroup$
    – Teoc
    Commented Apr 24, 2017 at 5:18
  • $\begingroup$ Those rate laws are directly derived from the reaction. For example, in an elementary reaction: $$\ce{mA + nB -> C}$$ The rate law would be $$Rate=[A]^m+[B]^n$$ $\endgroup$
    – Pritt says Reinstate Monica
    Commented Apr 24, 2017 at 5:22
  • $\begingroup$ According to my book rate would be $k[A]^m [B]^n$ is that just a typo on your part? Additionally, how did you derive the rate law for reactions in equilibrium? $\endgroup$
    – Teoc
    Commented Apr 24, 2017 at 5:32
  • 1
    $\begingroup$ You should really take a look at this so you can correctly format your future posts. $\endgroup$
    – ringo
    Commented Apr 24, 2017 at 5:43
  • 1
    $\begingroup$ @PrittBalagopal Wouldn't the rate be the negative of what you wrote, because we are measuring the rate of formation of product, not reactant? $\endgroup$
    – Teoc
    Commented Apr 24, 2017 at 15:30

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.