Do conformations arise from vibrations?

Question

I was wondering how a molecule transforms from one conformation to another. Surely, since the conformations are separated by a potential barrier, some activation energy must be required. The energies related to a molecule are translational, rotational, vibrational, and electronic. Out of these, translational and rotational motion do not change the intrinsic coordinates of a molecule and won't bring about a conformational change. The electronic transitions are too fast on the conformational time scale. This leaves, at least for an independent molecule, vibration as the only candidate for bringing about conformational transformations. Although collisions may also be considered, my focus is on independent molecules. I tried searching for a relation between conformations and vibrations but couldn't find any relevant results.

Further Clarification

Thank you everyone who has answered the question. It is clear that vibrations do result in conformers by changing intrinsic coordinates and many computational models utilize this in conformational analysis. Qualitatively, I am able to grasp the way this occurs. However, none of sources talk explicitly about physical emergence of conformations. If, for example, I could bring about a conformational change through radiation corresponding to a normal mode, then that would answer the question in affirmative. Moreover, knowing about the mechanism by which such a change would occur would be deeply illuminating.

Answer 1

In conformational analysis, it is customary to describe a molecule by its internal coordinates, namely bond lengths, bond angles and torsion angles (or dihedral angles, with a slightly different definition compared to torsion angles).

Typically, two states of a molecule with different conformations are characterized by a difference in torsion angle. Often, there are multiple energy minima along a torsion angle coordinate. At low temperature, you can imagine the molecule being trapped in one of those (have "that conformation") while at high temperature, it might have access to multiple conformations, spending more time in the low energy ones.

Vibrations are often discussed in terms of normal modes, where all atoms move in some concerted manner. For the water molecule, we know the symmetric and asymmetric stretch (bond lengths change) and the bending mode (angles change). Water does not have a torsion angle, and you might not thing of water as having different conformations.

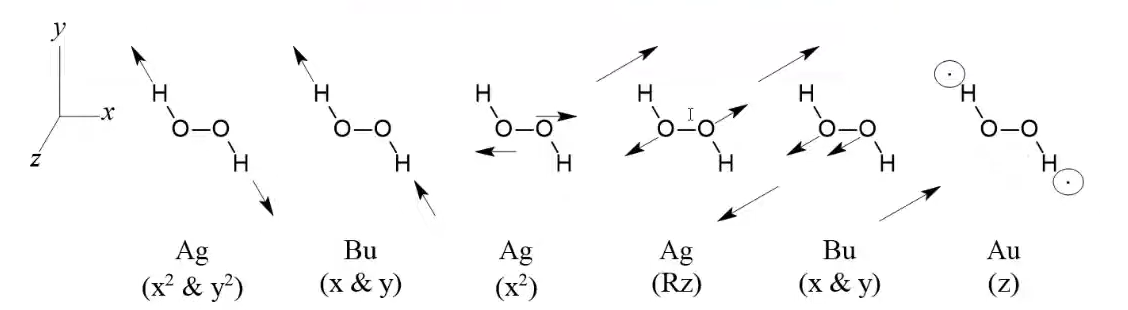
Source: PChem at Sam

You need at least 4 atoms for a torsion angle. Hydrogen peroxide (above) is a good example. Of the 3N - 6 = 6 normal modes, one is on a path that will change the torsion angle (the rightmost, labeled Au and called dihedral bend). At low temperature, hydrogen peroxide has a gauche conformation, with the torsion angle varying slightly (but staying either in the gauche+ or gauche- conformation). At higher temperatures, it can show rotation around the central bond to switch between gauche- and gauche+ via planar arrangements of the atoms.

In an isolated molecule, the energy to change conformation comes from the other normal modes through coupling (which might or might not be symmetry-permitted), like in a double pendulum.

I was wondering how a molecule transforms from one conformation to another.

In the context of normal modes, the molecule stays in the same conformation when you consider harmonic vibrations (vibrations around a single minimum). To go from one conformation to another (i.e. change at least one torsion angle substantially), you have to have sufficient activation energy to "escape" the local minimum, i.e. have to consider anharmonic vibration models.

Do conformations arise from vibrations?

In the normal mode picture, conformational change is a vibration along those modes that change torsion angles. So you could say that a gauche+ to gauche- conformational change in hydrogen peroxide is linked to the out-of-plane vibration labelled "Au" in the image above.

This leaves, at least for an independent molecule, vibration as the only candidate for bringing about conformational transformations. Although collisions may also be considered, my focus is on independent molecules.

Yes, for an isolated molecule, you would have coupled vibrations. Sometimes, the normal mode associated with a given torsion angle (or set of torsion angles) would have sufficient energy to break out of the local minimum to reach another conformation. If at that time, the energy is transferred back to the other modes, the molecule would remain in the new conformation until their is sufficient energy to break out of the new local minimum again.

Answer 2

Your broad intuition is right, conformational changes–like the flipping of a cyclohexane ring–happen because of combinations of vibrations and rotations in the molecule.

But it helps to put this in context. Even at room temperature there is a lot of thermal energy available to drive vibrations and rotations in many molecules. And that thermal energy is well distributed across many of those modes (and molecular motion of the whole molecule, molecules banging into each other is happening very fast and redistributes vibrational and rotational energy).

Flipping a cyclohexane ring (swapping equatorial for axial hydrogens or other substituents) requires coordinated changes across all the bonds in the molecule. (Remember, If the ring were "frozen" in the chair conformation, there would be two distinct types of hydrogen in a cyclohexane but at room temperature we see only one which implies the chairs flip rapidly). The lowest energy conformation is the "chair". But other conformations (eg the "boat") are also seen but have higher energy. But there is enough thermal energy at room temperature to drive the specific series of vibrations and rotations that convert chair to boat and to the opposite chair conformation.

The activation energy barrier to the flip is ~40kJ/mol which is small enough to overcome from normal thermal energy at room temperature. But it isn't just a single vibration or rotation that achieves this, it is a combination of many. Since the thermal energy is well distributed the sequence of vibrations and rotations is readily explored quickly at room temperature.

It is also worth noting that your claim that "translational and rotational motion do not change the intrinsic coordinates of a molecule and won't bring about a conformational change" is clearly not true for the cyclohexane example. The combination of bond rotations do change the spatial relationships of the atoms in the molecule. The major rotations around the C-C bonds, for example, swap the orientation of the hydrogens attached to the carbons.

Many other molecules also have enough thermal energy to cause vibrations and rotations to change their conformations at room temperature.

Answer 3

Yes, different conformations do arise from vibrations. And you've almost answered your own question. Conformations are different relative spatial arrangements of the atoms. Pure translations, rotations and electronic transitions by definition do not change the relative spatial arrangement, only vibrations can do that. Hence conformations arise from vibrations.

Now we often talk about rotating about a single bond to generate conformations. But strictly speaking that is a vibration, not a rotation, as it changes how atoms moved by that rotation are placed relative to the rest of the molecule - as chemists we often like to decouple the "interesting part" of a molecule and focus on the properties of that, similar is talking about the C=O stretch in a molecule when we really should be discussing normal modes, but in this case it leads to slightly sloppy language. That rotation is really a vibration. Hence conformations arise from vibrations.

Answer 4

A vibration is of dynamic (time dependent) nature, as such similar to a movie. On the other hand, a particular conformation is static (frozen), similar to a still frame, or single photo.

In this analogy, a vibration is a sequence of events where a molecule passes multiple conformations.