0
$\begingroup$

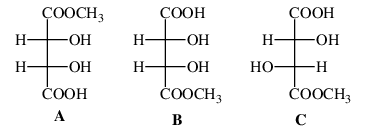
Which of the following are identical?

2,3‐dihydroxy‐4‐methoxy‐4‐oxobutanoic acid isomers

  1. A and B are identical
  2. A and B are diastereomers
  3. A and C are enantiomers
  4. A and B are enantiomers

What I know is that when we rotate a Fisher projection by 180°, then we get identical compounds (I might be wrong). But how to tell if the compounds are enantiomers of each other or not?

For that how to get the same group, i.e. $\ce{-COOH}$ in this case at the top to compare the compounds?

Also, is there any trick to tell that if they are diastereomers, enantiomers or identical by the hydroxyl group positions in Fisher projections?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ It is so because A and B are enantiomers. Tell me why. $\endgroup$
    – user55119
    Commented Nov 21, 2019 at 3:22
  • $\begingroup$ Rotating a Fischer projection by 180 deg. does not always give an identical compound. $\endgroup$
    – user55119
    Commented Nov 21, 2019 at 3:47

1 Answer 1

Reset to default
1
$\begingroup$

On rotating the compound B by 180°, we get the two -OH groups on the left side and the two H atoms on the right. In other words, B would then be an enantiomer of A.

Note: on rotating a Fischer projection by 180°, the compound remains the same; it's just that all the atoms have to be rotated, i.e. the atoms on C3 in this case would go to C2 and vice versa.

Improve this answer
$\endgroup$
6
  • $\begingroup$ So it should be one -OH group or two -OH groups on the opposite sides of 2 molecules for them to be enantiomers of each other? $\endgroup$
    – studious
    Commented Nov 21, 2019 at 15:39
  • $\begingroup$ And what about option B and C? Are they enantiomers because of the position of 1 -OH group is opposite? $\endgroup$
    – studious
    Commented Nov 21, 2019 at 15:42
  • $\begingroup$ Tech note: ˚ is a Unicode symbol that means "a ring above" (U+02DA); if you want to depict a degree symbol, then use °, "a degree sign" (U+00B0). Cherry-picking a random character that looks approximately the same as the correct one is a horrible practice. $\endgroup$
    – andselisk ♦
    Commented Nov 21, 2019 at 17:48
  • $\begingroup$ B, C and A,C form diastereomer pairs: diastereomers are isomers that are not mirror images of each other, while enantiomers are exact mirror images of each other. $\endgroup$
    – Aniruddha Deb
    Commented Nov 22, 2019 at 14:25
  • 1
    $\begingroup$ @andselisk I did not know that! alt+j on my mac gives me this character, which I always assumed was a degree sign. Thanks for letting me know. $\endgroup$
    – Aniruddha Deb
    Commented Nov 22, 2019 at 14:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.