Consider two types of reaction. One has more activation than other. If we increasing temperature by same amount in both, rate constant of which reaction will increase more?
My attempt: as we know that $k = A\mathrm e^{(-E/(RT))},$ if temperature is same in both, then the one having more activation energy will have lesser rate constant. Am I correct?
This can be answered both conceptually and mathematically.
Conceptually: Let's call the two reactions 1 and 2 with reaction 1 having the larger activation energy. Here is a plot of the arbitrary reactions.
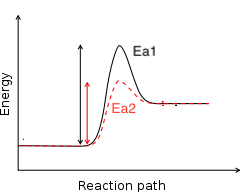
It is clear from this graph that it is "easier" to get over the potential barrier (activation energy) for reaction 2. Thus if we increase temperature, the reaction would get faster for reaction 2 before it gets faster for reaction 1. Therefore, when increasing the temperature by the same amount, the rate constant for the reaction with the lower activation energy will increase more.
Mathematically: You can understand how the rate constant changes with temperature by simply graphing. Here is a very quick and very rough graph on how the rate constant changes with temperature (plotting the equation $k=Ae^{-E/RT}$).
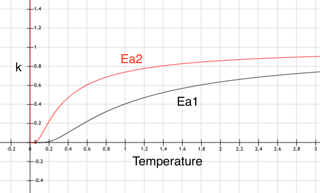
Notice how increasing the temperature causes a quicker increase in the reaction with the lower activation energy $(E_{a2})$. However, this is only an a priori expectation.
You mentioned in a comment above about differentiating. You can use that method as well. Taking the derivative of the equation for $k$ with respect to temperature while assuming $A$ and $E_a$ do not depend on temperature yields
$$\frac{dk}{dT} = \frac{AE_a}{RT^2}e^{-E_a/RT}$$
Edit: As porphyrin pointed out in the comments below, this equation elucidates the true behavior of the temperature dependence. Plotting this function for the two energies, we see that the derivative for the reaction with a smaller activation energy increases faster for lower temperatures. But for higher temperatures, there is a shift in the change of the rate constant. Therefore, the process is temperature dependent. At low temperatures, the rate constant for the reaction with low activation energy changes is more sensitive to temperature change while at high temperatures, the reaction with larger activation energy is more sensitive to temperature change.
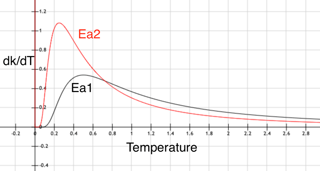
As a side note: at very large (infinite) temperatures, both functions approach the same value and there will be an equivalent distribution among the reactions' products.
From comments below: Prove that if we increase temperature in an endothermic reaction, equilibrium proceeds in forward direction.
There are a couple ways to do this. As this is now an equilibrium question, I will keep the regime to equilibrium instead of rate constants. First, let's use fundamental chemistry to get an expected result then use equations to show what the result would be quantitatively.
Fundamentals: Say you have a simple reversible reaction $$A\rightleftharpoons B$$ Given this reaction is endothermic, energy is required to drive the reaction. Because of this, we can write energy as a "reactant"
$$A + Energy \rightleftharpoons B$$
According to Le'Chatelier, if you increase temperature then the equilibrium must shift towards the products, thereby answering the question.
The Maths: Now, let's apply some math as overkill. The equilibrium constant is dependent on the standard free energy of the reaction given by the following equation
$$\Delta G^o = -RT\ln(K)$$
If we want to understand how the equilibrium constant will change we can rearrange this equation to solve for $K$ and plot as a function of temperature. Doing this and making the substitution for the free energy $\Delta G^o = \Delta H^o - T\Delta S^o$ gives
$$K = e^{-\frac{\Delta H^o}{RT} + \frac{\Delta S^o}{R}}$$
Since the reaction is endothermic, $\Delta H^o > 0$. This gives the same monotonically increasing profile that we see for the Arrhenius equation (plotted above). Therefore $K$ must always increase, and therefore the reaction proceeds in the forward direction, for increasing temperature. Of course, this is making the assumption that $\Delta H^o$ does not change with temperature.
$$k_\mathrm{high} = A e^{(−E_a/RT_\mathrm{high})}$$
$$k_\mathrm{low} = A e^{(−E_a/RT_\mathrm{low})}$$
$$\frac{k_\mathrm{high}}{k_\mathrm{low}} = e^{-E_a \cdot \beta}$$
If the activation energy is zero, the ratio of rate constants will be one, i.e. there is no temperature dependency of the rate. The higher the activation energy, the larger the exponent, the larger the ratio of the rate constants ($\beta$ is negative, so the exponent is positive).
It follows that if you are interested in the ratio of rates, it will be larger the higher the activation energy is.
The answers that come to the opposite conclusion are also correct. This is because the question is ill-defined and it is not clear what is being asked.
So we know that $$\ln\frac{k_{T_2}}{k_{T_1}} = \frac{E_\mathrm a}{R}(\frac{1}{T_1}-\frac{1}{T_2})$$ If we consider both were raised from $T_1$ to $T_2$, $$\frac{1}{T_1}-\frac{1}{T_2} = \text{constant}$$ So $$\frac{\ln\frac{k_{T_2}}{k_{T_1}}}{E_\mathrm a} = \text{constant}$$ So the one with higher $E_\mathrm a$ has a higher value for $\ln\frac{k_{T_2}}{k_{T_1}}$
Thus the fractional change of a reaction with higher $E_\mathrm a$ is more than the one with a lower $E_\mathrm a$.
The activation energy is the kinetic energy required from the reactants to form the products.Now lets go to your second part.Since the first reaction has a bigger activation energy than the second reaction , if we add energy to the system the first reaction has better chances of happening than the second reaction because more molecules from the first reaction will be converted in products than in the second case.To actually understand it you have to take quantum mechanics of every molecule in both cases and you will see it.
