I am trying to compute the ionization energy of $\ce{H2+}$ molecule ion from the electronic energy spectrum. The question is whether one should use the purely electronic Hamiltonian or the Hamiltonian with the internuclear repulsion term to do that? I illustrated two ways to compute the ionization energy below. Which one is correct?
Notice that, nuclei are not at the equilibrium distance since in my problem I can manage this distance by external forces.
I would like to clarify the matter since from OP's own answer I have a feeling he/she does not quite understand it.
So, first of all, in general the ionisation energy has to be calculated as the difference in total energy and not just the electronic one.
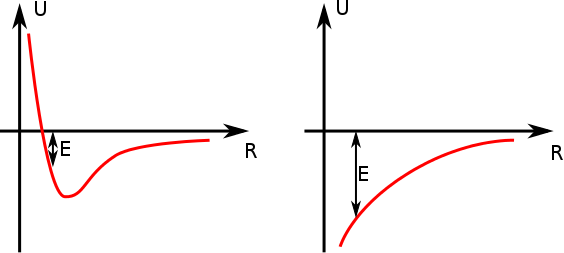
Secondly, for molecules there are two types ionization energies: adiabatic and vertical:
The adiabatic ionisation energy refers to the formation of the molecular ion in its ground vibrational state and the vertical ionization energy applies to the transition to the molecular ion without change in geometry. (From IUPAC Gold Book)
Note that in accordance with the Franck–Condon principle the most probable transition is the vertical one. Consequently, usually, when we say "ionisation energy" we actually mean the "vertical ionisation energy".
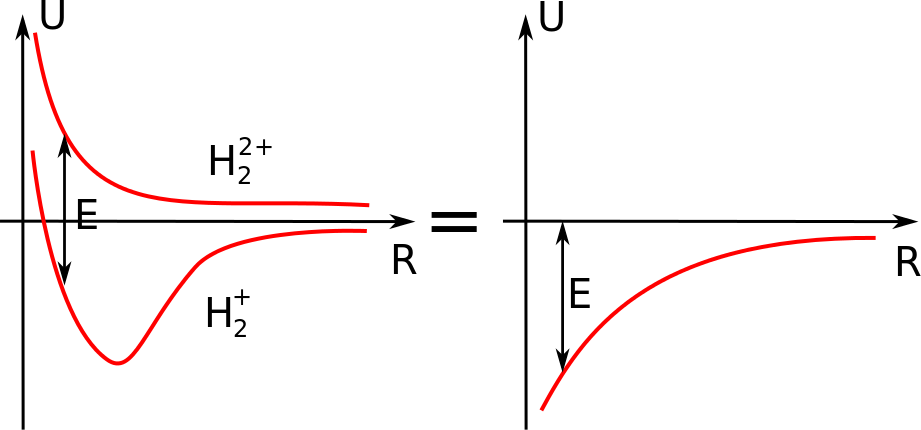
Finally, if the total energy is understood in the clamped nuclei approximation, i.e. as the electronic energy $E_{\mathrm{e}}$ together with the nuclear repulsion energy $V_{\mathrm{nn}}$, then for the vertical ionisation energy one could indeed use the difference in electronic energy rather than in total one simply because the nuclear repulsion energy stays the same. Mathematically, $V'_{\mathrm{nn}} = V_{\mathrm{nn}}$ since geometry is not changing, and thus, $$ E_{\mathrm{i}} = U' - U = (E'_{\mathrm{e}} + V'_{\mathrm{nn}}) - (E_{\mathrm{e}} + V_{\mathrm{nn}}) = E'_{\mathrm{e}} - E_{\mathrm{e}} \, , $$ where primed quantities refers to the resulting molecular specie and non-primed are that of the original molecular specie.
So, yes, for the vertical ionisation energy in the clamped nuclei approximation one can use the difference in electronic energy. And one can do so regardless of what are the molecular species in question. For the specific case of the vertical ionisation of $\ce{H2+}$ the electronic energy of the resulting $\ce{H2^2+}$ specie is obviously zero, so we specifically get what OP suggested $$ E_{\mathrm{i}} = E'_{\mathrm{e}} - E_{\mathrm{e}} = 0 - E_{\mathrm{e}} = - E_{\mathrm{e}} \, . $$ However, this is just a trivial simplification of a general formula for the vertical ionisation energy for the case of a zero-electron resulting specie.
Finally, I have found the answer. The correct way is the second one, presented in the right-hand side figure. Thus, the ionization energy is the difference between zero level and pure electronic energy. One could compute the ionization energy from the potential curve as well, but, in this case, the ionization energy is a difference between the potential energy of $\ce{H2^+}$ and $\ce{H2^{2+}}$. The potential curve for $\ce{H2^{2+}}$ just the Coulomb repulsive potential and, thus, we subtract the repulsion potential from the potential energy curve that leads us to the pure electronic energy.
