According to Wikipedia caesium’s density is $1.90\ \mathrm{g/cm^3}$ at $\Theta =20\ \mathrm{^\circ C}$. How does this change when $T$ changes? E.g. will it expand when melting?
8
-
1$\begingroup$ The only element I know that contracts on melting is iron. $\endgroup$– f pCommented Mar 27, 2013 at 12:09
-
$\begingroup$ Interesting fact. However, I wonder if there is something like a chart that plots density versus temperature. $\endgroup$– ArneCommented Mar 27, 2013 at 17:23
-
$\begingroup$ There are tables of data for elements so it should be easy to chart it. $\endgroup$– f pCommented Mar 27, 2013 at 17:29
-
1$\begingroup$ Start here $\endgroup$– f pCommented Mar 27, 2013 at 17:36
-
1$\begingroup$ And here $\endgroup$– f pCommented Mar 27, 2013 at 17:41
|
Show 3 more comments
3 Answers
$\begingroup$
$\endgroup$
Looking at the wikipedia page you can see that the density is $\rho=1.93$ kg/l at room temperature and $\rho=1.843 kg/l$ at it's melting point.
Additional information can be found in this paper, where they experimentally explore the density of liquid Cesium in the vicinity of its melting point. In the range of 302 to 375 K to be exact.
From their experimental data they deduce the following approximate equation for the density as a function of the temperature: $$\rho= 1829.12- 0.61483 \left(T-T_{melt}\right) $$ where $T_{melt}=301.6$ K.
This shows that the density reduces with temperature. Plotting the information that we have from the paper and from wikipedia yields:
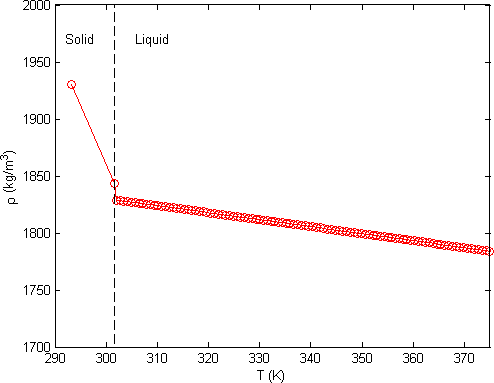
The jump at the melting point is from the mismatch between the paper and wikipedia. In the paper they specify specifically that the density is for the liquid case, on wikipedia it might (I'm not sure) be the density for the solid case at the melting point. If that is the case then the jump in density from solid to liquid is: $1843-1829=14 $kg/m$^3$
$\begingroup$
$\endgroup$
0
Since I cannot comment yet, the only other way I know to say something here is to post an answer.
To address your question about the units, the thermal expansion coefficient is a percentage. From wikipedia:
$$\alpha_L = \dfrac{1}{L}\dfrac{dL}{dT}$$
So, for a change dT of temperature, the metal will expand by a percentage of $\dfrac{dL}{L}$.
$\begingroup$
$\endgroup$
2
I think I found what I was looking for on Wikipedia. They give the thermal expansion coefficient for Caesium as $97 µm\cdot m^{−1}\cdot K^{−1}$.
-
$\begingroup$ One last thing: why is the coefficient $K^{-1}$? I would have assumed to have units $m\cdot K^{-1}$, so that I can multiply a temperature with the coefficient to get an expansion in meters. $\endgroup$– ArneCommented Mar 27, 2013 at 18:16
-
1$\begingroup$ Its dependants on the length of the piece Caesium- a longer piece of Caesium expands more. Its a standard dimensional quantity. $\endgroup$ Commented Jan 2, 2014 at 13:00