the Larmor frequencies of A and X are not the same despite being homonuclear (already called 'AX', chemical shift very different, Larmor frequency also differ), so the states should have different energy
Yes, that is precisely correct, so the two states $|\alpha\beta\rangle$ and $|\beta\alpha\rangle$ do not have the same energy. More formally, the Hamiltonian for the system (ignoring spin-spin coupling) is
$$\hat{H} = -\omega_I\hat{I}_{\!z} - \omega_S\hat{S}_z$$
where $\omega_I$ and $\omega_S$ are the Larmor frequencies of spin $I$ and $S$, and $\hat{I}_{\!z}$ and $\hat{S}_z$ are theie corresponding spin angular momentum operators, with the following properties:
\begin{align}
\hat{I}_{\!z} |\alpha\rangle &= \frac{\hbar}{2}|\alpha\rangle \\
\hat{I}_{\!z} |\beta\rangle &= -\frac{\hbar}{2}|\alpha\rangle
\end{align}
The energies of the states $|\alpha\alpha\rangle$, $|\alpha\beta\rangle$, $|\beta\alpha\rangle$, and $|\beta\beta\rangle$ can be evaluated fairly simply using
$$E = \langle \Psi | \hat{H} | \Psi \rangle$$
or in this case, since all of them are eigenstates of the Hamiltonian (without coupling), then
$$\hat{H}\Psi = E\Psi$$
and it suffices to read off the eigenvalue from the right-hand side. Proceeding in this manner, we have
\begin{align}
\hat{H}|\alpha\beta\rangle &= -\omega_I \hat{I}_{\!z}|\alpha\beta\rangle - \omega_S \hat{S}_z|\alpha\beta\rangle \\
&= -\omega_I (\hbar/2)|\alpha\beta\rangle - \omega_S (-\hbar/2)|\alpha\beta\rangle \\
&= [\hbar(\omega_S - \omega_I)/2] |\alpha\beta\rangle \\
\Longrightarrow E_{\alpha\beta} &= \hbar(\omega_S - \omega_I)/2
\end{align}
Likewise,
\begin{align}
\hat{H}|\beta\alpha\rangle &= [\hbar(\omega_I - \omega_S)/2] |\beta\alpha\rangle \\
\Longrightarrow E_{\beta\alpha} &= \hbar(\omega_I - \omega_S)/2
\end{align}
and in general, since $\omega_I \neq \omega_S$, these energies are different. The introduction of spin–spin coupling does not affect these conclusions.
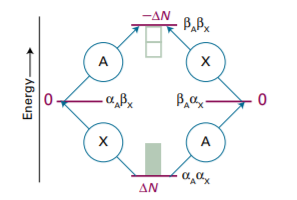
One question remains, which is: why does Atkins depict the states as having the same energy, despite them not being equal? One possible answer is laziness, but if you read their writings, Atkins (and Keeler) are quite particular about notation and technical correctness, so I feel like this is not the primary reason, or maybe I am more inclined to give the benefit of the doubt here.
A more convincing answer would be that the difference between the energies $E_{\alpha\beta}$ and $E_{\beta\alpha}$ is very small:
$$E_{\alpha\beta} - E_{\beta\alpha} = \hbar(\omega_S - \omega_I)$$
The difference in Larmor frequencies between spins, i.e. $\omega_S - \omega_I$, is generally on the order of Hz to kHz. On the other hand, the other energy differences on the graph are far larger:
$$E_{\beta\beta} - E_{\beta\alpha} = \hbar\omega_S$$
The Larmor frequency itself $\omega_S$ is on the order of MHz. So the energy difference between $\alpha\beta$ and $\beta\alpha$ is pretty negligible compared to the other energy differences shown in the diagram.