OK, let's step through your questions one at a time.
First off, what do they mean by steady-state number here?
Intermediates formed in reactions usually exist in low concentration. The steady-state assumption assumes that the concentration of this (low-concentration) intermediate will not change appreciably over the course of the reaction once steady state has been established. The rate of its formation is assumed to be equal to the rate of its consumption. Making the steady state assumption allows us to recast the previous statement as a mathematical equation as we'll see below.
In this case why must the rate of absorption be equal to the rate of desorption
Just like in a chemical equilibrium where it takes a finite time for the equilibrium to establish itself, it takes a finite time before the steady state is established. Once there is a finite, non-zero concentration of the intermediate and we have arrived at the steady state, then the rate of absorption and desorption will be equal and the non-zero concentration of the intermediate(s) will remain constant – a steady state.
Applying the steady-state assumption to the problem at hand allows us to state that for all of the intermediates (dust particles with 1 or 2 hydrogen atoms on them), the rate of hydrogen atom adsorption on an intermediate must be equal to the rate of hydrogen atom desorption from that intermediate. This can be written as
$$k_\mathrm a [\ce{H}] = k_\mathrm d N$$
and then rearranged and solved:
$$\begin{align}
N &= \frac{k_\mathrm a}{k_\mathrm d}[\ce{H}] \\
&= \frac{\pu{1.4 * 10^-5 cm3 s-1}}{\pu{1.9 * 10^-3 s-1}} \cdot \pu{10 cm^-3} \\
&= \pu{7.4 * 10^-2}
\end{align}$$
Why is it $k_\mathrm d N$?
Because $k_\mathrm d N$ represents the total rate of desorption of hydrogen atoms from dust particles, the right side of our verbal equation. It represents desorption from $x_1$ and $x_2$ - the only two species that can desorb, and it is equal to
$$k_\mathrm d N = k_\mathrm d x_1 + 2k_\mathrm d x_2$$
the factor of "2" arising because there are 2 hydrogen atoms on each dust particle in $x_2$, and both are viable candidates for desorption.
Further, why is the rate of desorption not simply the reverse of the absorption reaction and thus the same rate but with a negative sign?
It is the same rate, that's what $k_\mathrm a [\ce{H}] = k_\mathrm d N$ means. Rates are always a positive number, we are multiplying a rate constant times a concentration, both positive numbers. However, when we write an equation and insert the rate expression into it, we can insert a minus sign before the rate expression if the rate expression represents a pathway that is causing the concentration of a product, reactant or intermediate to decrease.
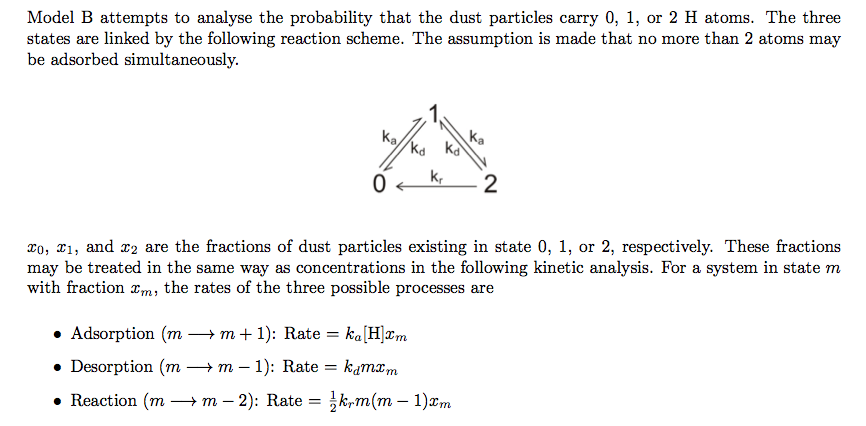
How did they come up with those rates?
- Adsorption rate: $r_\mathrm a = \sum_m k_\mathrm a [\ce{H}] x_m = k_\mathrm a [\ce{H}]x_0 + k_\mathrm a [\ce{H}]x_1$
This is just the typical kinetic expression – adsorption rate constant multiplied by the concentration of hydrogen atoms available to adsorb times the concentration of the various dust particles that can adsorb a hydrogen atom, which are only $x_0$ and $x_1$.
- Desorption rate: $r_\mathrm d = \sum_m k_\mathrm d m x_m = (k_\mathrm d \cdot 0 \cdot x_0) + (k_\mathrm d \cdot 1 \cdot x_1) + (k_\mathrm d \cdot 2 \cdot x_2)$
Here $m$ is a concentration term analogous to $[\ce{H}]$. It represents the number of hydrogen atoms on a dust particle, and can be 0, 1 or 2. Note how it "removes" $x_0$ from the desorption equation as it should since a particle with no hydrogen atoms cannot desorb. Notice too how it adjusts $x_2$ for the fact that there are two adsorbed hydrogen atoms in this case, so desorption is statistically twice as likely to happen. It differs from $[\ce{H}]$ in its units: $[\ce{H}]$ is in $\pu{atoms/cm3}$ while "m" is in units of atoms/dust particle.
- Reaction rate: $r_\mathrm r = \left(\frac{1}{2} \cdot k_\mathrm r \cdot 2 \cdot 1 \cdot x_2\right) \left(\frac{1}{2} \cdot k_\mathrm r \cdot 1 \cdot 0 \cdot x_1\right) = k_\mathrm r x_2$
EDIT 1:
Also, after your explanation I understood how they got the absorption and desorption rates, but I'm still confused on how they got the reaction rate $\left(\frac{1}{2} \cdot k_\mathrm r \cdot 2 \cdot 1 \cdot x_2\right) \left(\frac{1}{2} \cdot k_\mathrm r \cdot 1 \cdot 0 \cdot x_1\right)$. Could you please elaborate further on where all the numbers came from?
Note we have a factor of $1/2$ because it takes 2 hydrogen atoms to produce 1 hydrogen molecule. The $2$ and the $1$ come from the concentration terms $m(m-1)$. In the "Reaction" case we have to combine two hydrogen atoms. It is a bimolecular reaction and just like in the bimolecular reaction $\ce{A + B -> C}$ where the rate is proportional to $[\ce{A}][\ce{B}]$, here the analogous concentrations are $m(m-1)$. Initially the concentration is $m=2$, but after that first hydrogen atom is "selected" the remaining concentration from which to pick our second hydrogen atom has been decreased to $m-1$. What we're saying is that when we pick the first hydrogen we have two atoms to choose from, when we pick the second hydrogen atom we have one atom to choose from. If we had 5 hydrogen atoms on a particle and were still going to make 2 picks the equation for our two picks would become $5(5-1)=20$. We would expect this case to proceed $(20/2)=10$ times faster than the case with only 2 atoms on the dust particle.
There are 2 ways to form $\ce{H2}$ in our problem. Label the hydrogen atoms A and B, then we can either pick via an AB or BA sequence. $m(m-1)$ is exactly analogous to $[\ce{H}][\ce{H}]$, it's just not a square because there is a significant difference between $2\cdot 2$ and $2\cdot 1$. When dealing with a mole of atoms ($N = 6 \cdot 10^{23}$, or Avogadro's number) and they react with one another in a bimolecular reaction, there is no need to write $(6\cdot 10^{23})(6\cdot 10^{23} - 1)$, but in our case with such small numbers the difference matters.
Why does absorption have $[\ce{H}]x_m$ but desorption have $mx_m$?
As noted above, $m$ is a concentration term analogous to $[\ce{H}]$, but with different units.
Finally, for the reaction rate, where did they get $m(m−1)$?
See above.
why does the $k_\mathrm a$ term have $[\ce{H}]$ while the other terms do not?
The other terms use $m$ which, as explained above, is analogous to $[\ce{H}]$ in that they both reflect concentrations, $[\ce{H}]$ for hydrogen atoms in space and $m$ for hydrogen atoms on dust particles; they use different units as explained above.
What happened to the $mx_m$ that was mentioned above?
Since the full rate expressions have been written out, they have been replaced with integers, 0, 1, or 2, representing the concentration of hydrogen atoms on a dust particle.
Further, how did they derive the rate of change for $x_1$ and $x_2$?
The rate of change of $x_1$ is the rate of formation minus the rate of destruction.
The rate of formation is given by $k_\mathrm a[\ce{H}]x_0$ (the rate of a bare dust particle picking up a hydrogen atom) plus $2k_\mathrm d x_2$ (the rate of a dust particle with two hydrogen atoms losing one).
The rate of destruction is given by $k_\mathrm a[\ce{H}]x_1 + k_\mathrm d x_1$ (the rate of a dust particle with one hydrogen atom either picking up one more, or losing it).
For the time being, I'll leave the rate of change of $x_2$ as an exercise for you to test yourself on what you've learned.