The vapor pressure of water at $\pu{90 ^\circ C}$ is $\pu{525.8 torr}$, which is $\frac{\pu{1 atm}}{\pu{760 torr}} \times \pu{525.8 torr} \approx \pu{0.69 atm}$. Thus, it is true that water boils at $\pu{90 ^\circ C}$ at altitude with $\pu{0.69 atm}$ pressure. However, taking $\pu{300 min}$ to boil an egg in same altitude is another story (think of people in Bhutan where average altitude is $\pu{3.3 km}$! How long it would take them to cook their lunch?).
Since it said in the question, we don't have a choice but go for it. However, since no additional data is given, we have to assume the edd boils linearly. That means at time zero, there would be 100% row egg and 0% boiled egg in both situation and at $\pu{100 ^\circ C}$, 0% row egg and 100% boiled egg would be remaining after $\pu{3 min}$. Therefore, the average rate of an egg boiling at $\pu{100 ^\circ C}$ is $\frac{100\%}{3} \ \pu{sec-1}$. Similarly, average rate of an egg boiling at $\pu{90 ^\circ C}$ would be $\frac{100\%}{300} \ \pu{sec-1}$. Assuming linear boiling, I plotted the percent boiling versus time in both cases:
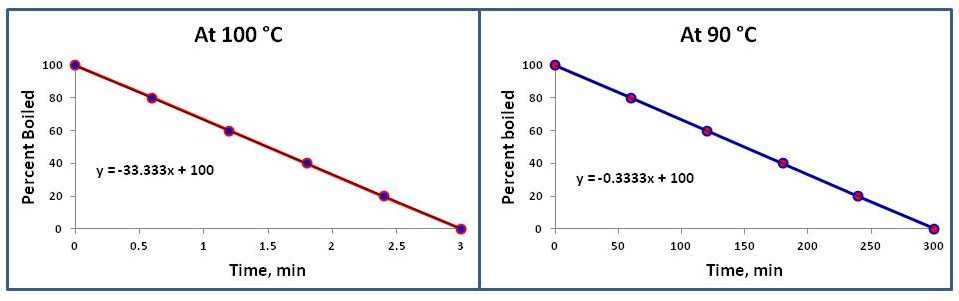
These plots show that the rate constant $k$ is same as the rate of boiling. Thus:
$$\frac{k_\pu{100 ^\circ C}}{k_\pu{90 ^\circ C}} = \frac{\frac{100}{3}}{\frac{100}{300}} = 100$$
If you want to use the slope of plots (which are equal to corresponding rate constants):
$$\frac{k_\pu{100 ^\circ C}}{k_\pu{90 ^\circ C}} = \frac{33.333}{0.333} = 100$$

