0
$\begingroup$

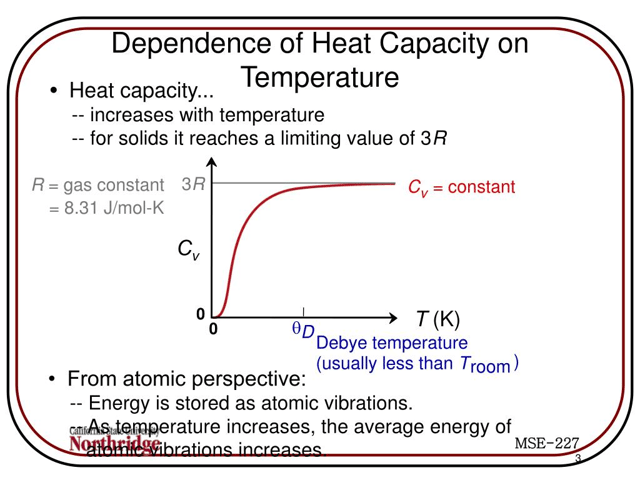
I was studying about the graph of dependence of heat capacity on temperature.

enter image description here

As you can see in this picture, heat capacity with constant volume increases near 0K. As the temperature keeps rising, it converges to 3R.

I was finding out why these changes happen. In the textbook, the author explained like this.

For the increasing part,

This corresponds to an increased ability of the lattice waves to enhance their average energy with increasing temperature.

And, for the converging part,

The quantity of energy required to produce a 1-degree temperature change is constant.

However, I could not understand about the reason of converging part.

I think the energy required to produce a 1-degree temperature change can be constant, but how this can be only applied only for converging part?

Improve this question
$\endgroup$
2
  • $\begingroup$ That's the definition of converging: it approaches and then reaches (matches) a determined value. To understand this you need to study statistical mechanics, which provides theories that explain the changes in $C_V$ with temperature. $\endgroup$
    – Buck Thorn ♦
    Commented Jun 13, 2020 at 2:04
  • $\begingroup$ The heat capacity is the slope of internal energy with temperature ($C_V=\partial U/\partial T|_V$ ) so as more energy is added by increasing temperature the rate at which this increases eventually becomes constant (as energy levels now v numerous) and hence the $C_V$ is constant. Similarly at v low $T$ v few energy levels can be populated and $U$ is almost constant, as they become populated $U$ increases and so does $C_V$. $\endgroup$
    – porphyrin
    Commented Jun 13, 2020 at 7:20

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.