Dependence of the rate of a catalysed reaction on substrate concentration?

Question

In my answer to this question: Master equation for catalyst and substrate binding?. I made the assumption along the following lines:

If we have a substrate concentration $[S]$ then the rate of binding of any substrate molecule with a given enzyme is proportional to $[S]$. Such that $$\text{rate}=\alpha [S]$$ I have however, read that it is rather a hyperbolic relation. So are there any (and if so what) assumptions required so that we can assume that the rate is proportional to $[S]$ rather then it been a hyperbolic relation?

Answer 1

There is always a maximum convertion rate $V_{max}$ for every given enzyme-substrate system. In some cases the substrate itself can inhibit the enzyme's activity at high concentrations, because of the formation of some ESS-complexes, which can not dissociate into product and enzyme.

The frequent correlation is described by the Michaelis-Menten kinetics, which introduces the Michaelis constant $K_M$ as the substrate concentration at which half of all enzyme is bound to substrate $-$ and thus the reaction is running at a speed of $\frac{V_{max}}{2}$.

As you can see, the relation is described by a saturation function. But for loose approximations, where $[S] \leq K_M$ you could say its roughly proportional: $$rate = \dfrac{V_{max}}{2 \times K_M} \times [S]$$

Answer 2

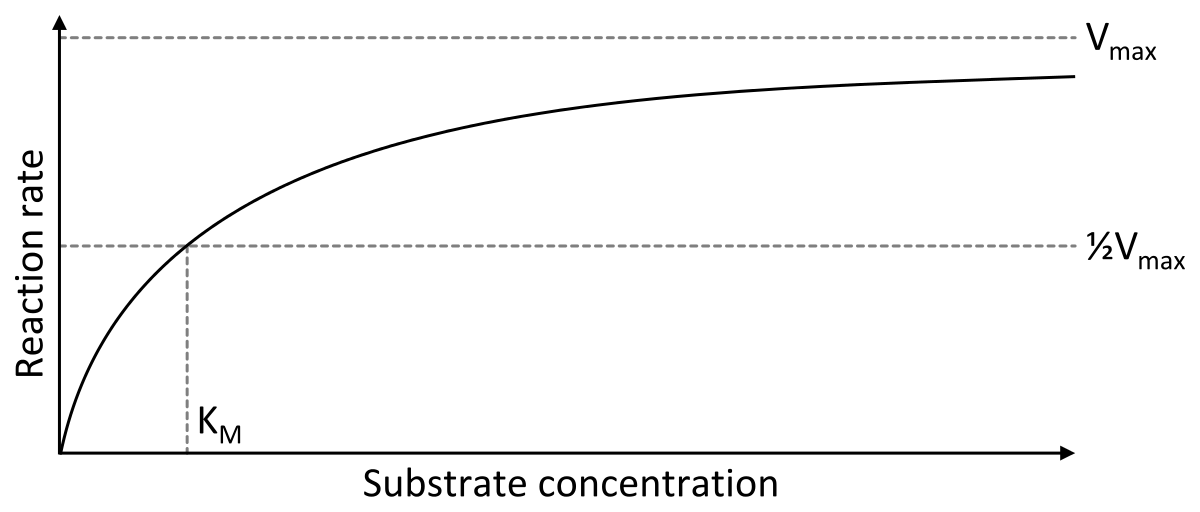
The curve is not hyperbolic. A hyperbolic curve is a result of an equation $f(x)=\dfrac{b}{a}\sqrt{x^2-a^2}$. Which is not the case here.

The enzyme catalysis (Michaelis-Menten model) can be described by the two step reaction.

$$\ce{E + S<=>[k_f][k_r] ES ->[k_{cat}] E + P}$$

In Michaelis-Menten kinetics you make either of the two approximations:

• Equilibrium approximation: assuming that first reaction (i.e. enzyme-substrate binding) is in equilibrium. Therefore $k_r[ES]=k_f[E][S]$

• Quasi-steady-state approximation (QSS): assuming that the concentration of $ES$ complex remains constant over time. Therefore $k_f[E][S]=(k_r+k_{cat})[ES]$

For both these cases you can substitute $[ES]$ with $[E_0]-[E]$ where $E_0$ is the total enzyme concentration. You can also represent $[E]$ in terms of $[S]$ using any of the above relationship. Then, you assume that maximum catalytic activity would be when all the available enzyme is complexed with the substrate ($[ES]=[E_0]$). In that case the maximum rate of catalysis $V_{max}$ would be $k_{cat}[E_0]$. When you put everything together then the rate of catalysis would turn out to be:

$$\frac{d[P]}{dt}=V_{max}\frac{[S]}{K_M+[S]}$$

Where:

• $K_M=\dfrac{k_r}{k_f}$ for equilibrium approximation

• $K_M=\dfrac{k_r +k_{cat}}{k_f}$ for QSS approximation

These kind of curves denote saturation kinetics and can also be seen in case of adsorption.