Can't silver have 61 neutrons? [closed]

Question

What happens if silver does have 61 neutrons?

Answer 1

The concerned silver nuclide with 61 neutrons is $\ce{^108_47Ag}$. This nuclide with 47 protons and 61 neutrons lies in the so-called valley of β-stability.

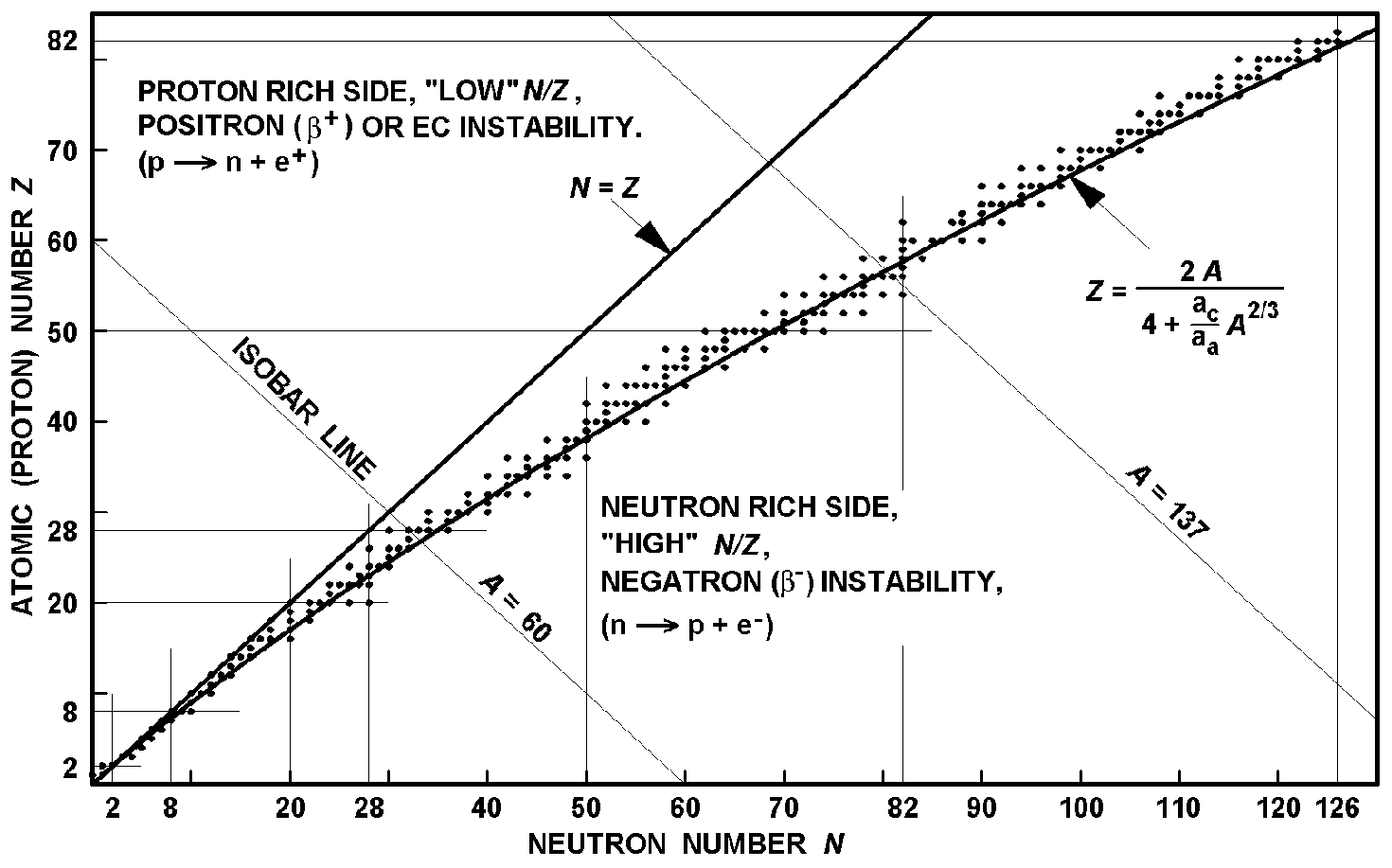
^{Image taken from Choppin, Liljenzin, Rydberg: Radiochemistry and Nuclear Chemistry, third edition (2002), p. 42}

Nuclides on right side of the valley (higher neutron numbers) are unstable to decay by β⁻ emission. Nuclides on the left side are unstable to β⁺ decay or electron capture.

Generally, for isobars (i.e. nuclides with a constant mass number $A$), the binding energy $E$ as a function of the atomic number $Z$ describes a parabola:

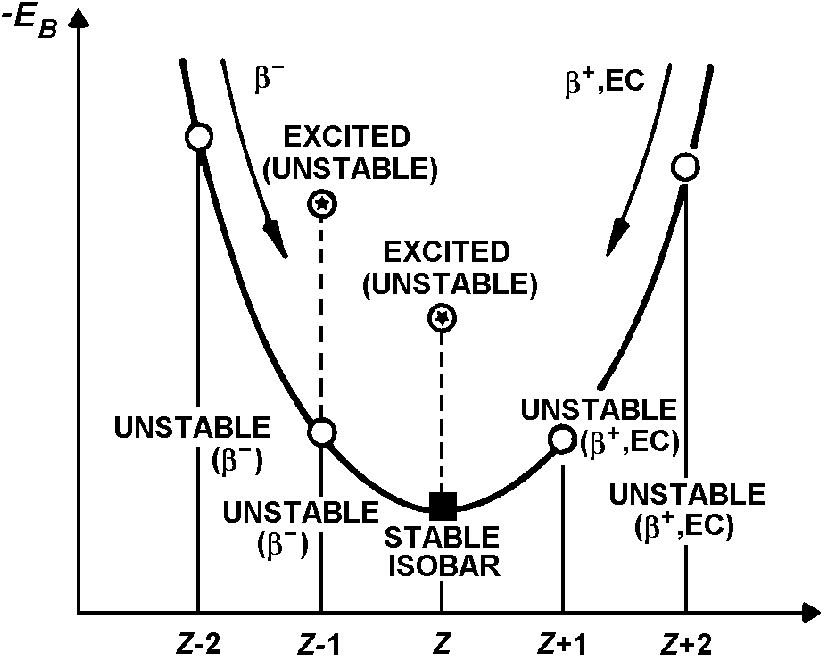
^{Image taken from Choppin, Liljenzin, Rydberg: Radiochemistry and Nuclear Chemistry, third edition (2002), p. 52}

The most stable nuclides lie at the bottom of the valley (but not necessarily exactly at the minimum of the parabola).

This diagram is correct for nuclides with odd mass number $A$ (i.e. with even $Z$ and odd $N$, or with odd $Z$ and even $N$). For nuclides with even $A$, however, two different parabolas exist, depending on whether the nuclides have even $Z$ and even $N$ or odd $Z$ and odd $N$:

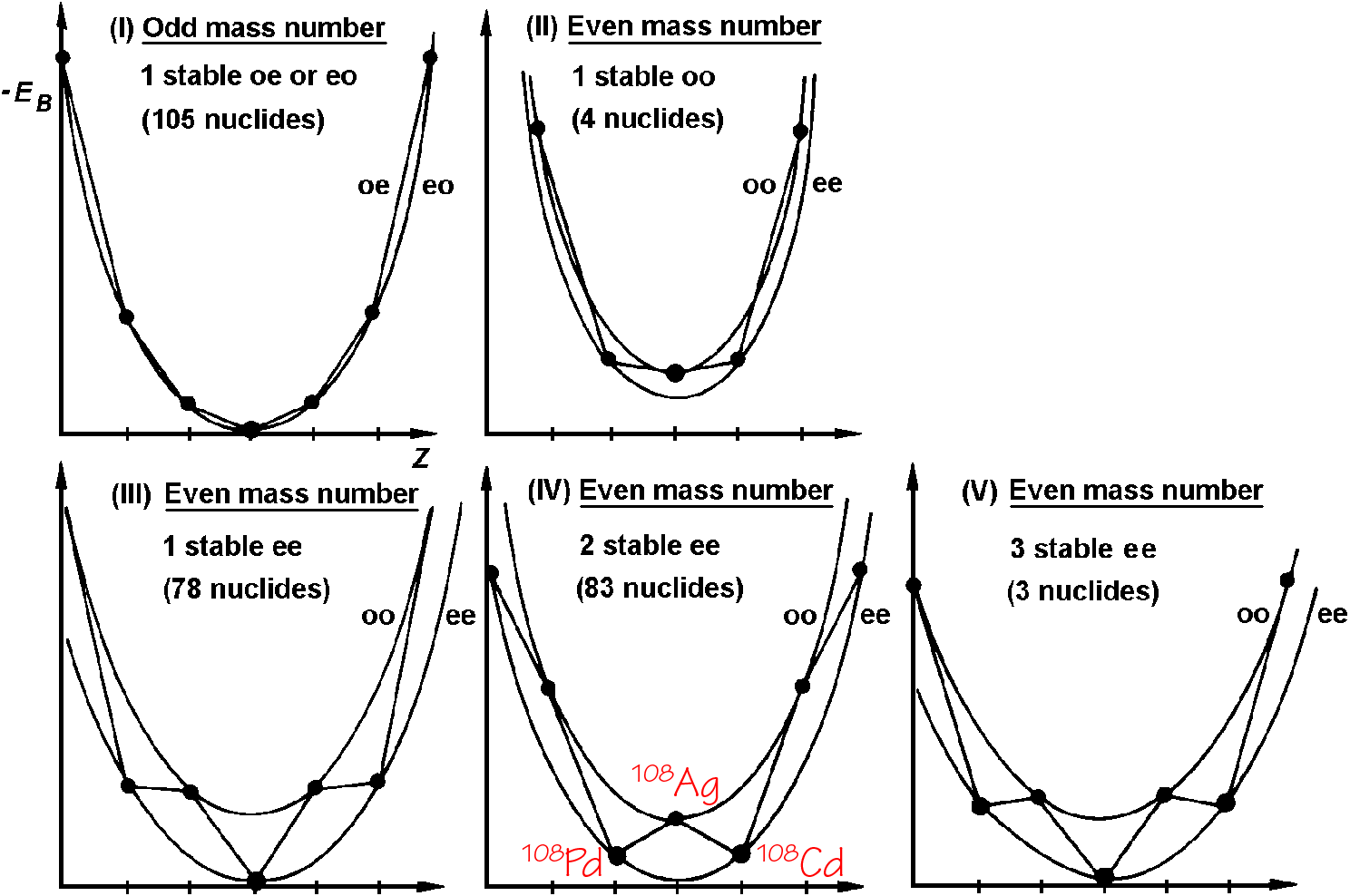
^{Image adapted from Choppin, Liljenzin, Rydberg: Radiochemistry and Nuclear Chemistry, third edition (2002), p. 52}

Nuclides with even $Z$ and even $N$ (ee) are more stable (i.e. have a larger nucleon binding energy) than nuclides with odd $Z$ and odd $N$ (oo).

The case (IV) with two parabolas also applies to the question. The stable nuclide $\ce{^108_46Pd}$ has 46 protons and 62 neutrons (even-even). The nuclide considered in the question, $\ce{^108_47Ag}$, has 47 protons and 61 neutrons (odd-odd). The next neighbour, $\ce{^108_47Cd}$ has 48 protons and 60 neutrons (even-even) and is stable.

Therefore, $\ce{^108_47Ag}$ is unstable to β⁻ decay to $\ce{^108_48Cd}$ $(97.65\ \%)$ as well as to β⁺ decay to $\ce{^108_46Pd}$ $(2.35\ \%)$.

Since $\ce{^108_47Ag}$ is radioactive with a short half-life of about $2.37\ \mathrm{min}$, it doesn’t exist in nature (except as an activation product in trace amounts).