The first thing to remember is that the "shell" model is an over-simplification of quantum chemistry. A better picture is that the electrons represent "clouds" (i.e., electron density).
Your question basically boils down to "why don't 5th and 6th row atoms get much larger?"
Quantum mechanics can give a relatively easy formula for the "average" radius of a given hydrogen orbital
$$\langle r \rangle = a_0 n^2 \left( 1 + \frac{1}{2} \left[ 1- \frac{l(l+1)}{n^2} \right] \right)$$
Here, $a_0$ is the Bohr radius, the expected radius of the hydrogen atom, and $n$ is the principal quantum number and $l$ is the angular quantum number (e.g., l=0 for "s" orbitals and n=5 and 6 for Rb and Cs).
It's pretty obvious that as n gets large for s orbitals (l=0), that formula approximates:
$$\langle r \rangle \approx \frac{3 a_0}{2} n^2$$
(The left side becomes smaller and smaller, so the coefficient gets closer and closer to 3/2.)
So for a hydrogen atom (one electron), the 5s and 6s orbitals in fact get very large.
Your question is about atoms. So we have to consider many electrons. Basically, this is another representation of the lanthanide contractionlanthanide contraction caused by relativistic effectsrelativistic effects.
Indeed, in that last link, there's a formula for the atomic radius after relativistic corrections.
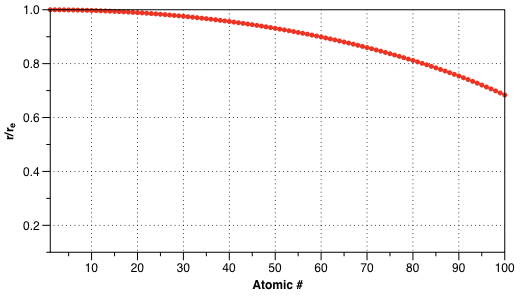
For atomic numbers 11 and 19, the relativistic correction is 0.99 (i.e., no real change to the radius). For atomic numbers 37 and 55, it's 0.963 and 0.916, respectively. So ~9% of the "ideal" radius is lost with $\ce{Cs}$.
