I have a software in my android device named hydrogen atom. It show orbital of hydrogen we have to set the quantum numbers and probability of finding particle. But what the blue and red colour area in below pictures represent.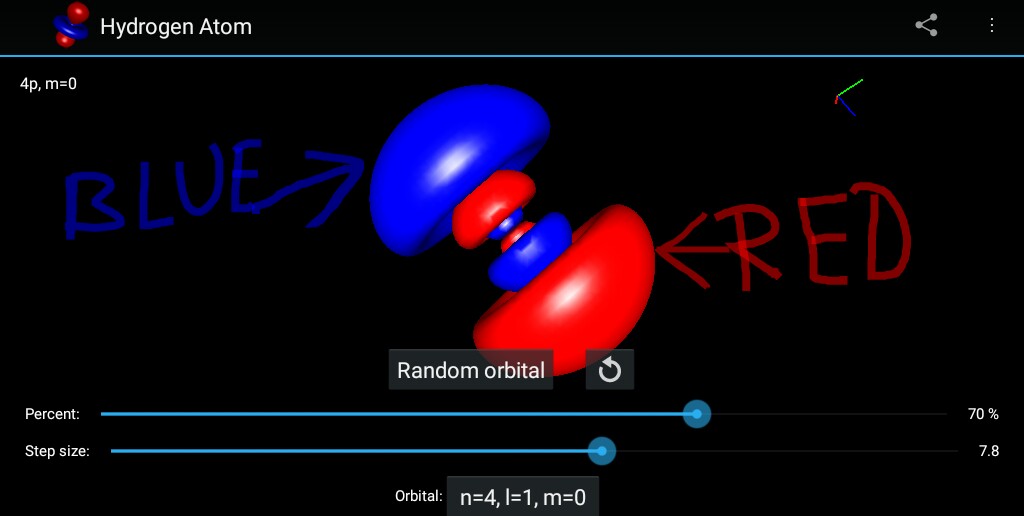
1 Answer
To answer this question, we need to start from the beginning.
Movement of an electron is described by wave function. The best layman description for it is that wave function is a quantum equivalent of trajectory. It isn't exactly trajectory, but it serves as equivalent for quantum mechanics.
A trajectory repeating itself and wrapped around an object is called an orbit. A quantum mechanical equivalent is a wave function, remaining constant in time. Such a function for hydrogen atom is called orbital.
Wave function is a function. It means, that it can be positive and negative. (Well, in case of a stationary function, see below). It usually doesn't matter in simple chemistry, but once we start talking about orbital interactions it becomes important. In short, orbitals interact with formation of orbitals with reduced energy as long as lobes with same sign overlap. Thus, it is important to know sign of the wave function in lobes of the orbitals.
So, whenever you see an orbital depicted using two colors, it means two different signs of the wave function. Note, since math, it is not important which color depicts what sign. If one inverses sign of all lobes simultaneously, the result will depict the same system, actually.
Side note 1: the surfaces depicted in the picture likely are isosurfaces (i.e. the function in consideration has same value in all surface points) of square of wave function that enclose volume containing some fixed amount of electron density (usually somewhere around 90-95%). There is no good way to depict wave function/orbital in 3 dimensions really.
Side note 2: wave function has well defined sign only in case of stationary system (i.e. unchanged with time). Whenever we talk about system evolving in time, we need to consider arcane math of complex numbers.
-
3$\begingroup$ and to add a little bit extra to your clear answer; sometimes the plus and minus are called 'parity' or 'phase'. This is important when bonding: in phase is bonding and out of phase is anti-bonding, most easily imagined with $\pi$ orbitals. $\endgroup$ Commented Apr 27, 2018 at 13:14
