4
$\begingroup$

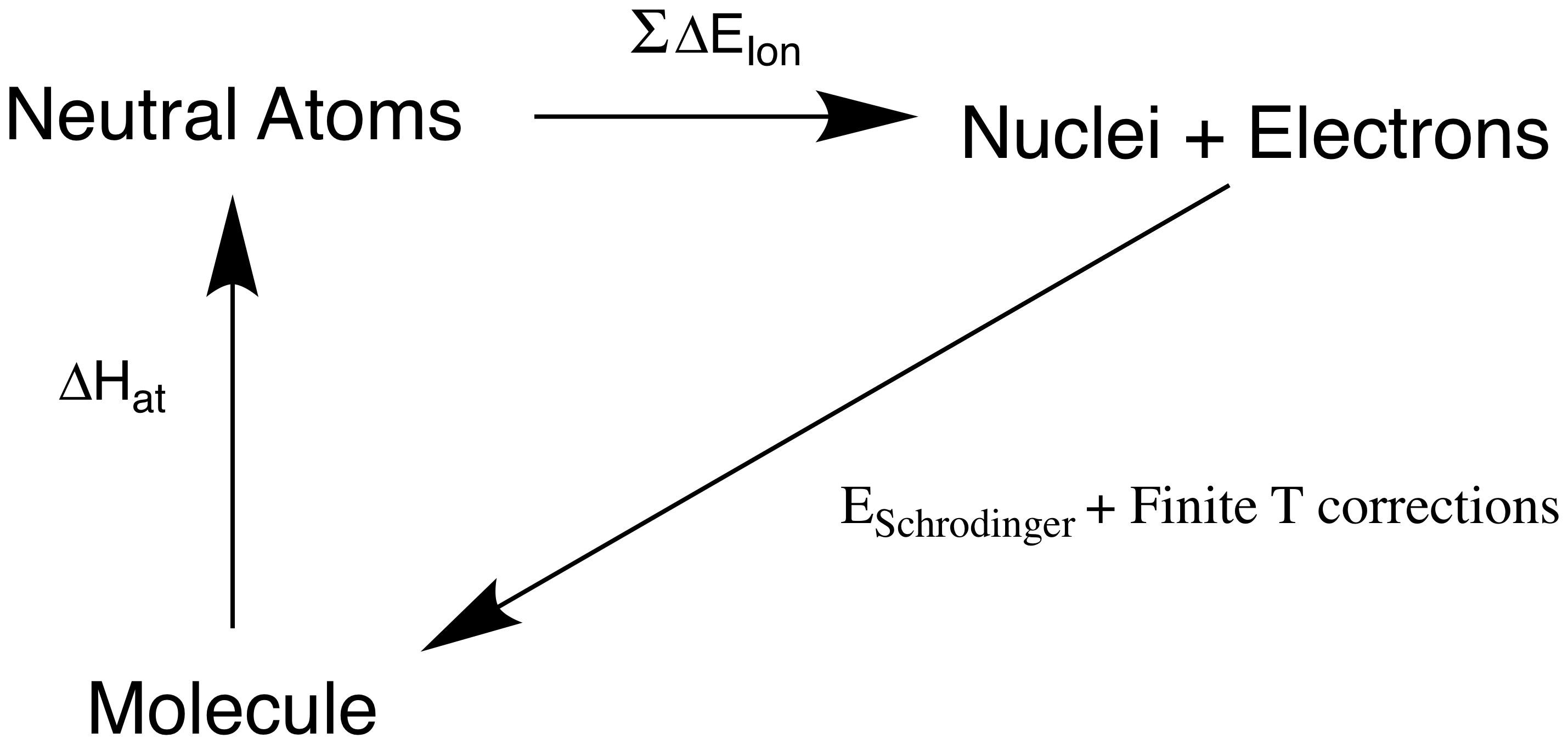
Atomization Energy ΔHat is the energy required to disintegrate a molecule into isolated atoms that are infinitely away from each other. The question is about atomization energy and its relation to the total energy (E) in Schrodinger equation (Eψ=Hψ).

If we disintegrate a molecule one step further to isolated electrons and nuclei, will the overall energy spent be equal to negative of the total energy of Schrodinger equation? I am assuming an energy reference will come into play as well as thermal energy. So let's say we are conducting the thought experiment of disintegration at 0 K (zero Kelvin) and we correct the values by assuming a reference energy point. Then, is the total Energy E equal to disintegration energy of the molecule into isolated electrons and nuclei?

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ You can easily test that when looking at a H atom. Calculate the energy on different distances between proton and electron. You can then extrapolate your findings to bigger atoms and molecules. Of course, you need to use an approximated Hamiltonian, but eh, for a basic discussion it's alright. $\endgroup$
    – user37142
    Commented Jul 13, 2017 at 5:16
  • 2
    $\begingroup$ The interaction between the isolated particles would vanish, therefore $\langle \hat V_{ij}\rangle=0$, but you still have the contributions from the kinetic energy $\langle \hat T_i\rangle$ of each individual particle. $\endgroup$
    – Feodoran
    Commented Jul 13, 2017 at 8:33
  • $\begingroup$ @Feodoran Makes sense. I also think that all identical nuclei when isolated have the same kinetic energy and all electrons when isolated have the same kinetic energy as well. So at least for two isomers it should be taken care of, should it not? In other words, the total disintegration energy difference between two isomers is equal to total (SE) energy difference. Is that about right? $\endgroup$
    – Kinformationist
    Commented Jul 13, 2017 at 14:08
  • $\begingroup$ @Fl.pf. Right this can be tested through actual calculations. I was trying to get an answer through conceptual thinking without doing the actual calculations. $\endgroup$
    – Kinformationist
    Commented Jul 13, 2017 at 14:10
  • $\begingroup$ @Kinformationist proof of concept assisted by calculation? $\endgroup$
    – user37142
    Commented Jul 13, 2017 at 14:52

1 Answer 1

Reset to default
2
$\begingroup$

If we disintegrate a molecule one step further to isolated electrons and nuclei, will the overall energy spent be equal to negative of total energy of schrodinger equation?

By convention, yes.

Energy as a absolute quantity is not well-defined, energy must be defined with respect to an energy zero. By convention, when we perform quantum chemical calculations our energy zero is free electrons and nuclei infinitely far apart, which we define to have zero potential energy as they cannot interact.

Also, quantum mechanically, we consider only the case at $0$ K. We can however add on corrections to the free energy due to entropy and internal motion at finite temperatures, as well as vibration zero point energies.

How is this different from the atomisation energy?

We can see that to get from the products of atomisation to the products of "disintegration" (as you refered to it), is to remove all the electrons from the neutral atoms. That energy is given by the sums of all the ionisation energies for each atom in the system, removing each electron one by one. This allows us to construct a Hess cycle.

Finite temperature corrections are added to the quantum mechanical energies as the potential energy and free energy are different at finite temperature.

Hess Cycle

Improve this answer
$\endgroup$
4
  • $\begingroup$ What do you mean by finite T corrections. Also E (SE) plus Nuclei and electrons leads to the Molecule. E(SE) includes individual kinetic contributions of Nuclei and electrons. What you wrote on the scheme is probably only the potential energy part of the E (SE). $\endgroup$
    – Kinformationist
    Commented Jul 27, 2017 at 22:14
  • $\begingroup$ When the electrons are bound to nuclei they have finite kinetic energy that is accounted for within the Electronic Schrodinger equation, and presuming you include nuclear kinetic energy it will include the vibrational zero point energy of your nuclei. So that kinetic energy is included in an ordinary Schrodinger equation energy. However that is all calculated to $0$ K. $\endgroup$
    – user213305
    Commented Jul 28, 2017 at 0:15
  • $\begingroup$ As such that doesn't include the effects of entropy. At finite temperature, multiple vibrational states will be accessible to the molecules, some of which will be occupied. This introduces vibrational entropy; rotational, translational and in extreme cases electronic entropy can also be introduced. This changes the free energy of the molecule as $G = U + PV - TS = H - TS$. However as you can see above my diagram used enthalpy $H$ rather than free energy $G$ so this corrections aren't required to complete the cycle as you point out. $\endgroup$
    – user213305
    Commented Jul 28, 2017 at 0:21
  • $\begingroup$ Finally, free energy due to entropy and (temperature in general) is not relevant to the case of a single molecule whose vibrational and rotational state we can measure/know. It is only relevant to and ensemble of many molecules at a given temperature where different numbers are in different rovibrational states. I'll try and clear up the main answer when I have a bit more time. $\endgroup$
    – user213305
    Commented Jul 28, 2017 at 0:24

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.