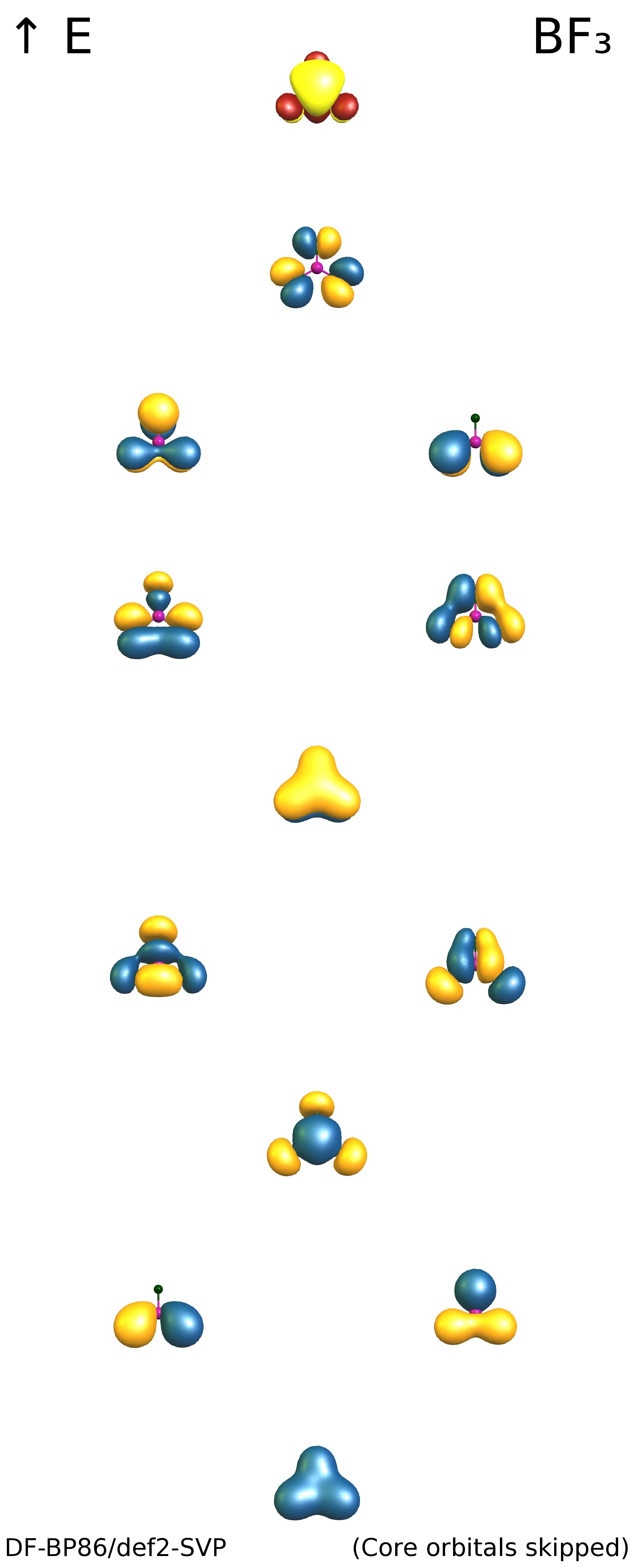
Here is the representative section from the natural bond orbital analysis:
(Occupancy) Bond orbital / Coefficients / Hybrids
------------------ Lewis ------------------------------------------------------
(core and symmetry equivalent orbitals skipped)
5. (1.99345) LP ( 1) F 2 s( 63.79%)p 0.57( 36.19%)d 0.00( 0.01%)
6. (1.94124) LP ( 2) F 2 s( 0.00%)p 1.00( 99.95%)d 0.00( 0.05%)
7. (1.86896) LP ( 3) F 2 s( 0.00%)p 1.00( 99.94%)d 0.00( 0.06%)
14. (1.99778) BD ( 1) B 1- F 2
( 17.29%) 0.4158* B 1 s( 33.25%)p 1.98( 65.98%)d 0.02( 0.76%)
( 82.71%) 0.9094* F 2 s( 36.24%)p 1.76( 63.62%)d 0.00( 0.14%)
---------------- non-Lewis ----------------------------------------------------
17. (0.38551) LV ( 1) B 1 s( 0.00%)p 1.00(100.00%)d 0.00( 0.00%)
18. (0.05374) BD*( 1) B 1- F 2
( 82.71%) 0.9094* B 1 s( 33.25%)p 1.98( 65.98%)d 0.02( 0.76%)
( 17.29%) -0.4158* F 2 s( 36.24%)p 1.76( 63.62%)d 0.00( 0.14%)
Here you can clearly see the approximate two sp orbitals and the remaining p lone pair orbitals.
The above remains true for (almost) all terminal atoms, because of the local linear symmetry (only one bonding partner, negligible external field), although with lesser extend for heavier atoms (like bromine). This is because hybridisation in general becomes a less reliable description due to the increase in the s-p gap.