Copper II forms many types of complexes with water. The aquo ion is [Cu(H$_2$O)$_6$]$^{2+}$$\ce{[Cu(H2O)6]^{2+}}$ where the oxygen ligates to the metal atom. The structure is a distorted octahedral one, two bonds are longer than the other four. (The Jahn-Teller effect$^*$ is behind the fact that two ligands are not bound as strongly as the other four). It is possible with ammonia to form various hydro complexes; [Cu(NH$_3$)(H$_2$O)$_5$]$^{2+}$$\ce{[Cu(NH3)(H2O)5]^{2+}}$...[Cu(NH$_3$)$_5$(H$_2$O)]$^{2+}$ $\ce{[Cu(NH3)5(H2O)]^{2+}}$. Similar complexes form with chloride ions. Copper sulphate is coloured in solution (due to [Cu(H$_2$O)$_6$]$^{2+}$$\ce{[Cu(H2O)6]^{2+}}$ ion) and in the crystal; the anhydrous crystal is colourless and this seems to indicate that the waters are bound, due to metal to ligand transitions, as in other transition metals. (The reason that sulphate is not significantly bound to the copper in aqueous solution, as it is in the crystal, is due to the vast excess of water (water is 55.5 M) which will displace the sulphate.)
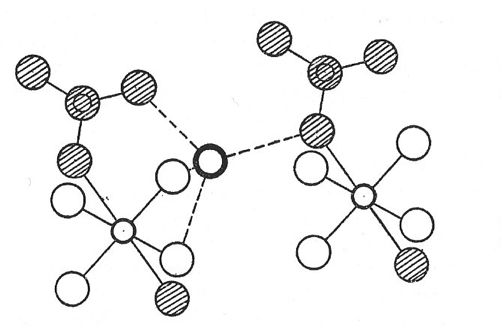
In the crystal of CuSO$_4$.5H$_2$O$\ce{CuSO4.5H2O}$ the metal is, as usual, 6 coordinated, with four water oxygens in the plane and one oxygen from a sulphate occupying each axial position. The extra ($5^{th}$$5^{\text{th}}$) water molecule is hydrogen bonded between the second oxygen of the sulphate and a bound water molecule in the plane. This same water's oxygen is also H$\ce{H}$-bonded to a sulphate oxygen bound to the next Cu$\ce{Cu}$ atom. The H$\ce{H}$ bonds are not quite of equal length 0.287 and 0.294 nm respectively.
(Fifth water oxygen thick black circle, sulphate hashed, Cu$\ce{Cu}$ small circle, other four oxygens, large circles. Source: Wells 'Structural Inorganic Chemistry')
So bonds are broken, Cu$\ce{Cu}$ to oxygen, also oxygen to oxygen and oxygen to sulphur hydrogen bonds as water is removed.
$^*$ The Jahn Teller theorem shows that any non-linear molecule in a degenerate state will undergo a distortion which will lower its symmetry and split the degenerate state. The theorem does not indicate what the distortion will be, or how big it will be. Nevertheless it is extremely important in describing the geometry of metal complexes.