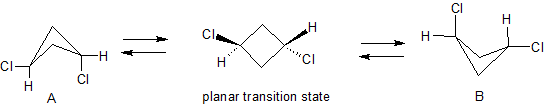
Cyclobutane and its substituted derivatives readily undergo a ring flip (or ring inversion) as pictured below.
The barrier to ring flipping is very low, around 1.5 kcal/mole, so at room temperature the flipping process is very rapid. The lowest energy conformation of cyclobutane exists in a puckered geometry as depicted in $\ce{A}$ and $\ce{B}$. In the ring flipping process, the molecule passes through a transition state where the cyclobutane ring is planar.
Only molecules that belong to symmetry classes (point groups)
- $\ce{C_{n}}$ (the molecule only contains a $\ce{C_{n}}$ axis)
- $\ce{C_{nv}}$ (the molecule contains a $\ce{C_{n}}$ axis and a $\ce{\sigma}_{v}$ plane)
- $\ce{C_{s}}$ (the molecule only has a plane of symmetry)
can have a dipole moment.
Conformers $\ce{A}$ and $\ce{B}$ both have $\ce{C_{s}}$ symmetry (the only symmetry element is a plane that bisects the ring and contains the two cyclobutane carbons bearing the substituents) and therefore do have a dipole moment. However, the dipole moments of conformers $\ce{A}$ and $\ce{B}$ are equal and opposite, so when flipping is rapid the dipole moment averages out to zero.
Therefore at room temperature, where flipping is rapid, the molecule has zerono measurable dipole moment. If you cooled the system down to a very low temperature where conformers $\ce{A}$ and $\ce{B}$ were not rapidly interconverting, then you could measure a non-zero dipole moment.