To complement Nisarg's answer, note that we have the following system of polynomial ODEs
$$\begin{bmatrix} \dot a \\ \dot b \\ \dot c\end{bmatrix} = \left( 3 a b - \frac32 c \right) \begin{bmatrix} -1\\ -1\\ +1\end{bmatrix}$$
Thus, given an initial state, the state will flow along a given line. Since concentrations must be non-negative, given an initial state, the set of all states is a line segment (one of whose endpoints is to be determined) in the non-negative octant. Equilibrium is found at the algebraic surface defined by
$$3 a b - \frac32 c = 0$$
Suppose that the initial state is $(1,1,0)$. Pictorially,

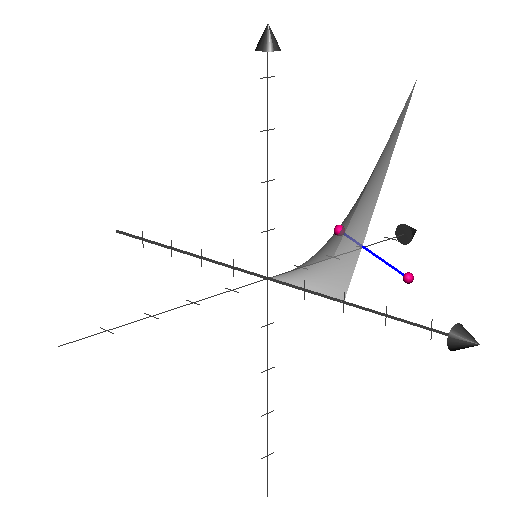
where the equilibrium point is $\left( \frac12, \frac12, \frac12 \right)$.
