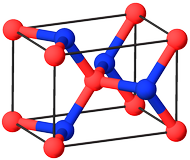
To address you concern about boron, there is a cubic diamond form of boron nitride $\ce{c-BN}$, ICSD #182731 [1], posseseswhich possesses $V_\mathrm{cell} = \pu{7.99 Å3}$, $Z = 2$ and the molar volume
$$V_\mathrm{m} = \frac{N_\mathrm{A}V_\mathrm{cell}}{Z} = \frac{\pu{6.022e23 mol-1}\cdot\pu{7.99 Å3}}{2} \approx \pu{2.406e-6 m3 mol-1}$$$$V_\mathrm{m} = \frac{N_\mathrm{A}V_\mathrm{cell}}{Z} = \frac{(\pu{6.022E23 mol-1})(\pu{7.99 Å3})}{2} \approx \pu{2.406E-6 m3 mol-1},$$
which is about $30\%$ less than that for the diamond. The only drawback is that this form of boron nitride is predicted to be stable above $\pu{11 Mbar}$.
Figure 1. Unit cell of $\ce{c-BN}$. Color code: $\color{#FFB5B5}{\Large\bullet}~\ce{B}$; $\color{#3050F8}{\Large\bullet}~\ce{N}$.
References
- Qiu, S. L.; Marcus, P. M. Structure and Stability under Pressure of Cubic and Hexagonal Diamond Crystals of C, BN and Si from First Principles. Journal of Physics: CondensedJ. Phys. Condens. Matter 2011, 23 (21), 215501. DOI: https://doi.org/10.1088/0953-8984/23/21/215501.