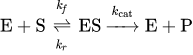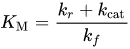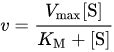I want to find a lower limit of the Michaelis constant for some evaluations of Michaelis-Menten enzyme kinetics. What is the lowest $K_m$ you ever encountered? Is there a theoretical limit?
-
1$\begingroup$ en.wikipedia.org/wiki/… $\endgroup$– MithoronCommented Nov 23, 2017 at 15:50
-
$\begingroup$ The link does provide some examples, but no lower limit for k_m. $\endgroup$– PascalIvCommented Nov 24, 2017 at 18:22
-
$\begingroup$ It is generally believed that evolution places a theoretical limit on kcat/Km, but not necessarily on Km. I know of one reported Km in the nanomolar rnage (3.2 nM to be precise): aem.asm.org/content/85/13/e00438-19. There are probably others that are lower. $\endgroup$– AndrewCommented Sep 23, 2020 at 20:04
-
1$\begingroup$ Since the function under consideration is catalytic (accelerating the reaction rate), you might consider the natural rate of the reaction (in absence of enzyme) as setting a limit on kcat. From the diffusion limited on rate (as explained in an answer) and setting the off-rate kr to zero you can estimate a lower meaningful (theoretical) bound on Km. $\endgroup$– Buck Thorn ♦Commented Sep 23, 2020 at 20:45
-
1$\begingroup$ The other way to set an upper limit on kcat is by the lifetime of the enzyme. Most cellular proteins are degraded within hours or days. So you could set a conservative lifetime as 100 days and say that at least one turnover must happen (on average) within that time, so minimum kcat is 3 per year ~ $1\times 10^{-8}$ s$^{-1}$. Dividing by kf = 10^9 M-1s-1 gives a limit on Km of $10^{-17}$ M give or take a few orders of magnitude. $\endgroup$– AndrewCommented Sep 24, 2020 at 16:44
1 Answer
Take this generic reaction representing the Michaelis-Menten enzyme kinetic:
You can derive the Michaelis-Manten constant $K_m$:
(for derivation see https://en.wikipedia.org/wiki/Michaelis%E2%80%93Menten_kinetics)
The quasi-steady state hypothesis was used, therefore the rate of formation and breakdown of $ES$ are the same; this is a good approximation if the enzyme concentration is much less than the substrate concentration $[S]$ or $K_m$ or both. That means that $K_m$ can be extremely small (almost zero) and the Michaelis-Menten equation still be valid proven that the enzyme concentration is much less than $[S]$.
$K_m$ is very small if:
$k_r$ and $k_{cat}$ are both very small compared to $k_f$, thus if the complex $ES$ is formed very quickly compared to its breakdown.
In this case the reaction proceeds at its maximum velocity $V_{max}=k_{cat}*E_0$, with $E_0=[ES]+[E]$:
About the theoretical limit on how small $K_m$ can be there are two considerations:
First, $k_f$ cannot be cannot be faster than the diffusion-controlled encounter of an enzyme and its substrate. This means that $k_f$ cannot be higher than $10^9 s^{-1} M^{-1}$ (ref.https://www.ncbi.nlm.nih.gov/books/NBK22430/#:~:text=This%20rate%20cannot%20be%20faster,s%2D1%20M%2D1.)
Second, $k_{cat}$ cannot be too small or the product $P$ formed is produced at a rate not suitable for the cell survival.
Generally, I haven't encountered $K_m$ smaller than $10^{-6}M$ in biological enzymes (e.g Triosephosphate isomerase, TPI) but possibly there are. In TPI, $ k_f$ ∼ $10^9 s^{-1} M^{-1}$, therefore $k_r$ ∼ $k_{cat}$ ∼ $10^{3}s^{-1}$ that is still quite high. It would be very interesting to know if there are enzymes with an even lower $K_m$. Very intresting question.