6
$\begingroup$

When I tried to count, I found 24 tetrahedral holes (each formed by 2 body centers of 2 unit cells sharing a face and 2 vertices of the same edge of that face). As each hole is being shared by 2 unit cells, there are 12 holes per unit cell.

Also,I found 6 octahedral holes (6 at face centers being shard by 2 unit cells each and 12 at every edge center being shared by 4 unit cells each).

Generally I was taught that for $n$ packed spheres, there should be $2n$ tetrahedral holes. Does the analysis above mean that this rule doesn't apply here?

Improve this question
$\endgroup$
2
  • 3
    $\begingroup$ Bcc isn't a close packed structure, so I don't think one should expect that the usual 1:1:2 ratio of sphere:octahedral hole:tetrahedral hole is fulfilled. As far as I can tell, your analysis leading to 6 octahedral and 12 tetrahedral per unit cell is correct (textbooks state this when describing the structure of α-AgI). However, these holes are slightly distorted from perfect octahedral/tetrahedral symmetry. $\endgroup$
    – orthocresol
    Commented Aug 27, 2017 at 15:21
  • 1
    $\begingroup$ Exactly what you are asking : youtu.be/J8gsZ50Vcqs $\endgroup$
    – Kashmiri
    Commented Nov 30, 2020 at 18:17

3 Answers 3

Reset to default
3
$\begingroup$

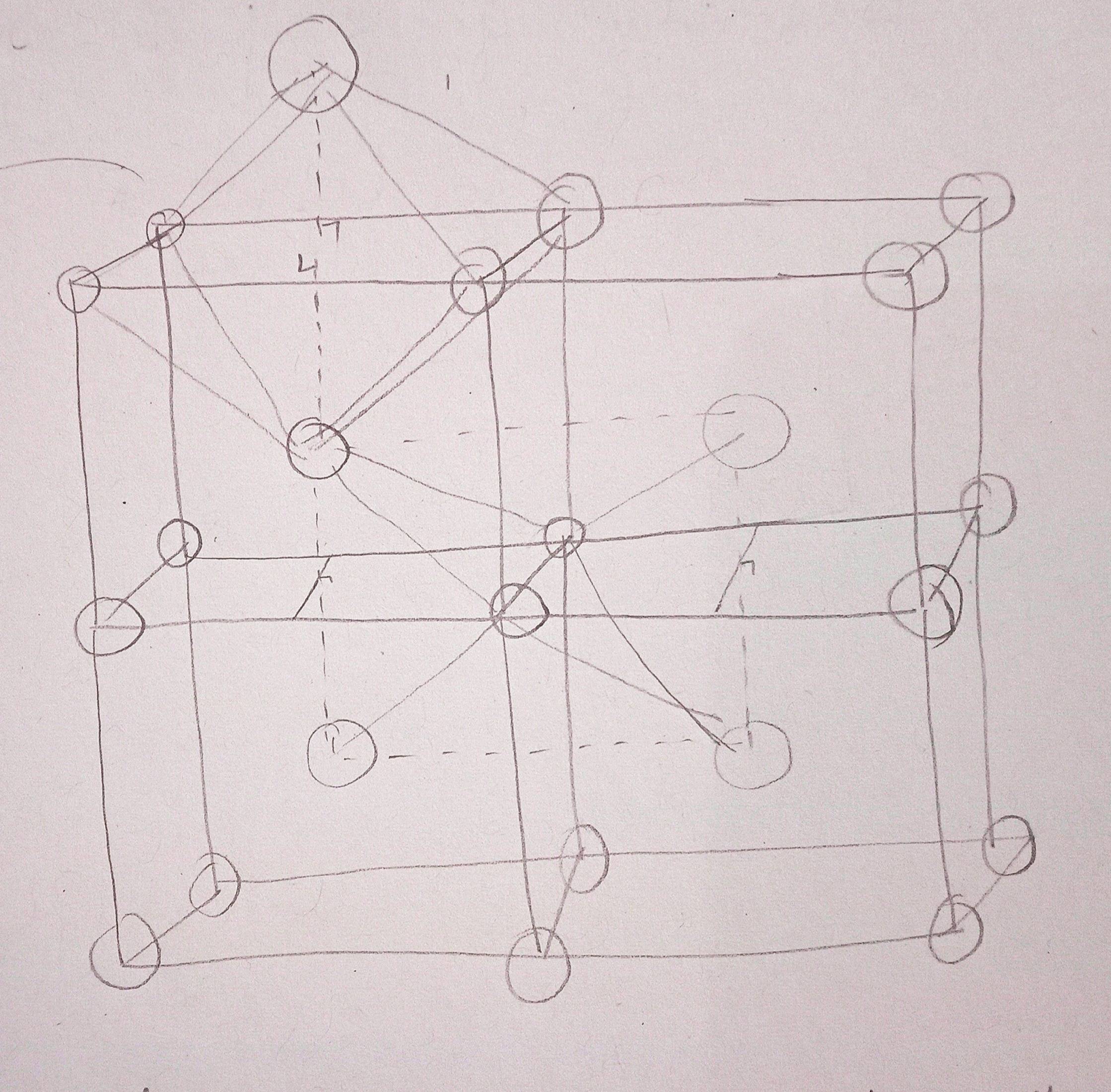enter image description here It's a partial answer : The diagram shows two places where irregular octahedral voids exist in a BCC lattice. By symmetry you'll be able to say that there exist:

6 octahedral voids on each surface and 12 octahedral voids on each edge length which gives us $6$ octahedral voids per unit cell.

A professor goes on for an hour to explain it here: https://youtu.be/J8gsZ50Vcqs

Improve this answer
$\endgroup$
2
$\begingroup$

BCC doesn't have any tetrahedral voids, however we have octahedral holes at face centres. Four adjacent corners and two closest body centres. In a homogeneous lattice the octahedral is compressed along the body-centre-atom axis. However I believe we can form a hetrogenous lattice and form a close approximation to octahedral.

We have thus $6\times1/2 = 3$ distorted octahedral voids.

Improve this answer
$\endgroup$
1
  • $\begingroup$ BCC does have distorted tetrahedral voids $\endgroup$
    – Kashmiri
    Commented Nov 30, 2020 at 17:59
2
$\begingroup$

A BCC has 6 octahedral holes and 12 tetrahedral holes.

At each face of the bcc, there is one octahedral hole. There is also an octahedral hole on each edge. We divide the holes found on the faces by 2 as they lay between two unit cells, and we divide the holes found on the edges by 4 because they likewise lay between 4 unit cells. There are 6 faces and 12 edges in a cubic cell.

6/2 + 12/4 = 6 octahedral voids

Likewise, there is a total of 12 tetrahedral voids found within a bbc.

If you have trouble visualizing tetrahedral holes, use a dice (or any cube) and point one corner towards you so that you are staring down the C3 axis of rotation. You should be able to see that the edges connected from the corner closest to you to the corners further from you clearly form a tetrahedron.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.