This has been bugging me for a while now...
Obviously, to calculate the volume/space occupied by a mole of (an ideal) gas, you'll have to specify temperature ($T$) and pressure ($P$), find the gas constant ($R$) value with the right units and plug them all in the ideal gas equation $$PV = nRT.$$
The problem? It seems to be some sort of common "wisdom" all over the Internet, that one mole of gas occupies $22.4$ liters of space. But the standard conditions (STP, NTP, or SATP) mentioned lack consistency over multiple sites/books. Common claims: A mole of gas occupies,
- $\pu{22.4 L}$ at STP
- $\pu{22.4 L}$ at NTP
- $\pu{22.4 L}$ at SATP
- $\pu{22.4 L}$ at both STP and NTP
Even Chem.SE is rife with the "fact" that a mole of ideal gas occupies $\pu{22.4 L}$, or some extension thereof.
Being so utterly frustrated with this situation, I decided to calculate the volumes occupied by a mole of ideal gas (based on the ideal gas equation) for each of the three standard conditions; namely: Standard Temperature and Pressure (STP), Normal Temperature and Pressure (NTP) and Standard Ambient Temperature and Pressure (SATP).
Knowing that,
- STP: $\pu{0 ^\circ C}$ and $\pu{1 bar}$
- NTP: $\pu{20 ^\circ C}$ and $\pu{1 atm}$
- SATP: $\pu{25 ^\circ C}$ and $\pu{1 bar}$
And using the equation, $$V = \frac {nRT}{P},$$ where $n = \pu{1 mol}$, by default (since we're talking about one mole of gas).
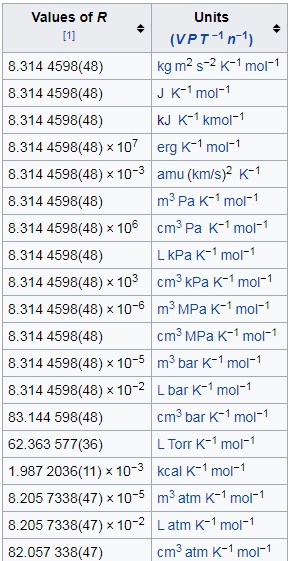
I'll draw appropriate values of the gas constant $R$ from this Wikipedia table:
The volume occupied by a mole of gas should be:
At STP \begin{align} T &= \pu{273.0 K},& P &= \pu{1 bar},& R &= \pu{8.3144598 \times 10^-2 L bar K^-1 mol^-1}. \end{align} Plugging in all the values, I got $$V = \pu{22.698475 L},$$ which to a reasonable approximation, gives $$V = \pu{22.7 L}.$$
At NTP \begin{align} T &= \pu{293.0 K},& P &= \pu{1 atm},& R &= \pu{8.2057338 \times 10^-2 L atm K^-1 mol^-1}. \end{align} Plugging in all the values, I got $$V = \pu{24.04280003 L},$$ which to a reasonable approximation, gives $$V = \pu{24 L}.$$
At SATP \begin{align} T &= \pu{298.0 K},& P &= \pu{1 bar},& R &= \pu{8.3144598 \times 10^-2 L bar K^-1 mol^-1}. \end{align} Plugging in all the values, I got $$V = \pu{24.7770902 L},$$ which to a reasonable approximation, gives $$V = \pu{24.8 L}.$$
Nowhere does the magical "$\pu{22.4 L}$" figure in the three cases I've analyzed appear. Since I've seen the "one mole occupies $\pu{22.4 L}$ at STP/NTP" dictum so many times, I'm wondering if I've missed something.
My question(s):
- Did I screw up with my calculations?
- (If I didn't screw up) Why is it that the "one mole occupies $\pu{22.4 L}$" idea is so widespread, in spite of not being close (enough) to the values that I obtained?