To expand on my comment above, I will reiterate that all chemical reactions are equilibria and this is due to the interplay of enthalpy and entropy. More specifically, it is due to enthalpy and entropy of the individual compounds of the reaction as well as an additional entropy component due to mixing.
Consider the general reaction $$\ce{A<=>B}$$ and let $\chi_\ce{i}$ denote the mole fraction of compound $\ce{i}$ in the solution or gas mixture. The enthalpy and the entropy of the compounds, combined together to form $\Delta G_{compounds}=\Delta H_{compounds}-T\Delta S_{compounds}$ or alternatively $$G=(\chi_\ce{B})\cdot H_{\ce{B}}+(1-\chi_\ce{B})\cdot H_{\ce{A}}-(\chi_\ce{B})\cdot T\Delta S_{\ce{B}}-(1-\chi_\ce{B})\cdot T\Delta S_{\ce{A}}$$ has a particular side of the reaction it favors. This component of the free energy leads to a complete preference; if this were the only factor, the solution would be all $\ce{A}$$(\chi_\ce{A}=1)$ or all $\ce{B}(\chi_\ce{B}=1)$. If we were to plot $G$ as a function of $\chi_\ce{B}$, it would either be linear increasing (reactants favored), linear decreasing (products favored), or flat (reactants and products equally favored).
However, there is an additional entropy component due to mixing, which leads to an additional free energy component. We can write the entropy of mixing as $$\Delta S_{mixing}=-\mathrm{R}[\chi_\ce{A}\ln(\chi_\ce{A})+\chi_\ce{B}\ln(\chi_\ce{B})]=-\mathrm{R}[(1-\chi_\ce{B})\ln(1-\chi_\ce{B})+(\chi_\ce{B})\ln(\chi_\ce{B})]$$
where we have used the fact that $\chi_\ce{B}+\chi_\ce{A}=1$. We can then write the free energy of mixing as $$\Delta G_{mixing}=\mathrm{RT}[(1-\chi_\ce{B})\ln(1-\chi_\ce{B})+(\chi_\ce{B})\ln(\chi_\ce{B})]$$ We can see graphically what these two mixing equations would look like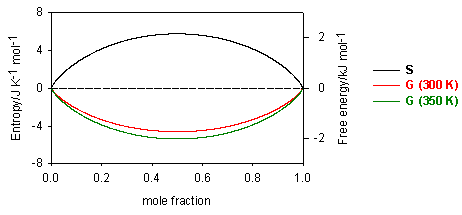
Combining all this together, we can write $$G_{total}=(\chi_\ce{B})\cdot H_{\ce{B}}+(1-\chi_\ce{B})\cdot H_{\ce{A}}-(\chi_\ce{B})\cdot T\Delta S_{\ce{B}}-(1-\chi_\ce{B})\cdot T\Delta S_{\ce{A}}+\mathrm{RT}[(1-\chi_\ce{B})\ln(1-\chi_\ce{B})+(\chi_\ce{B})\ln(\chi_\ce{B})]$$ We can take the derivative of this function with respect to $\chi_\ce{B}$ and set it equal to zero to determine where the miniumum free energy is $$\frac{\delta G_{total}}{\delta\chi_\ce{B}}=0= H_{\ce{B}} - H_{\ce{A}}- T\Delta S_{\ce{B}}- T\Delta S_{\ce{A}}+\mathrm{RT}[-\ln(1-\chi_\ce{B})+\ln(\chi_\ce{B})]$$ $$H_{\ce{B}} - H_{\ce{A}}- T\Delta S_{\ce{B}}- T\Delta S_{\ce{A}}=-\mathrm{RT}[-\ln(1-\chi_\ce{B})+\ln(\chi_\ce{B})]$$
This second equation is the crux of all this. While we cannot solve this for an exact value without knowing the enthalpy and entropy of the compounds, we can definitively say that $\chi_\ce{B}\ne1$ and $\chi_\ce{B}\ne0$. Why? Plugging either of the values into the equation will result in the right side being $\infty$, meaning the differences in enthalpy and entropy of the components would also have to be $\infty$ for the equation to be satisfied. Thus, the minimum free energy will never be attained by letting the reaction go to completion; it will always be more favorable to allow at least some mixing even if one side of the reaction has a much lower enthalpy and higher entropy of its compounds.
Thus all reactions are technically equilibria. I say technically because the mixing free energy might be negligible compared to the overall free energy and so the reaction could appear to have gone to completion to the extent that we can observe experimentally.
So for your reaction, the reason it doesn't all remain as $\ce{CH3COOH}$ and water is that the entropy of mixing makes it favorable for some of the $\ce{CH3COOH}$ to dissociate.
(For a brief version of this argument and where I got the graph, see http://rkt.chem.ox.ac.uk/tutorials/equilibrium/entropy_mixGas.html)

