Is hybridization used in ab initio valence bond calculation?

Question

Many general chemistry textbooks introduced the concept "hybridization" to construct a symmetry-adapted VB-type wavefunction. In the textbooks, usually the minimal basis is used and without optimizing all resonance forms.

My question is, in the ab initio valence bond calculation with extended basis set and optimizing many resonance forms,

(i) do people still use hybridization to construct the wavefuntion?

(ii) if the answer to question (i) is yes, does the result differ from the textbook description? For instance, the lone pair electron in the $\ce{H2O}$ molecule? (is there any unitary equivalent result like orbital localization in MO-based method?)

I asked the question (ii), since NBO analysis tends to give non-equivalent composition for lone pairs in $\ce{H2O}$, one sp, the other p. I am wondering what is the answer in ab initio valence bond method.

Answer 1

(i) do people still use hybridization to construct the wavefuntion?

Yes and no. No: Only atomic orbitals are included in the base set. Yes: if the wavefunction is optimized, linear combinations of atomic orbitals are allowed, and we all know that one S-orbital + some P-orbitals give hybridized orbitals.

For $\ce{H2O}$, the lowest 3 molecular orbitals are generally these (see below):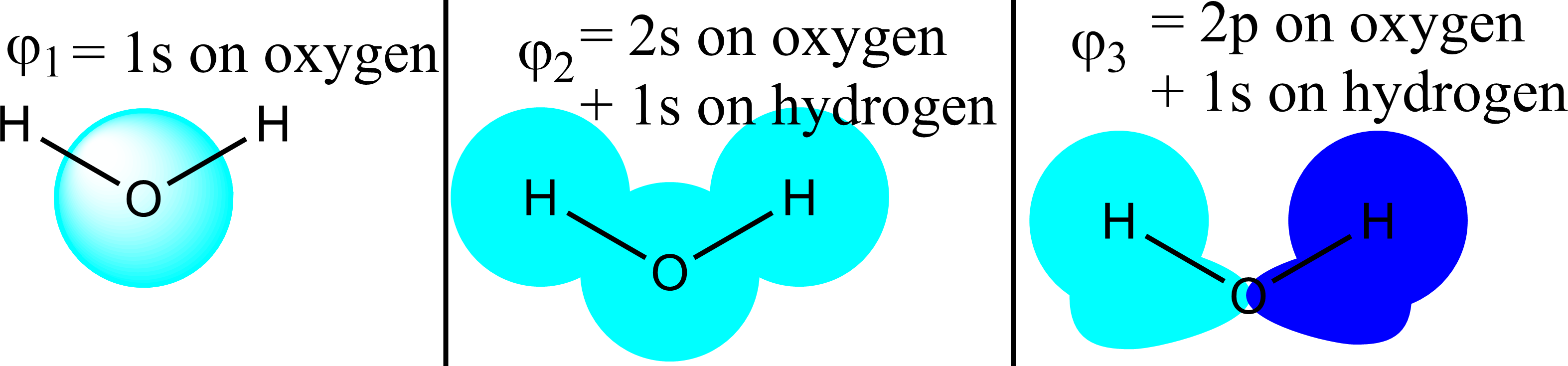

At first, this result seems unlike the SP$^3$ bonds between hydrogen and carbon. There is a trick, however. It is called "localization of the orbitals", meaning that we generate linear combinations of molecular orbitals to generate new ones. Below, I'll give you an example in which we linear combinations of molecular orbitals $\varphi_2$ and $\varphi_3$ generate orbitals that look more like the SP$^3$ orbitals from text books.

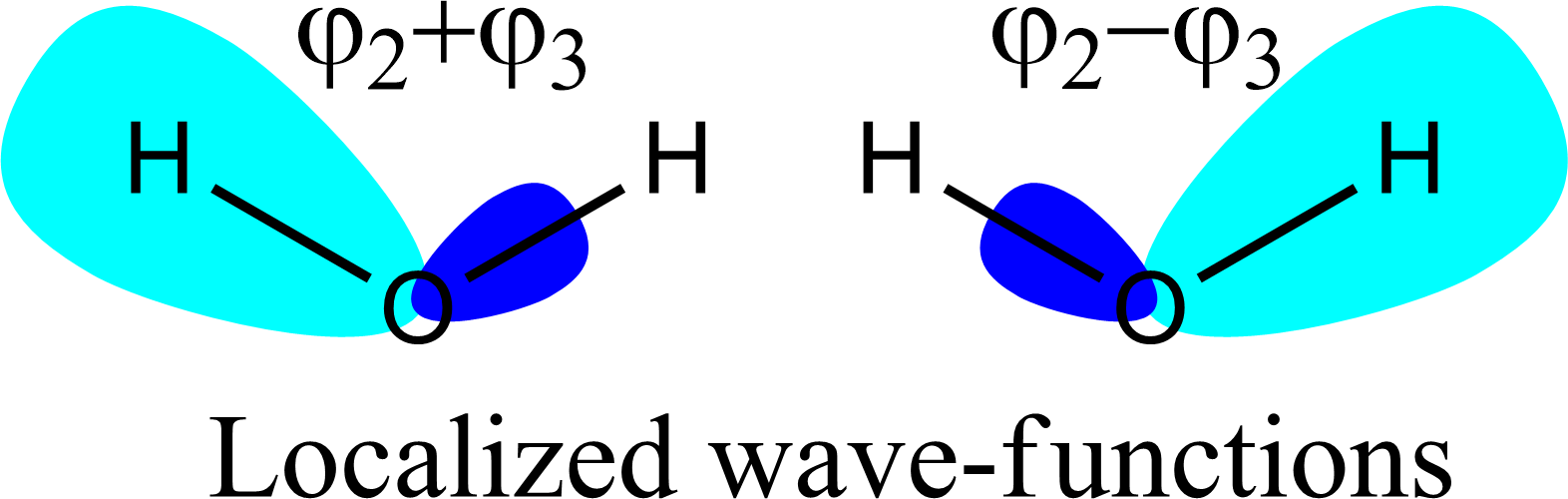
(ii) if the answer to question (i) is yes, does the result differ from the textbook description? For instance, the lone pair electron in the $\ce{H2O}$ molecule? (is there any unitary equivalent result like orbital localization in MO-based method?)

As you may have noticed above, the results differ slightly, but I can elaborate.

Lone pairs are given by $\varphi_4$ and $\varphi_5$ below.

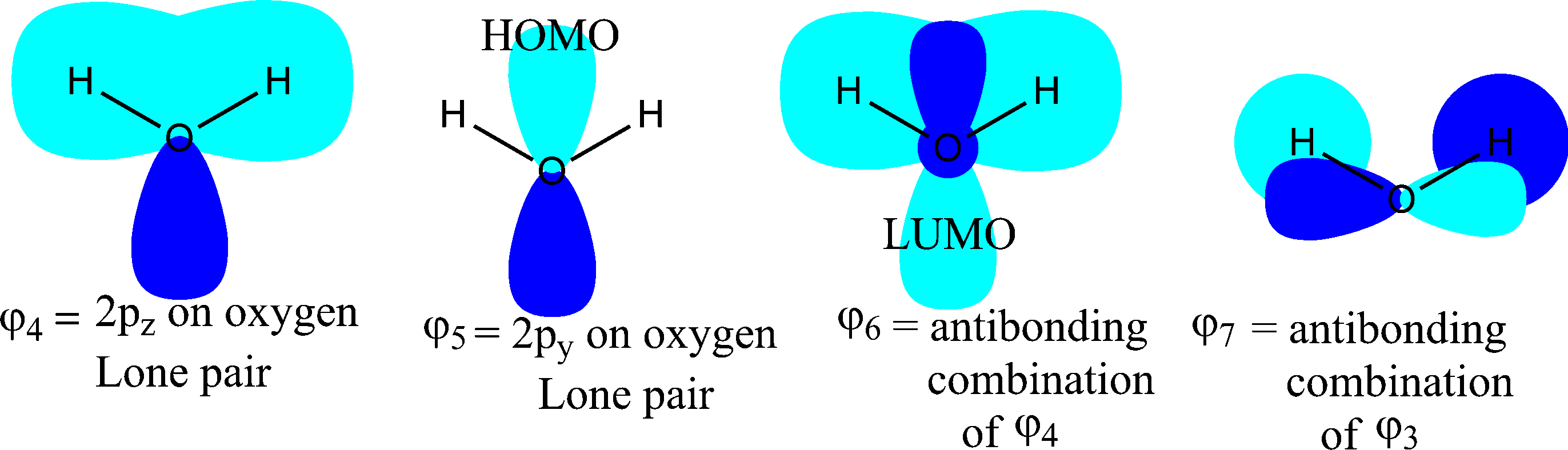
For $\varphi_4$, note that p$_z$ is along the z-axis, which is in the plane of the oxygen and hydrogen atoms: it is the 2-fold rotation axis.

For $\varphi_5$, note that p$_y$ is along the y-axis, which is perpendicular to the plane of the oxygen and hydrogen atoms: it is out of the plane.

For $\varphi_6$ and $\varphi_7$, note that they are unoccupied orbitals (i.e. lumo and lumo+1)

Now, orbital localization can be applied, linear combinations of $\varphi_4$ and $\varphi_5$ will give 2 lone pair orbitals, that stick slightly out of plane. I haven't tried drawing those yet, as it is not so easy to do so in this representation in 2D, so I'l try to switch to another representation...

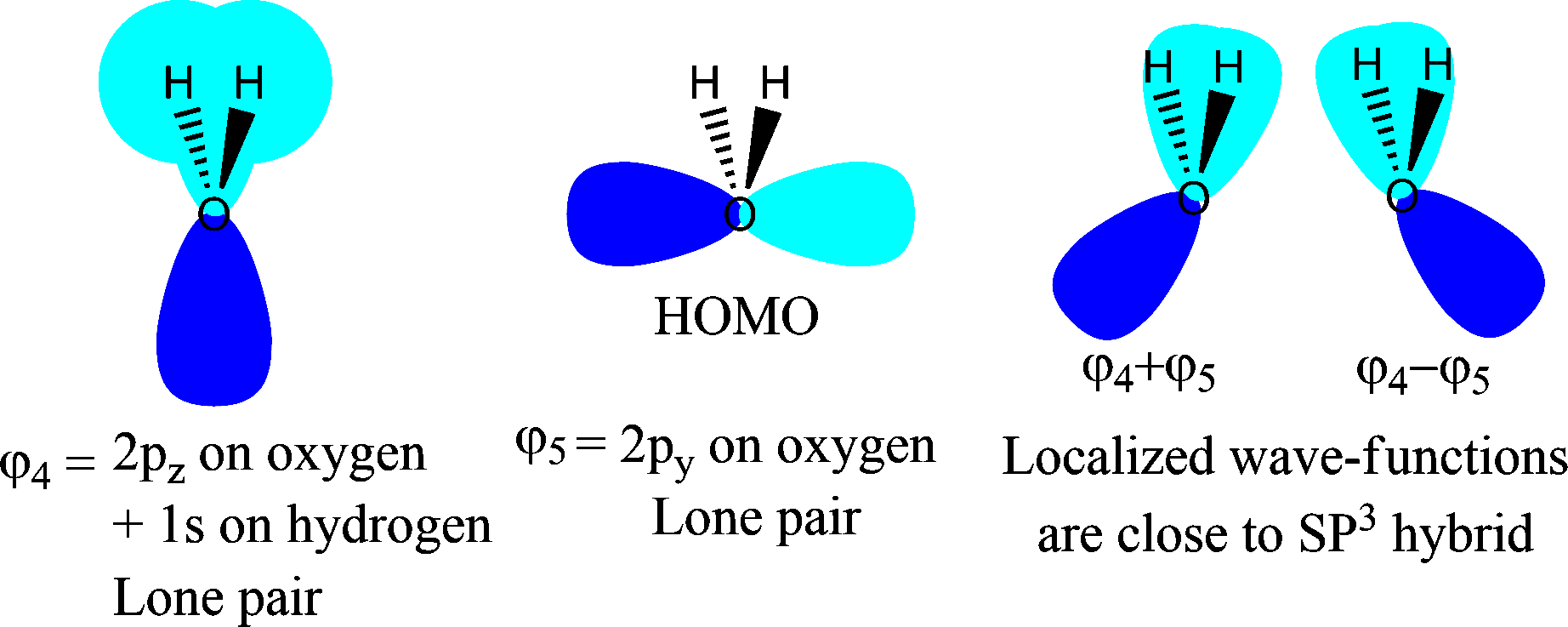
As you see, those localized molecular orbitals closely resemble the SP$^3$ lone pair orbitals on oxygen, as predicted by valence bond theory. The only difference is the shape & probability of the small counter-part of the lobe; I included phase, not probability density here.

Does this answer your question?

Answer 2

I can answer your first question definitively from my experience in quantum chemistry. Yes, hybrid orbital basis sets are still used, albeit minimally. Typically, these basis sets are used in QM/MM in hopes to scale down the QM computation. There are severe limitations in this approach. You tend to fix the geometry to hybrid orbitals, as you can only overlap certain orbitals. It becomes "ball and stick" chemistry. For this reason, it is not popular. Better to use a traditional QM technique on a smaller region where you will have reactivity.

I can attempt to answer your second question from a comprehensive standpoint as I have never done ab-initio calculations myself using hybrid orbitals. The results should be similar for geometries of simple molecules and break down for increasingly complex molecules. This is because non-VB electrons can undergo orbital overlap.