5
$\begingroup$

Explain how a molecular orbital can have a nodal plane and still be a bonding molecular orbital.

I understand the concept of how nodal planes are formed and how they have a probability of 0 when trying to find an electron in the nodal plane, but I don't understand how it can be considered a bond especially since these nodal planes are usually called "anti-bonding". This question itself seems contradictory and I'm having a hard time trying to understand how this is possible.

Improve this question
$\endgroup$
0

2 Answers 2

Reset to default
3
$\begingroup$

The number of nodal planes isn't the determining factor. Yes, having more nodal planes increase the energy of the orbital, but you need to consider the fact that some MOs (specifically the anti bonding ones) are raised in energy. Overall, the total energy of the orbitals is the same as before, so you stabilize some and destabilize others. Take a look at the MOs and you can see that the there's two bonding MOs with one nodal plane perpendicular to the plane of the benzene with $E_{1}$ symmetry. Likewise, there's a pair of MOs with two nodal planes that with $E_{1}$ symmetry that are anti-bonding. These pairs happened to be correlated in that the increase in energy of the anti-bonding orbitals is essentially matched by the stabilization of the bonding orbitals.

Improve this answer
$\endgroup$
2
$\begingroup$

An intuitive way of looking at this is that when you have many atoms to consider, there will be multiple bonding/antibonding interactions between atoms. Whether the MO is overall bonding or antibonding will depend on the sum of these interactions. This is rather simplified, but using the example of benzene again, we can examine the three bonding π MOs:

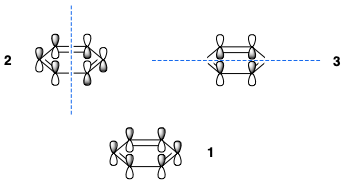
π bonding MOs of benzene

MO 1 (the lowest-energy π MO) has one nodal plane in the plane of the ring. However, because this isn't a plane separating two constituent atomic orbitals (the p orbitals), this nodal plane doesn't tell us anything about bonding/antibonding properties of the MO. Indeed, this nodal plane only arises because the building blocks – the p-orbitals – have a node in their centre. The interactions between different p-orbitals are all bonding in nature, because the shaded (positive) lobes of each p-orbital are overlapping with the shaded lobes of their neighbours (and vice versa for the unshaded lobes).

MO 2 has a nodal plane between p-orbitals, which suggests the presence of antibonding character. That is true: there are two major antibonding interactions, one between the top-left and top-right carbons, and one between the bottom-left and bottom-right carbons. (The middle-left and middle-right carbons are too far apart). However, there are also multiple bonding interactions: the three carbons on the left, for example, are all interacting with each other in a bonding fashion. Overall, this MO is bonding, although not quite "as bonding" as MO 1.

Likewise, MO 3 has antibonding interactions between the top-left and bottom-left carbons (for example). It also has bonding interactions between the top-left and top-right carbons. However, the antibonding interactions are occurring over a greater distance than the bonding interactions. Consequently, they are weaker than the bonding interactions, and again we have a case of net bonding.

Improve this answer
$\endgroup$
5
  • $\begingroup$ I'm not sure if Hückel theory is rigorous enough to invoke, but in that theory the coefficients are also different, which is why even though MO3 has "fewer bonding" orbitals, it can still be degenerate with MO2. This can be intuitively depicted by varying the sizes of the orbitals. Page 5 in the following link shows this: ocw.mit.edu/courses/chemistry/5-61-physical-chemistry-fall-2007/… $\endgroup$
    – Blaise
    Commented Jan 24, 2019 at 2:53
  • $\begingroup$ I wish one would separate the concept of bonding/ anti-bonding interactions from energy considerations. There are probably examples where an 'overall bonding' MO is anti-bonding with respect to one bond, which will be elongated due to that fact. After all these considerations led to the believe that the HOMO of CO is anti-bonding, which cannot be supported by any kind of theory. There is one universal truth: All molecular orbitals have nodal surfaces. Within every MO there are bonding and/or anti-bonding interactions. $\endgroup$
    – Martin - マーチン ♦
    Commented Jan 25, 2019 at 18:13
  • 1
    $\begingroup$ @Blaise Symmetry alone dictates that MO2 and 3 depicted above are degenerate (and any linear combinations thereof). Hückel's calculations show that in a very simple and accurate way. $\endgroup$
    – Martin - マーチン ♦
    Commented Jan 25, 2019 at 18:18
  • $\begingroup$ @Martin-マーチン in Huckel theory, any interaction between atom $i$ and $j\neq i$ leads to a contribution of $2\beta_{ij}c_ic_j$ to the energy $E = \mathbf{c^THc}$; so AFAIK I think it is fair enough to link bonding ($c_ic_j > 0$) interactions to a decrease in energy and antibonding ($c_ic_j < 0$) interactions to an increase (since $\beta_{ij} < 0$). The way I'm seeing it (correct me if I'm wrong), the problem is linking this to the overall bonding/antibonding character of an MO. For benzene it is simple, because every p-orbital has the same value of $\alpha$; so for the MO if $E < \alpha$ [...] $\endgroup$
    – orthocresol
    Commented Jan 25, 2019 at 19:54
  • $\begingroup$ [...] we can say it is bonding, and if $E > \alpha$ it is antibonding. In the absence of any interactions between pairs of AOs (which corresponds to turning every off-diagonal element $\beta_{ij}$ to zero), every MO simply has the energy $\alpha$, so any form of bonding interactions will bring the energy below $\alpha$. Thus we regain the qualitative and rather simplified picture in my answer. For CO we don't have the privilege of all $\alpha_i$ being the same, and consequently I don't know if this kind of approach remains valid. $\endgroup$
    – orthocresol
    Commented Jan 25, 2019 at 19:57

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.