As you may know, atomic orbitals are wave functions, solutions of the Schrödinger equation for an atomic system.
In a perfectly spherical system you may express an orbital as a function depending on the distance from the nucleus ($r$) and two angles ($\phi$ and $\theta$).
[If you pick a particular $r$, the angles $\phi$ and $\theta$ work in a way similar to how we locate points on the surface of the earth (latitude and longitude).]
This being said, the image below may clarify things and answer your question (source):
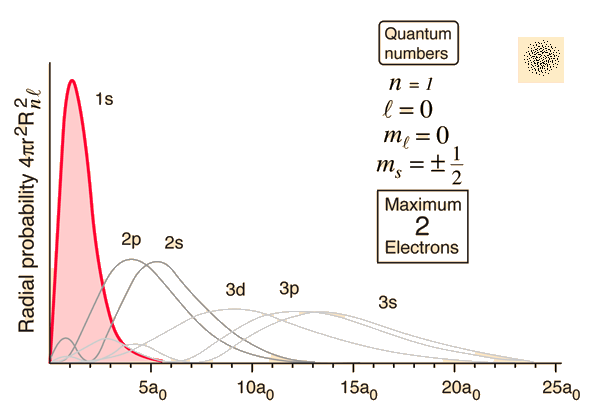
The graph deals only with s atomic orbitals of hydrogen, the 1s highlighted in red.
See the x-axis? It is measured in multiples of $a_0$ a.k.a. Bohr radius,
$$a_0 = \frac{4 \pi \epsilon_0 \hbar^2}{m_e e^2} = \frac{\hbar}{m_e c \alpha}$$
This is the radius obtained from Bohr's theory for the 1s orbital of hydrogen.
How can it the useful as a unit in this graph?
Well, the square of a wave function is a probability distribution, $\rho(r, \phi, \theta) = \Psi^2 (r, \phi, \theta)$.
If you had a dice with probability distribution $\bar{\rho}(n) = 1/6$ (a fair dice) for each $n = 1,..., 6$, you would get, throwing that dice, on average,
$$\sum_{n=1}^6 \bar{\rho}(n) \times n = \sum_{n=1}^6 \frac{n}{6} \\= \frac{1}{6} + \frac{2}{6} + \cdots + \frac{6}{6} = 3.5$$
So $3.5$ is the expected value of throwing a dice.
See how the last equality is just the usual average we're familiar with?
But the first one is most useful in our context.
Since our variables $r$, $\phi$ and $\theta$ are all continuous, in order to get an average radius, we integrate instead of sum:
$$\int_0^{2 \pi} \int_0^{\pi} \int_0^\infty [\rho(r, \phi, \theta) \times r] r^2 \sin(\phi) d r d \phi d \theta $$
$r^2 \sin(\phi) d r d \phi d \theta$ is just the volume element in spherical coordinates (in our dice example we had an implicit $1$ there as "volume"), what matters is just what is in squared brackets.
Now 1s ($n = 1$, $\ell = 0$) orbitals do not depend on angles, they are perfectly spherical.
That means that $\Psi_{n \ell m_\ell}(r, \phi, \theta) = R_{n \ell} (r)$ with $n = 1$, $\ell = 0$ and $R_{n \ell} (r)$ is some function describing the radial electron wave function.
Our integration simplifies to:
$$\int_0^{2 \pi} \int_0^{\pi} \int_0^\infty [R^2_{n \ell} (r) \times r] r^2 \sin(\phi) d r d \phi d \theta \\
= \int_0^{2 \pi} \int_0^{\pi} \sin(\phi) d \phi d \theta \int_0^\infty [R^2_{n \ell} (r) \times r] r^2 d r \\
= 4 \pi \int_0^\infty [R^2_{n \ell} (r) \times r] r^2 d r \\
= \int_0^\infty [ (4 \pi r^2 R^2_{n \ell} (r)) \times r ] d r $$
This surely looks like the expected value of throwing a dice!
The variable being averaged is $r$ and the new probability distribution is $\rho_\text{radial} (r) = 4 \pi r^2 R^2_{n \ell} (r)$, called radial probability distribution.
That's what's being plotted in the figure above.
If you go further and carry out the last integral ($R_{1 0} (r)$ can be found here), you'll see the answer is just $a_0$, the Bohr radius.
That's expected, after all, since Bohr's theory was quite successful for the hydrogen atom.
Nothing has been disproved.
Thus, the atomic radius of an atom can be obtained from quantum mechanics by calculating the expected value of the distance between the outermost electron and the nucleus, as MaxW has pointed out.

