6
$\begingroup$

I understand the geometry and calculation of a dihedral angle, discussed in this question. It is not clear how the direction is defined for a chemical bond. On the wiki page for dihedral angles, it states that, "the dihedral angle $\phi$ is the counterclockwise angle..." between the planes $P_{123}$ and $P_{234}$ (where $P_{123}$ is defined as the plane containing atoms 1, 2, and 3 in the diagram below, and likewise for $P_{234}$).

$\hspace{45 mm}$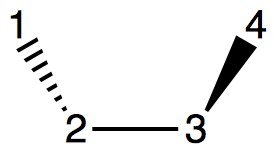bonds

This counterclockwise definition means that the direction of the dihedral angle is defined using the left-hand rule about the line connecting atom 2 to atom 3. The left-hand rule is unusual in mathematics and geometry. Alternatively, this example may be defining the directionality of $\phi$ simply so the result is a positive angle less than $180^\circ$.

I have not been able to find any chemistry standards on this. Is there a common convention?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
5
$\begingroup$

The IUPAC Gold Book states that,

In a Newman projection the torsion angle is the angle (having an absolute value between 0° and 180°) between bonds to two specified (fiducial) groups, one from the atom nearer (proximal) to the observer and the other from the further (distal) atom. The torsion angle between groups A and D is then considered to be positive if the bond A-B is rotated in a clockwise direction through less than 180° in order that it may eclipse the bond C-D: a negative torsion angle requires rotation in the opposite sense.

This implies a range of -180 to 180, which is by far the most typical range used, but I certainly have seen reports ranging from 0..360, e.g., 225 degree dihedrals.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ When they report a negative dihedral angle, or an angle larger than 180, there has to be some way they are determining directionality. Otherwise the results are ambiguous. $\endgroup$
    – Steven C. Howell
    Commented Nov 4, 2015 at 20:15
  • $\begingroup$ The link you provided does describe the orientation, "In a Newman projection the torsion angle is the angle (having an absolute value between 0° and 180°) between bonds to two specified (fiducial) groups, one from the atom nearer (proximal) to the observer and the other from the further (distal) atom. The torsion angle between groups A and D is then considered to be positive if the bond A-B is rotated in a clockwise direction through less than 180° in order that it may eclipse the bond C-D: a negative torsion angle requires rotation in the opposite sense." $\endgroup$
    – Steven C. Howell
    Commented Nov 4, 2015 at 20:17
  • $\begingroup$ I am not familiar with the IUPAC Gold Book but it seem valid. According to this definition, the wiki page is incorrect and the torsion angle is defined using the right-hand rule along the line connecting atoms 2 and 3. $\endgroup$
    – Steven C. Howell
    Commented Nov 4, 2015 at 20:27
  • 1
    $\begingroup$ My point is that despite the Gold Book, different software (or people) report different references. $\endgroup$
    – Geoff Hutchison
    Commented Nov 4, 2015 at 21:00
  • $\begingroup$ This is good to note. I think the most important point is that they use the same directionality (but maybe this is not the case). If a positive angle is always defined using the right-hand rule about the B-C line (simplified version of the IUPAC Gold Book), converting from -60 to +300 is straightforward. Without a common orientation, anything but 0-180 is ambiguous. $\endgroup$
    – Steven C. Howell
    Commented Nov 4, 2015 at 21:05

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.