According to me, $\ce{Fe^{2+}}$ should be a better reducing agent because $\ce{Fe^2+}$ - after being oxidized - will attain a stable $\ce{d^5}$ configuration, whereas $\ce{Cr^2+}$ will attain a $\ce{d^3}$ configuration. I think the half filled $\ce{d^5}$ configuration is more stable than the $\ce{d^3}$ configuration. Why is this not so?
$\begingroup$
$\endgroup$
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
0
The short answer is thermodynamics. Reduction with $\ce{Cr^2+}$ must be more exergonic than reduction with $\ce{Fe^2+}$, we'll get to some numbers in a bit, but let's deal with the concept.
It is tricky to compare the "stability" of two possible products that occur from different pathways. What is more important for the spontaneity of the reaction is the change in (free) energy that occurs from start to finish.
To put it another way, it may be that $\ce{Fe^3+}$ is more stable than $\ce{Cr^3+}$ on an absolute scale, but what we really care about is how much more stable $\ce{Cr^3+}$ is to $\ce{Cr^2+}$ compared to how much more stable $\ce{Fe^3+}$ is to $\ce{Fe^2+}$.
Let's examine all four using electron configuration as you have done:
- $\ce{Fe^2+}$ is $\ce{d^6}$ or more probably $\ce{[Ar] 4s^1 3d^5}$ - two half-filled half shells!
- $\ce{Fe^3+}$ is $\ce{d^5}$, which is $\ce{[Ar] 3d^5}$ - one filled half shell.
The difference between the two iron ions might not be that large.
- $\ce{Cr^2+}$ is $\ce{d^4}$, which is $\ce{[Ar] 3d^4}$ or $\ce{[Ar] 4s^2 3d^2}$
- $\ce{Cr^3+}$ is $\ce{d^3}$, which is $\ce{[Ar] 3d^3}$ or $\ce{[Ar] 4s^2 3d^1}$
The energy difference between chromium ions might be larger. Represented graphically, the reaction coordinate energy diagram for the two process might be:
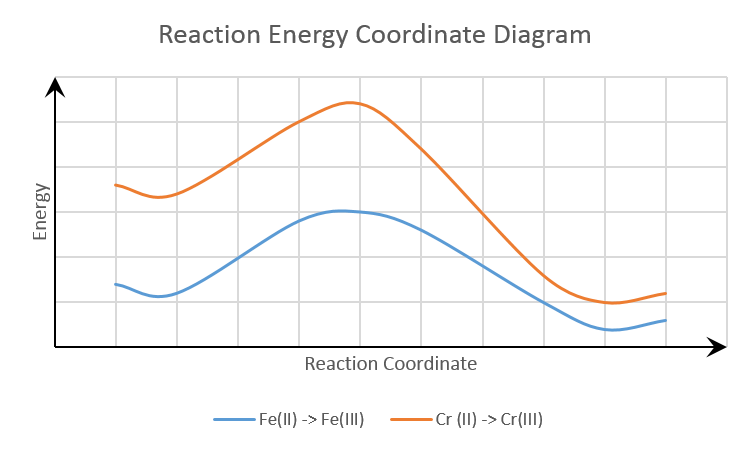
Now the number part.
We can go looking for some standard thermodynamic data to help make our case. The best data are for standard reduction potentials, because these data are for exactly what we want!
Taking the reduction potential data and writing the equations in the direction we care about, we have:
$$\begin{align} &\ce{Fe^2+ -> Fe^3+} &&&E^\circ=\pu{-0.77 V}\\ &\ce{Cr^2+ -> Cr^3+} &&&E^\circ=\pu{+0.44 V} \end{align}$$
Spontaneous reactions produce positive potential differences, so we can see right now that $\ce{Cr^2+}$ is a better reducing agent.
Let's go a step farther to free energy.
$$\Delta G^\circ =-nFE^\circ$$
However, to deal with free energy, we need a full reaction. Technically, comparing the two half-reactions in isolation is just as bad. However, the definition of the standard electrode potential and the standard free energy come with a common zero point in terms of half-reaction: $\ce{2H+ + 2e- -> H2}$.
The two full reactions (net ionic equations anyway) are:
$$\begin{align} &\ce{2Fe^2+ + 2H+ -> 2Fe^3+ + H2} &&E^\circ=\pu{-0.77 V}&&&\Delta G^\circ=\pu{+74kJ/mol}\\ &\ce{2Cr^2+ + 2H+ -> 2Cr^3+ + H2} &&E^\circ=\pu{+0.44 V}&&&\Delta G^\circ = \pu{-42 kJ/mol} \end{align}$$
And the winner is chromium, by a whopping $\pu{116 kJ/mol}$. In fact, the data might show that $\ce{Fe^2+}$ is more stable than $\ce{Fe^3+}$, while $\ce{Cr^2+}$ is less stable than $\ce{Cr^3+}$.
answered Jul 7, 2015 at 18:45
$\begingroup$
$\endgroup$
In water both $\ce{Cr^{3+}}$ and $\ce{Fe^{3+}}$ are in octahedral configurations. This means that $d$-orbitals become unequal in their energy; specifically 3 of them are lower and 2 are higher. This is the premise of 'crystal field theory'.
Depending on the nearest neighborhood, the splitting may be strong enough to force electron pairing or it may not. For water it usually isn't. This means that 'first half-filled shell' here is 3 electrons - $\ce{V^{2+}}$ $\ce{Cr^{3+}}$ or $\ce{Mn^{4+}}$. The second half-filled shell in low field (water ligand) is $\ce{Mn^{2+}}$ or $\ce{Fe^{3+}}$ (5 $d$-orbitals), both surprisingly stable. In strong field (say, $\ce{CN^-}$ ligand), it is 6 electrons (3 double occupied lower orbitals), like in $\ce{[Co(NH3)_{6}]^{3+}}$ and $\ce{[Fe(CN)_{6}]^{4-}}$. The next "subshell" is, 8 electrons, (6 on 3 lower orbitals and 2 on higher), like in $\ce{Ni^{2+}}$. Depending on the strength of the ligands, an unusual square planar coordination may become preferable, with two higher orbitals also splitting. It is typical for $\ce{Ni}$ subgroup in +2 oxidation state and $\ce{Cu}$ subgroup in +3 oxidation state.
TL;DR : invest some time into reading about crystal field theory.
$\begingroup$
$\endgroup$
0
It is quite easy, a good reducing agent means which can be oxidised easily, for:
$\ce{Cr^2+ -> Cr^3+} $, we have $\mathrm d^3$ configuration for $\ce{Cr^3+} $, which we can say that stable as all the three electrons are in $\mathrm{t_{2g}}$ level, hence it is half-filled. On the other hand, for:
$\ce{Fe^2+ -> Fe^3+} $, we have $\mathrm d^5$ configuration for $\ce{Fe^3+} $, which is again stable due to half-filled d orbitals. So, who is more stable? we can figure out from $\ce{M^3+/M^2+}$ standard electrode potentials. for $\ce{Cr^2+ -> Cr^3+} $, it is $\pu{-0.41 V}$ , and for $\ce{Fe^2+ -> Fe^3+} $, it is $\pu{0.77 V}$.
The positive one indicates that it is difficult to remove electron from $\ce{Fe^2+}$ and negative one indicates that it is easier to remove electron from $\ce{Cr^2+}$. Hence, $\ce{Cr^2+}$ is a stronger reducing agent.
$\begingroup$
$\endgroup$
$\ce{Cr^2+}$ is a better reducing agent. As it attains a $\ce d^3$ configuration on loosing an electron while $\ce{Fe^2+}$ attains $\ce d^5$ configuration. In an aqueous medium $\ce d^3$ (half filled $\ce{t_{2g}}$ orbital) is more stable than $\ce d^5$.
