Reason for the formation of azeotropes

Question

Why is it that some liquid mixtures (that exhibit positive/negative deviation from Raoult's law) form azeotropic mixtures at certain compositions? What is the physical reason behind this; are there any extra forces holding the two components together? If so, please elaborate on this.

Answer 1

A solution that has a maximum or minimum vapour pressure (vs mole fraction) is called an azeotrope. The liquid is in equilibrium with the vapour and mole fractions in the liquid are the same as in the vapour at a given temperature. As the composition of liquid and vapour are the same the $dp/dx_A=0$ where $p$ is pressure and $x_A$ mole fraction of species A. (The mole fraction of species B is $x_B=1-x_A$). The cause of this effect is intermolecular interaction between species A and B. This may be hydrogen bonding but need not be since benzene/cyclohexane and chloroform/hexane and many others form an azeotrope. Other intermolecular interactions are often generally called van-der-waals interactions and are dipole-dipole and pi-pi interactions among others.

In a perfect solution the total pressure $p$ of a binary mixture varies linearly with mole fraction of either component, (Raoult's law)i.e. $\displaystyle p=x_Ap^0_A+x_Bp^0_B = x_Ap^0_A+(1-x_A)p^0_B$ where $x$ are the mole fractions and $p^0_A,p^0_B$ the partial pressures of the pure liquids. Experimentally this 'law' is found to occur only when the species A and B are very similar, such as ethylene bromine and propylene bromide, or benzene and dichlorobenzene. Clearly such mixtures will not form azeotropes. What is needed is to modify the partial pressures to account for interaction between molecules.

The result of allowing pairs of molecules to interact with energy $\Delta E$ is

$$p=p^0_Ax_Ae^{(1-x_A)^2\Delta E/k_BT}+p^0_B(1-x_A)e^{x_A^2\Delta E/k_BT}$$

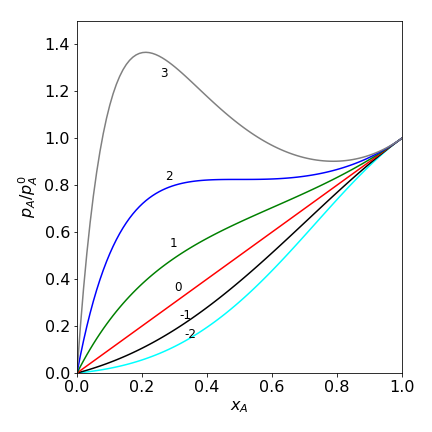
which now depends on the A-B interaction energy and predicts variations from Raoults law for energies comparable or larger than $k_BT$ where $k_B$ is the Boltzmann constant. The detailed calculation is shown below and the figure shows how the vapour pressure deviates from the idea. The numbers show different values of $\Delta E/k_BT $. A value of zero corresponds to Raoult's law. The total pressure expressed in this way has a maximum or minimum depending on the sign and magnitude of the interaction energy.

A figure showing ratio of vapour pressure vs mole fraction for species A. The numbers correspond to $\Delta E/k_BT$. The curve with $\Delta E/k_BT=1$ is close to that for ethanol in water. When the total pressure for species A and B is plotted a maximum or minimum is produced just as in an azeotrope.

---

Derivation of vapour pressure curve equation.

The aim is to calculate the chemical potential using statistical arguments and the interaction energy between molecules. The chemical potential is given by $\mu=\mu^0+RT\ln(a)$ where $a$ is the activity, and the partial pressure $p=p^0a=p^0\gamma x$ where $\gamma$ is the activity coefficient and $x$ the mole fraction. The chemical potential is the partial derivative of the Gibbs energy with number of moles $n$, for species A for example $\displaystyle\mu_A= \left( \frac{\partial G}{\partial n_A}\right)_{T,p,n_B} $. Notice that $T,p$ and $n_B$ are the temperature, pressure, and the number of moles of species B, all of which should be held constant. The Gibbs energy is $\Delta G = \Delta H-T\Delta S = \Delta E+p\Delta V-T\Delta S$ and as we shall make the volume constant $\Delta G = \Delta H-T\Delta S = \Delta E-T\Delta S$ thus the energy and entropy changes have to be determined.

A regular solution is considered to be a random mixture of type A and B molecules in which specific interactions, such as hydrogen bonding, are absent but in which there is nevertheless interaction between molecules of either type and between different types, i.e interactions of the form AA, BB and AB and these need not be equal to one another. The entropy of mixing is assumed to be that of an ideal solution. Some simplifying, but not entirely realistic, assumptions are made so that a tractable solution can be obtained and are (i) that the molecules are situated on a lattice (ii) the molecules are of approximately the same size, which will allow random mixing and no change in volume, and (iii) intermolecular forces are only between nearest neighbours. This is called the Bragg-Williams model.

As we assume $z$ nearest neighbour interactions only, the intermolecular potential energy of solution A with molecules at their equilibrium positions is $U_{AA}=N_Azw_{AA}/2$ for $N_A$ molecules with average potential energy $w_{AA}$. The 1/2 arises because there are $N_Az/2$ pairs of molecules. There is a similar energy for solution B. In the mixture there are three types of pairs AA, BB and AB and the total energy of the mixture is then

$$U_{AB}=N_{AA}w_{AA}+N_{BB}w_{BB}+N_{AB}w_{AB}$$

and the change in energy on mixing is

$$\Delta E_{mix}= U_{AB}-U_{AA}-U_{BB} $$

To continue the calculation $N_{AA}$ and $N_{BB}$ are needed in terms of $N_{AB}$.

Each A molecule has $z$ neighbours and so $zN_A$ in total. This number of neighbours is also $N_{AB}+2N_{AA}$ producing $N_{AA}=(zN_A-N_{AB})/2$ and similarly for B, $N_{BB}=(zN_B-N_{AB})/2$. The factor of 2 arises because AA pairs are counted twice and AB pairs only once. Substituting gives

$$\Delta E_{mix} = N_{AB} \left( w_{AB}-\frac{ w_{AA} }{2}-\frac{ w_{BB} }{2} \right)$$

A statistical argument is now used to find the number $N_{AB}$. As $N = N_A+N_B $ the first random choice to pick any molecule can be made in $N$ ways, the next can be made in $N-1$ ways hence to pick two $N(N-1)/2 \approx N^2/2$ as $N$ is very large. (The 1/2 arises are there are two ways of choosing, i.e. it does not matter which is chosen first.) This gives the number of all pairs. If, however, we must choose molecule A first and then B the number is $N_AN_B$ then the fraction of these AB pairs is $2N_AN_B/N^2$. As each of the $N$ molecules has $z$ neighbours, the total number of neighbouring pairs is $Nz/2$ thus

$$N_{AB}= \frac{zN}{2}\frac{2N_AN_B}{N^2}=\frac{zN_AN_B}{N}$$

The energy is now

$$\Delta E_{mix} = z\frac{N_AN_B}{N_A+N_B} \left( w_{AB}-\frac{ w_{AA} }{2}-\frac{ w_{BB} }{2} \right)$$

Letting $n_A,\,n_B$ be the number of moles of A and B respectively, and $N_0$ Avogadro's number and making $\displaystyle w= w_{AB}-\frac{ w_{AA} }{2}-\frac{ w_{BB} }{2} $ gives

$$\Delta E_{mix} = N_0 z w\frac{n_An_B}{n_A+n_B}$$

As we assume that the mixing is random the entropy change on mixing is that for an ideal mixture, namely

$$\Delta S_{mix} = -R\left(n_A\ln(x_A)+n_B\ln(x_B)\right)$$

where $x$ are the mole fractions. The derivation of the entropy change can be found here Deriving the entropy of mixing of a non-ideal solution.

Combining equations gives

$$\Delta G_{mix} = N_0 z w\frac{n_An_B}{n_A+n_B} -RT\left(n_A\ln(x_A)+n_B\ln(x_B)\right)$$

The free energy is $G=G_A+G_B+\Delta G_{mix} $ and differentiating to give the chemical potential gives

$$\mu_A=\mu_A^0 + \left( \frac{\partial \Delta G_{mix}}{\partial n_A}\right)_{T,p,n_B} $$

where $G_B$ differentiates to zero because $n_B$ is a constant when differentiating with $n_A$. By definition $\partial G/\partial n_A=\mu^0$. Performing the differentiation $\Delta G_{mix}$ with $n_A$ gives

$$\mu_A=\mu_A^0 +RT\left(\ln(x_A)+\frac{N_0zw}{RT}x_B^2\right) $$

simplifying by letting $\beta = N_0zw/RT$ gives $\displaystyle \mu_A=\mu_A^0 +RT\left(\ln(x_A)+\beta x_B^2\right) $. There is a similar equation for $\mu_B$.

Since $\mu_A=\mu^0_A+RT\ln(a_A)$ then $\ln(a_A)=\ln(x_A)+\beta x_B^2$ and as $a_A=\gamma_A x_A$ then $\gamma_A=e^{\beta x}$ and so

$$p_A=p_A^0\gamma_A x_A = p_A^0x_Ae^{\beta x_B^2}$$ and also for $p_B$

$$p_B=p_B^0x_Be^{\beta x_A^2}$$

as $\beta = N_0zw/RT=zw/k_BT$ then as $zw$ is an energy this partial pressure becomes equal to $p^0_Ax_Ae^{(1-x_A)^2\Delta E/k_BT}$ which is the equation for the partial pressure we sought.

The azeotrope can be determined by calculating the derivative of the total pressure and setting it to zero, $\left( dp/dx_A \right)_T =0$ giving $\displaystyle x_A^{azeo} =\frac{1}{2} \left( 1+ \frac{k_BT}{\Delta E}\ln\left( \frac{p_A^0}{p_B^0} \right) \right)$

Answer 2

Before getting to the azeotropes, it's worth reminding ourselves of the relevant assumption that we make about ideal mixtures: that the intermolecular interactions have constant energy regardless of the mixture.

Given the important role that intermolecular interactions have in determining the boiling point of a pure liquid, it's surprising that so many mixtures behave approximately ideally, in line with this assumption. There are two main reasons for this.

First, liquids are only miscible if they have somewhat similar types of intermolecular interactions with similar magnitudes of energy. For example, water and hexane have very different intermolecular interactions, so surrounding water with hexane or hexane with water is quite unfavorable, and the two do not mix. Thus, any actual mixture has interactions between the two types of molecules that are not hugely different from the interactions within the pure liquids.

Second, substances that are liquid are room temperature generally do not have especially strong intermolecular forces, so the small differences in the intermolecular interactions of two miscible liquids is usually dwarfed by the impact of the difference in molecular weight that is a major determinant of boiling point. Water is quite unusual in having such a low molecular weight and still being a liquid at room temperature, a symptom of the exceptionally strong role of intermolecular interactions in determining its boiling point.

With that in mind, we can talk about azeotropes. To get a minimum boiling azeotrope, the deviation from Raoult's Law requires that the vapor pressure of the lower boiling component be reduced from what is predicted for ideal behavior and that the vapor pressure of the higher boiling component be increased. In order for that to happen, we need two liquids with substantially different intermolecular interactions whose mixing changes those interactions in opposite directions.

In the example of the water:ethanol azeotrope, the water is able to increase the amount of hydrogen bonding between ethanol molecules by increasing the density of hydrogen bond donors and acceptors per unit volume. At a low water concentration, the water also does not interfere greatly with the hydrophobic interactions between the ethyl ends of the molecules. As a result, the average intermolecular interaction for the ethanol molecules is stronger than in pure ethanol, and we have the necessary decrease in the vapor pressure of ethanol. For the water, when it is at low concentration in mixture, each water molecule is surrounded by ethanol molecules, so it has fewer hydrogen bonding interactions than in pure water. As a result, its average intermolecular interactions are weaker than in pure water, and we have the necessary increase in vapor pressure. Many low molecular weight alcohols form azeotropes with water for the same reasons.

When the water concentration gets below what is required for the azeotrope, enough of the alcohol molecules interact only with other alcohol molecules that the deviation from ideality decreases.

For the maximum-boiling azeotropes, the requirement is that the vapor pressure of both components is decreased. This is quite common in acid:water mixtures where the degree of ionization of the acid in the mixture is higher than when it is in pure form, which means that there are more ionic interactions, which are quite strong. If the water concentration gets below what is required for the azeotrope, the amount of ionization decreases and the deviation from ideality decreases with it. When the water concentration is higher than in the azeotrope, there may be a great deal of ionization of the acid, but there is enough water that is not interacting with the acid (and conjugate base) molecules that the deviation from ideality is less.

Answer 3

 Is there any extra force holding the two components together?

   Correct. Namely, hydrogen bonding. As a quick refresher, hydrogen bonding is an electrostatic attraction between an electropositive hydrogen atom on one compound being (weakly) attracted to a highly electronegative atom on another, nearby molecule, typically oxygen, although it can happen between a hydrogen and another electronegative atom such as oxygen or fluorine.

   Azeotropes form when there is a 'deviation from Raoult's law':

   A solution that shows positive deviation from Raoult's law forms a minimum boiling azeotrope. A very well-known example would be the azetrope that forms from a ethanol and water mixture when it is approximately 95% ethanol by volume. Once this composition has been achieved, the liquid and vapor have the same composition, and no further separation occurs. Methods for breaking this azetrope are typically either via chemical means, such as adding a small amount of benzene to the azetrope, or by a drying agent such as $\ce{H2SO4}$, magnesium sulfate, or calcium chloride. Interesting thing to note: You do not have to dry all the way to 100% ethanol, but simply BREAK the azetrope by getting it beyond that 95.6% concentration. After you get it beyond ~96%, you can distill the rest of the way to 99% via the normal distillation method.

   A solution that shows a negative deviation from Raoult's law forms a MAXIMUM boiling azeotrope, or as it is often stated: A negative deviation from Raoult's law is where the adhesive forces between different components are stronger than the average cohesive forces between like components. In consequence each component is retained in the liquid phase by attractive forces that are stronger than in the pure liquid so that its partial vapor pressure is lower. Nitric acid and water is a classic example of this class of azeotrope which forms when the solution has an approximate composition of 68% nitric acid and 32% water by mass.

Answer 4

I like the wikipedia answer https://en.wikipedia.org/wiki/Azeotrope

in other words: Raoult's law predicts the vapor pressures of ideal mixtures as a function of composition ratio. More simply: per Raoult's law molecules of the constituents stick to each other to the same degree as they do to themselves. For example, if the constituents are X and Y, then X sticks to Y with roughly equal energy as X does with X and Y does with Y. A so-called positive deviation from Raoult's law results when the constituents have a disaffinity for each other – that is X sticks to X and Y to Y better than X sticks to Y. Because this results in the mixture having less total affinity of the molecules than the pure constituents, they more readily escape from the stuck-together phase, which is to say the liquid phase, and into the vapor phase. When X sticks to Y more aggressively than X does to X and Y does to Y, the result is a negative deviation from Raoult's law. In this case because the molecules in the mixture are sticking together more than in the pure constituents, they are more reluctant to escape the stuck-together liquid phase.

When the deviation is great enough to cause a maximum or minimum in the vapor pressure versus composition function, it is a mathematical consequence that at that point, the vapor will have the same composition as the liquid, resulting in an azeotrope.

Why do things stick to each other?

I like to think of atoms as having dispersion and electrostatic attractions/repulsion. This is a simplification, but that is not a bad thing for a stack exchange answer. The core of an atom interacts with the core of other atoms, a simple model of this is the Lennard-Jones model. Far away they attract, really close they push away from each other. Around the core are electrons. They interact with other atoms and their electrons too.

Hydrogen is interesting because when it is part of a molecule, usually the rest of the molecule hogs the electrons, so the hydrogen becomes positively charged. This happens in other molecules too, so they have areas that are more positive and also other areas on them that are more negative. Thus these now positive hydrogens become attracted to the overly negative areas of other molecules and they "stick" to them but only for a short while because they are after all covalently bonded to their own molecule, and this is much stronger than any short "flings" hydrogen may have with other molecules.