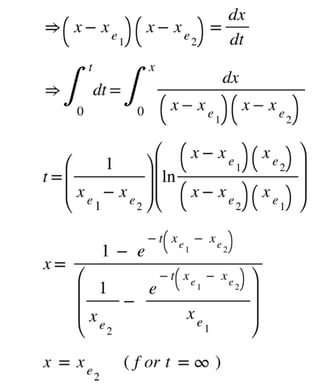0
$\begingroup$

Say there is a reversible reaction, A + B ⇌ AB. Let K1 be rate of forward reaction and K2 be the rate of backward reaction.

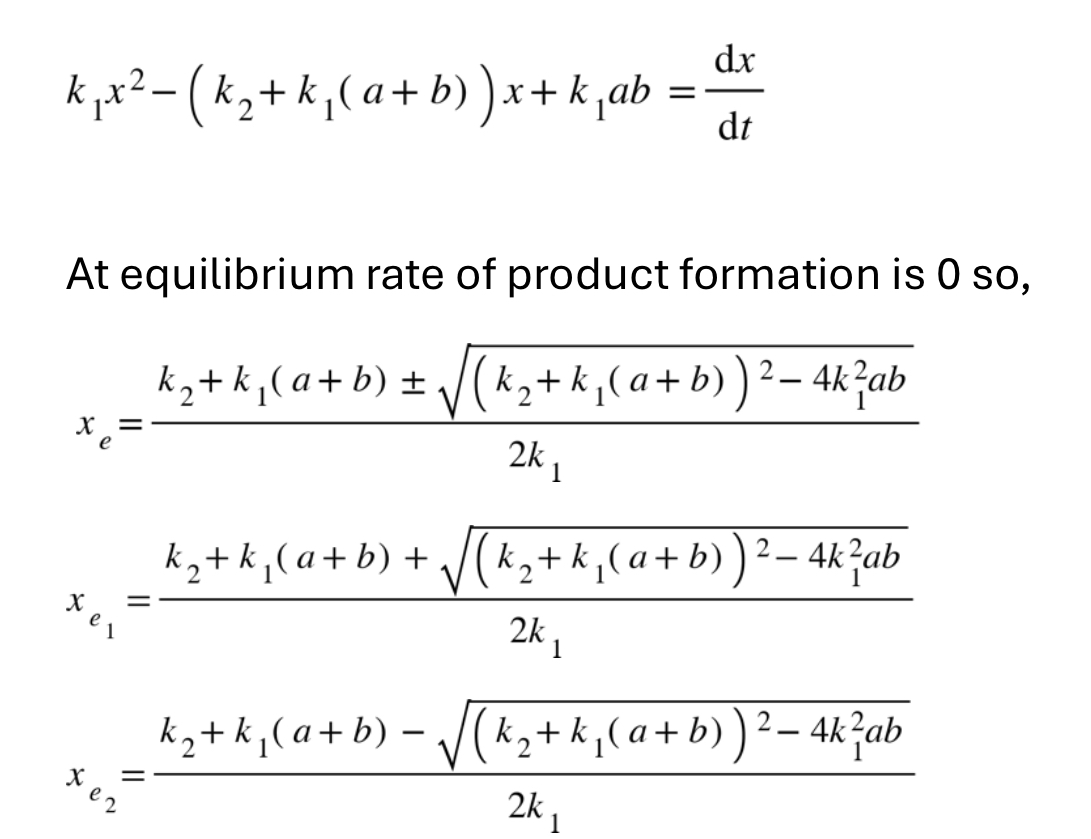
When I try to solve such an equation for AB (the concentration of AB produced at any point of time is taken as x, given that initially no AB was present) taking initial concentrations for A as a and B as b, I get two values for AB at equilibrium.

Result 1 :enter image description here

Also when I try to solve further for x I get the following results.

Result 2 :

enter image description here

Now, when I try to put x for $x_{e_1}$ I get the result $x_{e_1}$= $x_{e_2}$. To me this seems inconsistent with the result I had observed before if there can be more than one values at equilibrium which is also unlikely (Result 1).

So can anyone please interpret/correct the above results for the obtained values of x. Obviously this tells me that values under square root in the obtained roots are zero but still since I couldn’t find the solution anywhere I would like to confirm the answer.

Improve this question
$\endgroup$
8
  • $\begingroup$ You know that the $x_e$ values have to be real and positive. Then you can see that the result inside the square root has to be positive. As this has to be the case only the root $x_{e2}$ could then be negative which is not physically possible. This sort of thing is common between maths giving answers and reality. You could integrate the differential equation, it is a standard integration (but messy) to find the values at long times. $\endgroup$
    – porphyrin
    Commented Oct 13 at 10:08
  • 2
    $\begingroup$ Dimensionally what you have looks wrong. Have you missed a k1 in front of a and b in the bracket before and in the discriminant? Showing more of your working would be helpful. $\endgroup$
    – Ian Bush
    Commented Oct 13 at 11:01
  • $\begingroup$ @porphyrin I corrected the equation and found an answer but I would still like to confirm it. $\endgroup$
    – Lakshya Kumar Singh
    Commented Oct 13 at 11:32
  • 1
    $\begingroup$ Guides for formatting of chemical/mathematical formulas/expressions/equations: Basics / Detailed / Upright vs Italics / Math SE Mathjax tutorial // MathJax is preferred not to be used in CH SE Q titles. $\endgroup$
    – Poutnik
    Commented Oct 13 at 11:43
  • $\begingroup$ your correction has not addressed the issue pointed out by @IanBush. Specifically, you cannot sum $a+b+k_2$ as $k_2$ is not a concentration. Start fresh and redo the factoring/rearranging. That sum should have the form $k_1a + k_1b +k_2$ $\endgroup$
    – Andrew
    Commented Oct 13 at 12:55

1 Answer 1

Reset to default
3
$\begingroup$

Dimensionally this last edit is not correct, you have concentration on the left and concentration/time on the right. If the reaction is A+B=C then $d[A]/dt=-k_1[A][B]+k_2[C]$ and if $A_0,B_0,C_0$ are the initial amounts and amount $x$ reacts at time $t$ then

$$\displaystyle \frac{dx}{dt}= k_1(A_0-x)(B_0-x)-k_2(C_0+x)$$

and then you can integrate this in the form $\displaystyle \int_0^x \frac{dx}{a+bx+cx^2}=\int_0^t dt$ and its simpler to work out work out the constants $a,b,c$ after doing the integration.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.